Trig Ratio Worksheet with Answers and Examples
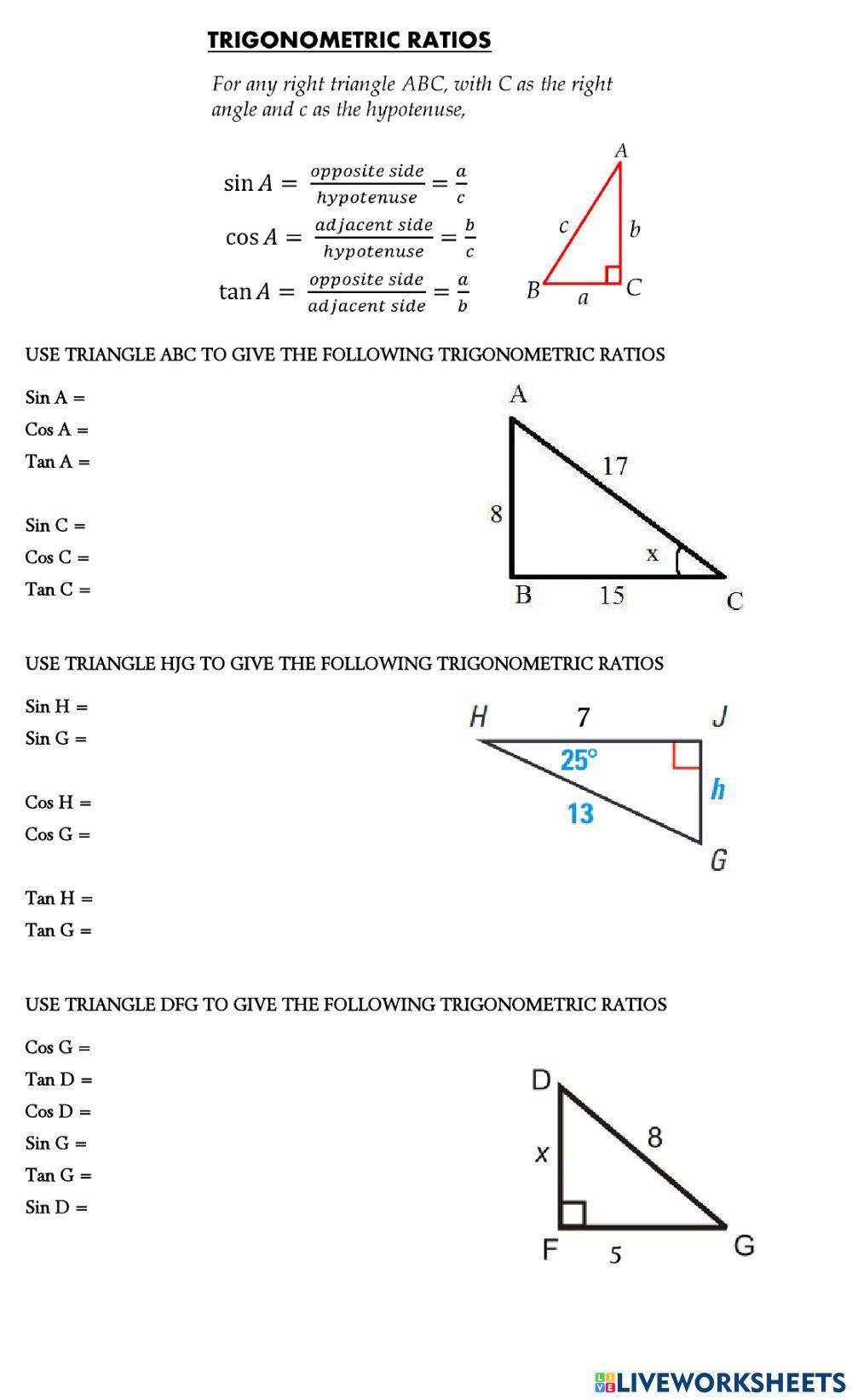
Understanding Trigonometric Ratios: A Comprehensive Guide
Trigonometry is a branch of mathematics that deals with the relationships between the sides and angles of triangles. Trigonometric ratios, also known as trig ratios, are the building blocks of trigonometry. In this article, we will delve into the world of trig ratios, exploring their definitions, formulas, and examples. We will also provide a worksheet with answers to help you practice and reinforce your understanding.
What are Trigonometric Ratios?
Trigonometric ratios are ratios of the lengths of the sides of a right triangle. The three basic trig ratios are:
- Sine (sin): The ratio of the length of the side opposite a given angle to the length of the hypotenuse (the side opposite the right angle).
- Cosine (cos): The ratio of the length of the side adjacent to a given angle to the length of the hypotenuse.
- Tangent (tan): The ratio of the length of the side opposite a given angle to the length of the side adjacent to that angle.
These ratios can be expressed mathematically as:
sin(A) = opposite side / hypotenuse cos(A) = adjacent side / hypotenuse tan(A) = opposite side / adjacent side
Trigonometric Ratio Formulas
Here are some important trig ratio formulas to remember:
- Pythagorean Identity: sin^2(A) + cos^2(A) = 1
- Reciprocal Identities: sin(A) = 1 / csc(A), cos(A) = 1 / sec(A), tan(A) = 1 / cot(A)
- Quotient Identities: tan(A) = sin(A) / cos(A), cot(A) = cos(A) / sin(A)
Examples and Practice Problems
Now that we’ve covered the basics, let’s practice with some examples.
Example 1: Find the sine, cosine, and tangent of angle A in the right triangle below.
| Angle A | 30° |
| Opposite side | 3 |
| Adjacent side | 6 |
| Hypotenuse | 6.928 |
Using the trig ratio formulas, we can calculate:
sin(A) = 3 / 6.928 = 0.433 cos(A) = 6 / 6.928 = 0.866 tan(A) = 3 / 6 = 0.5
Example 2: Find the value of angle A in the right triangle below.
| Opposite side | 5 |
| Adjacent side | 12 |
| Hypotenuse | 13 |
Using the trig ratio formulas, we can calculate:
tan(A) = 5 / 12 = 0.417 A = arctan(0.417) = 22.62°
Trig Ratio Worksheet with Answers
Now it’s your turn to practice! Here are 10 trig ratio problems with answers.
| Problem | Answer |
|---|---|
| 1. Find sin(A) in a right triangle with angle A = 45°, opposite side = 3, and hypotenuse = 3√2. | 0.707 |
| 2. Find cos(A) in a right triangle with angle A = 60°, adjacent side = 6, and hypotenuse = 12. | 0.866 |
| 3. Find tan(A) in a right triangle with angle A = 30°, opposite side = 2, and adjacent side = 4. | 0.5 |
| 4. Find the value of angle A in a right triangle with opposite side = 8, adjacent side = 15, and hypotenuse = 17. | 27.46° |
| 5. Find sin(A) in a right triangle with angle A = 75°, opposite side = 5, and hypotenuse = 10. | 0.966 |
| 6. Find cos(A) in a right triangle with angle A = 40°, adjacent side = 9, and hypotenuse = 13. | 0.766 |
| 7. Find tan(A) in a right triangle with angle A = 50°, opposite side = 6, and adjacent side = 8. | 0.75 |
| 8. Find the value of angle A in a right triangle with opposite side = 12, adjacent side = 16, and hypotenuse = 20. | 36.87° |
| 9. Find sin(A) in a right triangle with angle A = 25°, opposite side = 4, and hypotenuse = 8. | 0.5 |
| 10. Find cos(A) in a right triangle with angle A = 65°, adjacent side = 10, and hypotenuse = 20. | 0.423 |
💡 Note: Make sure to check your answers using a calculator or trig table.
What is the difference between sine, cosine, and tangent?
+Sine, cosine, and tangent are three basic trigonometric ratios that describe the relationships between the sides and angles of a right triangle. Sine is the ratio of the length of the side opposite a given angle to the length of the hypotenuse, cosine is the ratio of the length of the side adjacent to a given angle to the length of the hypotenuse, and tangent is the ratio of the length of the side opposite a given angle to the length of the side adjacent to that angle.
How do I calculate trigonometric ratios?
+To calculate trigonometric ratios, you can use the formulas: sin(A) = opposite side / hypotenuse, cos(A) = adjacent side / hypotenuse, and tan(A) = opposite side / adjacent side. You can also use trig tables or calculators to find the values of trigonometric ratios.
What are some common applications of trigonometric ratios?
+Trigonometric ratios have many applications in physics, engineering, navigation, and computer science. They are used to describe the relationships between the sides and angles of triangles, and to solve problems involving right triangles. Some common applications include calculating distances, heights, and angles in various fields.
In conclusion, trigonometric ratios are essential in understanding the relationships between the sides and angles of triangles. By mastering the formulas and applications of sine, cosine, and tangent, you can solve a wide range of problems in various fields. Remember to practice regularly and use trig tables or calculators to reinforce your understanding.
Related Terms:
- Hipotenusa
- Trigonometri
- Segitiga
- Geometri
- Logaritma
- Fungsi trigonometri