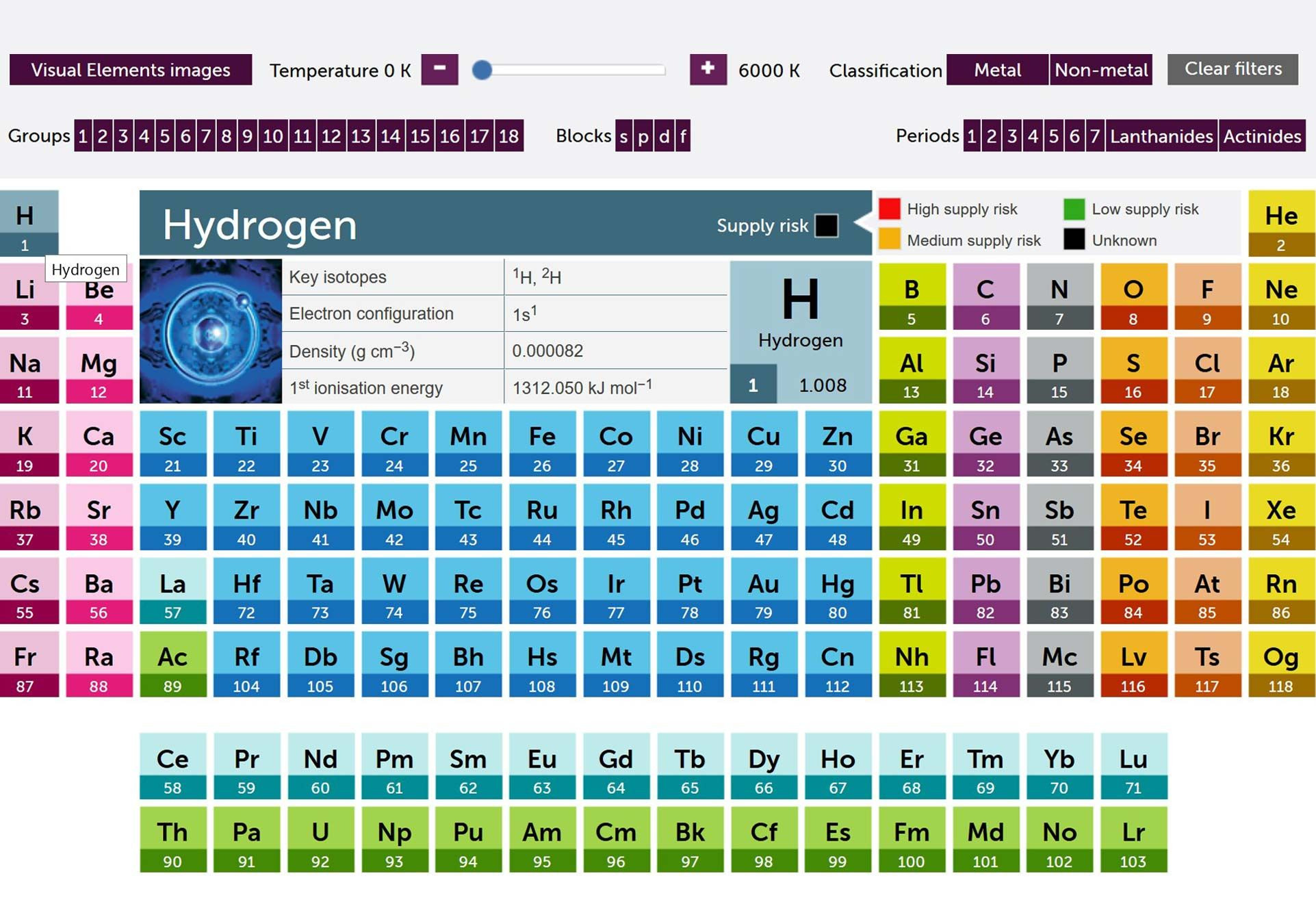
6 Ways to Transform Functions with Ease
Transforming functions is a crucial aspect of mathematics, particularly in calculus, algebra, and other branches of mathematics. It allows us to modify existing functions to fit different scenarios, making it easier to solve problems and analyze data. In this blog post, we will explore six ways to transform functions with ease.
Understanding Function Transformations
Before we dive into the six ways to transform functions, it’s essential to understand what function transformations are. A function transformation is a change made to the graph of a function, which can include shifting, stretching, compressing, or reflecting the graph.
1. Shifting Functions
Shifting a function involves moving the graph of the function horizontally or vertically. There are two types of shifts: horizontal shifts and vertical shifts.
- Horizontal Shifts: A horizontal shift involves moving the graph of a function left or right by a certain number of units. For example, if we have the function f(x) = x^2, and we want to shift it 2 units to the right, the new function would be f(x - 2) = (x - 2)^2.
- Vertical Shifts: A vertical shift involves moving the graph of a function up or down by a certain number of units. For example, if we have the function f(x) = x^2, and we want to shift it 3 units up, the new function would be f(x) + 3 = x^2 + 3.
2. Stretching and Compressing Functions
Stretching and compressing functions involve changing the width or height of the graph of a function.
- Stretching: A stretch involves multiplying the function by a constant factor, which stretches the graph vertically. For example, if we have the function f(x) = x^2, and we want to stretch it by a factor of 2, the new function would be f(x) = 2x^2.
- Compressing: A compression involves dividing the function by a constant factor, which compresses the graph vertically. For example, if we have the function f(x) = x^2, and we want to compress it by a factor of 2, the new function would be f(x) = (1⁄2)x^2.
3. Reflecting Functions
Reflecting a function involves flipping the graph of the function over the x-axis or y-axis.
- Reflection over the x-axis: A reflection over the x-axis involves changing the sign of the function. For example, if we have the function f(x) = x^2, and we want to reflect it over the x-axis, the new function would be f(x) = -x^2.
- Reflection over the y-axis: A reflection over the y-axis involves changing the sign of the input variable. For example, if we have the function f(x) = x^2, and we want to reflect it over the y-axis, the new function would be f(-x) = (-x)^2.
4. Rotating Functions
Rotating a function involves rotating the graph of the function by a certain angle.
- 90-degree rotation: A 90-degree rotation involves swapping the x and y variables. For example, if we have the function f(x) = x^2, and we want to rotate it 90 degrees, the new function would be f(y) = y^2.
5. Scaling Functions
Scaling a function involves changing the size of the graph of the function.
- Scaling vertically: A vertical scale involves multiplying the function by a constant factor. For example, if we have the function f(x) = x^2, and we want to scale it vertically by a factor of 2, the new function would be f(x) = 2x^2.
- Scaling horizontally: A horizontal scale involves dividing the input variable by a constant factor. For example, if we have the function f(x) = x^2, and we want to scale it horizontally by a factor of 2, the new function would be f(x) = (x/2)^2.
6. Combining Transformations
Combining transformations involves applying multiple transformations to a function.
- Example: If we have the function f(x) = x^2, and we want to shift it 2 units to the right, stretch it by a factor of 2, and reflect it over the x-axis, the new function would be f(x - 2) = -2(x - 2)^2.
In conclusion, transforming functions is a powerful tool in mathematics that allows us to modify existing functions to fit different scenarios. By understanding the six ways to transform functions, you can solve problems and analyze data with ease.
What is a function transformation?
+A function transformation is a change made to the graph of a function, which can include shifting, stretching, compressing, or reflecting the graph.
What are the six ways to transform functions?
+The six ways to transform functions are: shifting, stretching and compressing, reflecting, rotating, scaling, and combining transformations.
What is the difference between a horizontal shift and a vertical shift?
+A horizontal shift involves moving the graph of a function left or right by a certain number of units, while a vertical shift involves moving the graph up or down by a certain number of units.