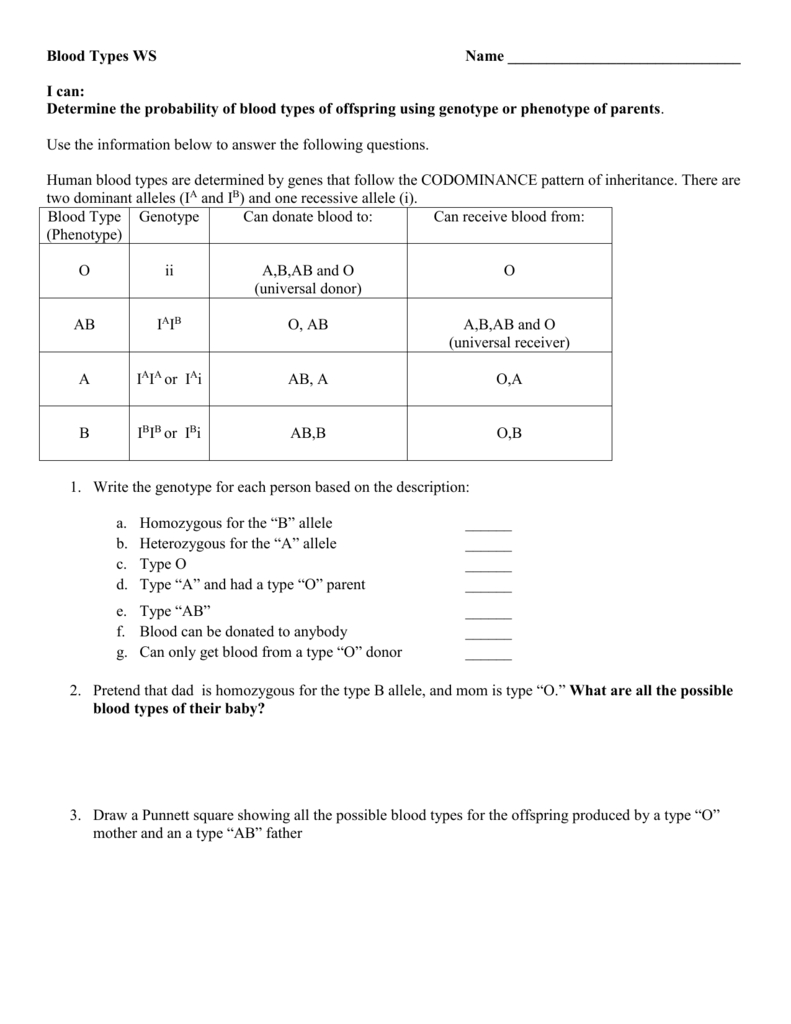
5 Ways to Transform Quadratic Functions

Unlocking the Power of Quadratic Functions

Quadratic functions are a fundamental concept in mathematics, and they have numerous applications in various fields, including physics, engineering, and economics. These functions are polynomial functions of degree two, which means the highest power of the variable is two. Quadratic functions can be represented in the form of f(x) = ax^2 + bx + c, where a, b, and c are constants. In this article, we will explore five ways to transform quadratic functions, which will help you to better understand and manipulate these functions.

Method 1: Horizontal Shifts

A horizontal shift is a transformation that moves the graph of a function horizontally. In the case of quadratic functions, a horizontal shift can be achieved by adding a constant to the variable x. For example, consider the quadratic function f(x) = x^2 + 2x + 1. To shift the graph of this function two units to the right, we can replace x with (x - 2). The resulting function is f(x) = (x - 2)^2 + 2(x - 2) + 1.

👉 Note: When applying a horizontal shift, the parabola opens in the same direction, but its vertex moves horizontally.

Method 2: Vertical Shifts

A vertical shift is a transformation that moves the graph of a function vertically. In the case of quadratic functions, a vertical shift can be achieved by adding a constant to the function. For example, consider the quadratic function f(x) = x^2 + 2x + 1. To shift the graph of this function three units upwards, we can add 3 to the function. The resulting function is f(x) = x^2 + 2x + 1 + 3.

👉 Note: When applying a vertical shift, the parabola opens in the same direction, but its vertex moves vertically.

Method 3: Reflections

A reflection is a transformation that flips the graph of a function over a line. In the case of quadratic functions, a reflection can be achieved by multiplying the function by -1. For example, consider the quadratic function f(x) = x^2 + 2x + 1. To reflect the graph of this function over the x-axis, we can multiply the function by -1. The resulting function is f(x) = -(x^2 + 2x + 1).

👉 Note: When applying a reflection, the parabola opens in the opposite direction, and its vertex is reflected over the line.

Method 4: Stretching and Compressing

Stretching and compressing are transformations that change the width of the graph of a function. In the case of quadratic functions, stretching and compressing can be achieved by multiplying the function by a constant. For example, consider the quadratic function f(x) = x^2 + 2x + 1. To stretch the graph of this function vertically by a factor of 2, we can multiply the function by 2. The resulting function is f(x) = 2(x^2 + 2x + 1).

👉 Note: When applying stretching and compressing, the parabola opens in the same direction, but its width changes.

Method 5: Rotating

Rotating is a transformation that rotates the graph of a function around a point. In the case of quadratic functions, rotating can be achieved by using a rotation matrix. For example, consider the quadratic function f(x) = x^2 + 2x + 1. To rotate the graph of this function by 45 degrees around the origin, we can use a rotation matrix.

The resulting function is f(x) = (x’ - 1)^2 + 2(x’ - 1) + 1.

👉 Note: When applying a rotation, the parabola opens in a different direction, and its vertex is rotated around the point.

In conclusion, transforming quadratic functions is a powerful tool for understanding and manipulating these functions. By applying horizontal shifts, vertical shifts, reflections, stretching and compressing, and rotating, you can change the shape and position of the graph of a quadratic function. This knowledge can be applied to solve problems in various fields, including physics, engineering, and economics.