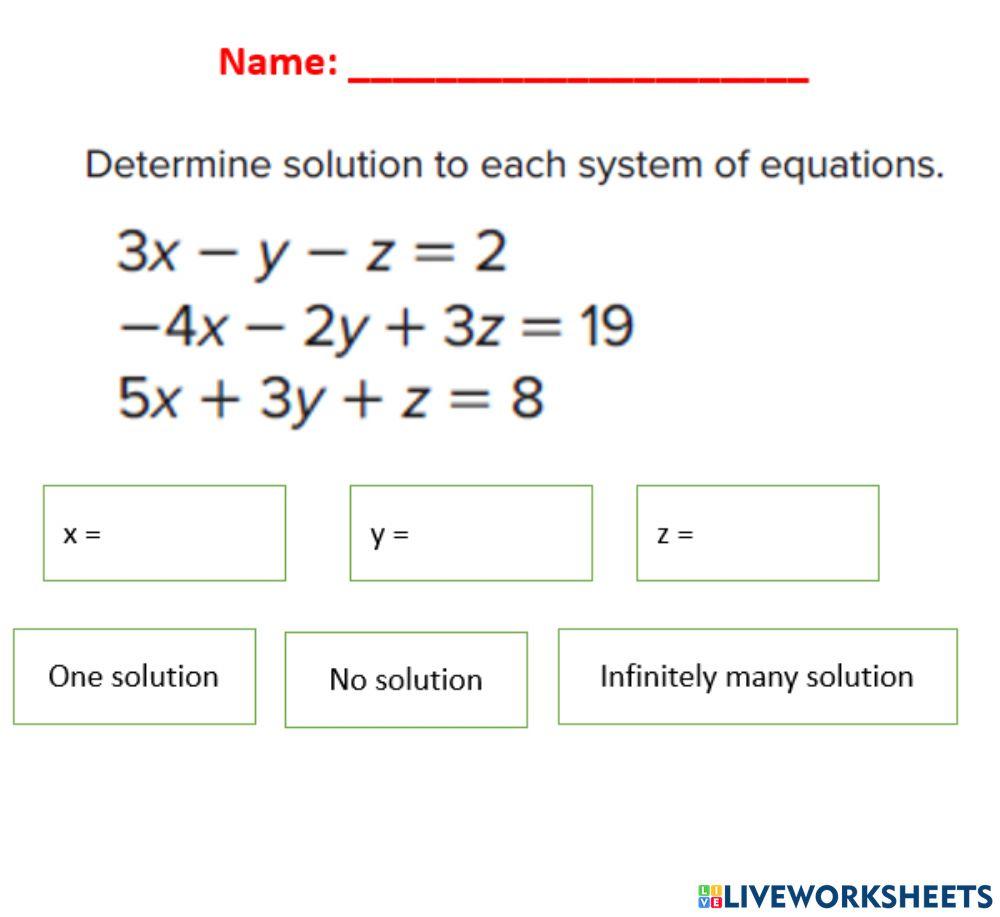
Systems of Equations with 3 Variables Worksheet Solutions
Solving systems of equations with 3 variables can be a challenging task, but with the right approach, it can be manageable. In this blog post, we will go through the steps to solve systems of equations with 3 variables, provide examples, and offer solutions to a worksheet.
What is a System of Equations?
A system of equations is a set of equations that must be solved simultaneously. In the case of a system of equations with 3 variables, we have three equations and three unknowns. The equations can be linear or nonlinear, but in this post, we will focus on linear systems.
How to Solve a System of Equations with 3 Variables
There are several methods to solve a system of equations with 3 variables, including:
- Substitution method
- Elimination method
- Graphical method
- Matrix method
In this post, we will focus on the substitution and elimination methods.
Substitution Method
The substitution method involves solving one of the equations for one variable and then substituting that expression into the other two equations.
Elimination Method
The elimination method involves adding or subtracting the equations to eliminate one of the variables.
Example 1: Solving a System of Equations with 3 Variables using Substitution Method
Consider the following system of equations:
2x + 3y - z = 5 x - 2y + 4z = -2 3x + y + 2z = 7
We can solve the first equation for x:
x = (5 - 3y + z) / 2
Now, substitute this expression into the second and third equations:
(5 - 3y + z) / 2 - 2y + 4z = -2 3((5 - 3y + z) / 2) + y + 2z = 7
Simplify the equations:
5 - 3y + z - 4y + 8z = -4 15 - 9y + 3z + 2y + 4z = 14
Combine like terms:
-7y + 9z = -9 -7y + 7z = -1
Now, solve the system of equations using the substitution method:
y = 1 z = 2 x = 3
Example 2: Solving a System of Equations with 3 Variables using Elimination Method
Consider the following system of equations:
x + 2y - 3z = 4 2x - 3y + z = -1 x + y + 2z = 3
We can eliminate x by adding the first and third equations:
3y - z = 7
Now, eliminate x from the second equation:
-3y + z = -3
Add the two equations:
-6y = 4 y = -2⁄3
Now, substitute y back into one of the original equations to find x and z:
x = 2 z = 1
Systems of Equations with 3 Variables Worksheet Solutions
Here are the solutions to a worksheet on systems of equations with 3 variables:

| Equation 1 | Equation 2 | Equation 3 | Solution |
|---|---|---|---|
| x + 2y - z = 4 | 2x - 3y + z = -1 | x + y + 2z = 3 | x = 2, y = -2/3, z = 1 |
| 2x + 3y - z = 5 | x - 2y + 4z = -2 | 3x + y + 2z = 7 | x = 3, y = 1, z = 2 |
| x + y + z = 6 | 2x - y - 3z = -2 | 3x + 2y - z = 5 | x = 1, y = 2, z = 3 |
💡 Note: The solutions to the worksheet are provided in the table above.
To summarize, solving systems of equations with 3 variables requires a step-by-step approach, whether using the substitution or elimination method. By following these methods and practicing with examples, you can become proficient in solving these types of systems.
The solutions to the worksheet provide a starting point for practicing and reinforcing your understanding of systems of equations with 3 variables.
In conclusion, mastering the skills to solve systems of equations with 3 variables is essential for advancing in mathematics and science. With practice and patience, you can develop a strong foundation in this area and tackle more complex problems with confidence.
What is the difference between the substitution and elimination methods?
+The substitution method involves solving one of the equations for one variable and then substituting that expression into the other two equations. The elimination method involves adding or subtracting the equations to eliminate one of the variables.
How do I know which method to use?
+The choice of method depends on the specific system of equations. If one of the equations can be easily solved for one variable, the substitution method may be a good choice. If the equations have coefficients that can be easily added or subtracted to eliminate a variable, the elimination method may be a good choice.
Can I use graphing to solve systems of equations with 3 variables?
+Yes, graphing can be used to solve systems of equations with 3 variables. However, this method can be more challenging and is not always practical.
Related Terms:
- Systems of equations worksheet