7 Ways to Calculate Cylinder Surface Area

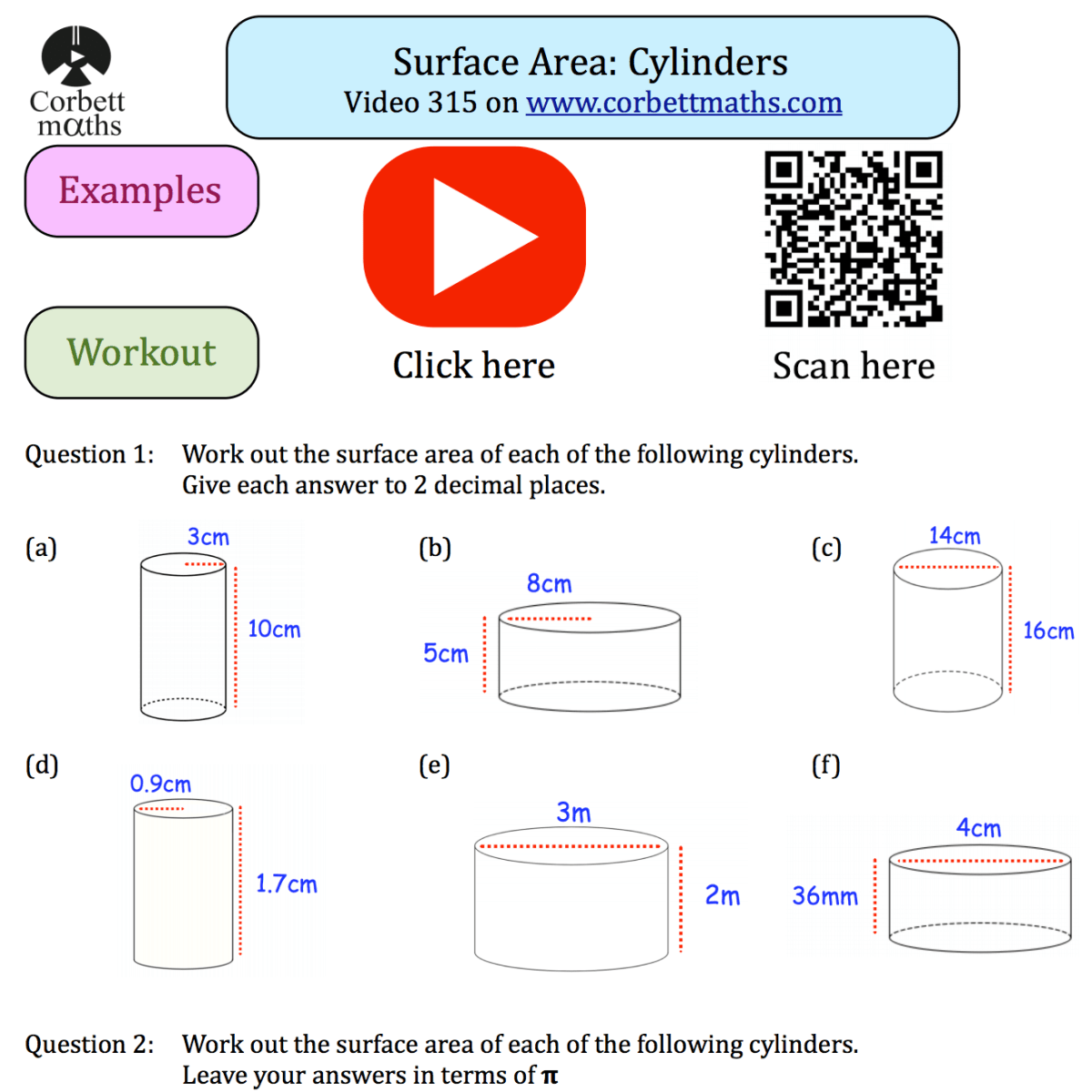
Understanding Cylinder Surface Area

Calculating the surface area of a cylinder can be a bit complex, but with the right formulas and understanding, it can be done easily. A cylinder is a three-dimensional shape with two parallel and circular bases connected by a curved lateral surface. To calculate the surface area of a cylinder, we need to consider the areas of the two bases and the lateral surface.

Formulas for Cylinder Surface Area

There are several ways to calculate the surface area of a cylinder, and each method has its own formula. Here are 7 ways to calculate the surface area of a cylinder:

1. Using the Formula: 2πrh + 2πr^2

This is the most common formula for calculating the surface area of a cylinder. Where:

• r is the radius of the base
• h is the height of the cylinder
• π is a constant approximately equal to 3.14159

Formula: 2πrh + 2πr^2

2. Using the Formula: 2πr(r + h)

This formula is similar to the previous one, but it combines the terms differently.

Formula: 2πr(r + h)

3. Using the Formula: πr(l + 2r)

This formula is used when the lateral surface area is given, and we need to find the total surface area.

Formula: πr(l + 2r)

4. Using the Formula: 2πr^2 + 2πrh

This formula is similar to the first one, but the terms are rearranged.

Formula: 2πr^2 + 2πrh

5. Using the Formula: 2πr(r + √(h^2 + 4r^2))

This formula is used when the slant height of the cylinder is given, and we need to find the total surface area.

Formula: 2πr(r + √(h^2 + 4r^2))

6. Using the Formula: 2πrh + 2πr√(r^2 + h^2)

This formula is similar to the previous one, but the terms are rearranged.

Formula: 2πrh + 2πr√(r^2 + h^2)

7. Using the Formula: πr(l + √(4r^2 + h^2))

This formula is used when the lateral surface area and the height of the cylinder are given, and we need to find the total surface area.

Formula: πr(l + √(4r^2 + h^2))

📝 Note: These formulas are used for different scenarios, so make sure to choose the correct one based on the given information.

Example Calculations

Let’s consider an example to illustrate how to use these formulas:

Suppose we have a cylinder with a radius of 4 cm and a height of 10 cm. We need to find the total surface area of the cylinder.

Using the first formula: 2πrh + 2πr^2

Surface Area = 2π(4)(10) + 2π(4)^2 Surface Area ≈ 301.59 cm^2

Using the second formula: 2πr(r + h)

Surface Area = 2π(4)(4 + 10) Surface Area ≈ 301.59 cm^2

Both formulas give us the same result.

Conclusion

Calculating the surface area of a cylinder can be done using various formulas, each with its own scenario. Understanding the correct formula to use based on the given information is crucial for accurate calculations. By mastering these formulas, you’ll be able to solve problems related to cylinder surface area with ease.

Related Terms:

• Cylinder surface area volume Worksheet
• Surface area of sphere worksheet