5 Ways to Master Square Roots with Worksheets

Unlocking the Secrets of Square Roots

Are you tired of struggling with square roots? Do you wish you could confidently solve equations and problems that involve these mathematical concepts? Look no further! Mastering square roots is a crucial skill that can open doors to new levels of mathematical understanding and problem-solving abilities. In this article, we’ll explore five effective ways to master square roots, along with accompanying worksheets to help you practice and reinforce your learning.

Method 1: Understanding the Concept of Square Roots

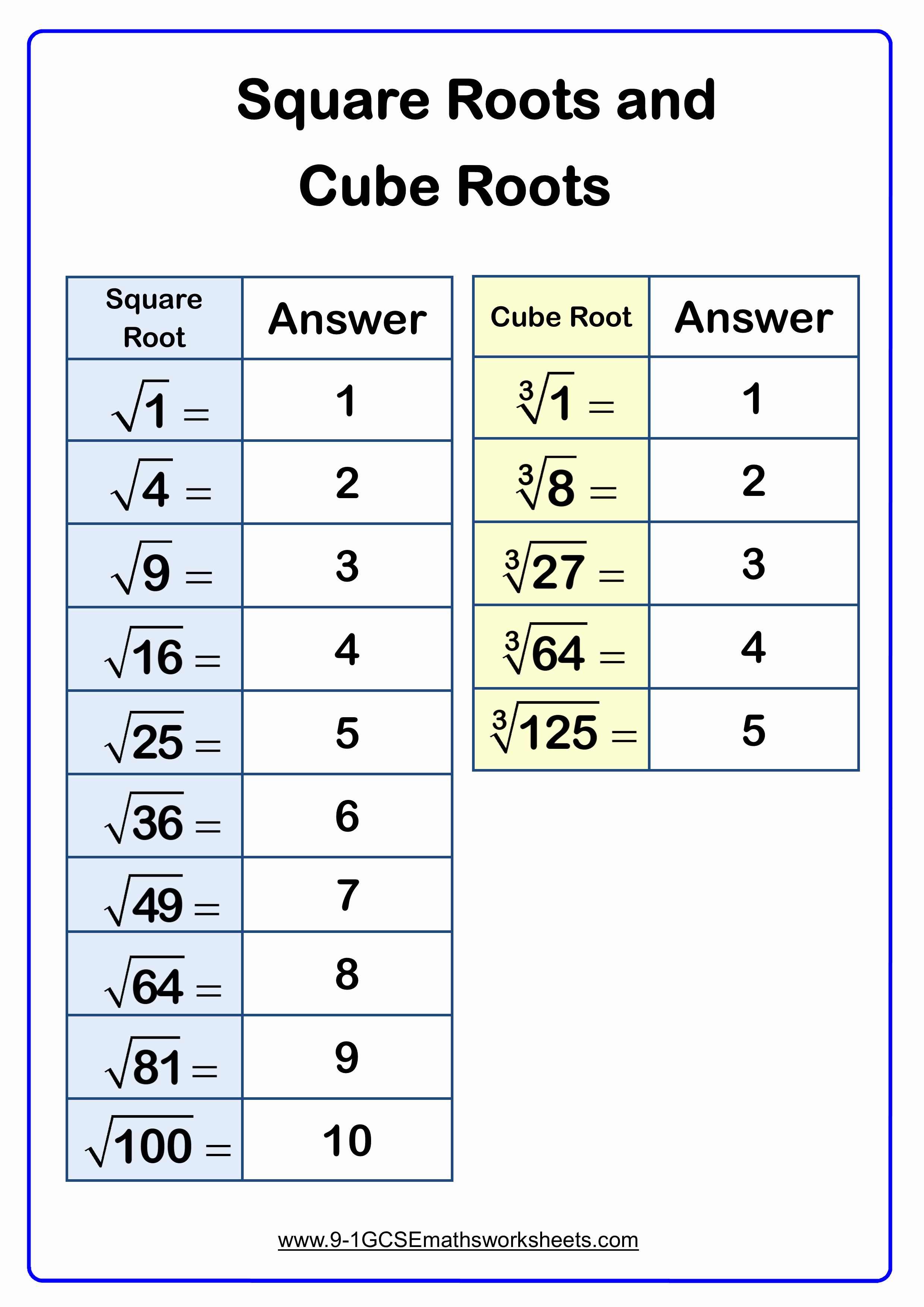
Before diving into calculations, it’s essential to understand the concept of square roots. A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4, because 4 × 4 = 16.

Worksheets:

• Complete the following table with the square roots of the given numbers:
  

  Number	Square Root
  25
  36
  49	___

Method 2: Simplifying Square Roots

Simplifying square roots involves finding the largest perfect square that divides the number. This method can help you solve equations more efficiently. For example, to simplify √48, you can break it down into √16 × √3, which simplifies to 4√3.

Worksheets:

• Simplify the following square roots:
  • √75 = __
  • √125 = __
  • √200 = ___

Method 3: Estimating Square Roots

Estimating square roots can be useful when you need a quick approximation. To estimate the square root of a number, find the nearest perfect square that is either higher or lower than the number. For example, to estimate √50, you can find the nearest perfect squares, which are 49 and 64. Since 50 is closer to 49, you can estimate the square root to be around 7.

Worksheets:

• Estimate the square roots of the following numbers:
  • √42 ≈ __
  • √67 ≈ __
  • √85 ≈ ___

Method 4: Solving Equations with Square Roots

Solving equations with square roots requires careful attention to the properties of square roots. For example, when solving √x = 5, you need to square both sides of the equation to isolate x, resulting in x = 25.

Worksheets:

• Solve the following equations:
  • √x = 3
  • √2x = 6
  • x + √x = 10

Method 5: Applying Square Roots to Real-World Problems

Applying square roots to real-world problems can help you see the relevance of these mathematical concepts. For example, if you need to calculate the length of a side of a square garden with an area of 256 square meters, you can use the square root function to find the answer.

Worksheets:

• A rectangular garden measures 10 meters by 5 meters. What is the length of the diagonal path through the garden?
• A water tank can hold 216 cubic meters of water. What is the length of the side of the tank if it is a cube?

📝 Note: Remember to check your calculations and show your work in the worksheets.

In conclusion, mastering square roots requires a combination of understanding the concept, simplifying, estimating, solving equations, and applying it to real-world problems. By practicing these methods with the accompanying worksheets, you’ll become more confident and proficient in your ability to work with square roots.

Related Terms:

• Bilangan
• Perkalian
• Matematika
• Akar bilangan
• Akar kuadrat
• LCM Worksheet pdf