Solving Two Step Inequalities Made Easy
Solving Two Step Inequalities Made Easy
When it comes to solving inequalities, many students find it challenging, especially when it involves multiple steps. Two-step inequalities are a common type of inequality that requires a bit more work to solve, but with the right approach, it can be made easy. In this article, we will explore the concept of two-step inequalities, provide examples, and offer a step-by-step guide on how to solve them.
What are Two-Step Inequalities?
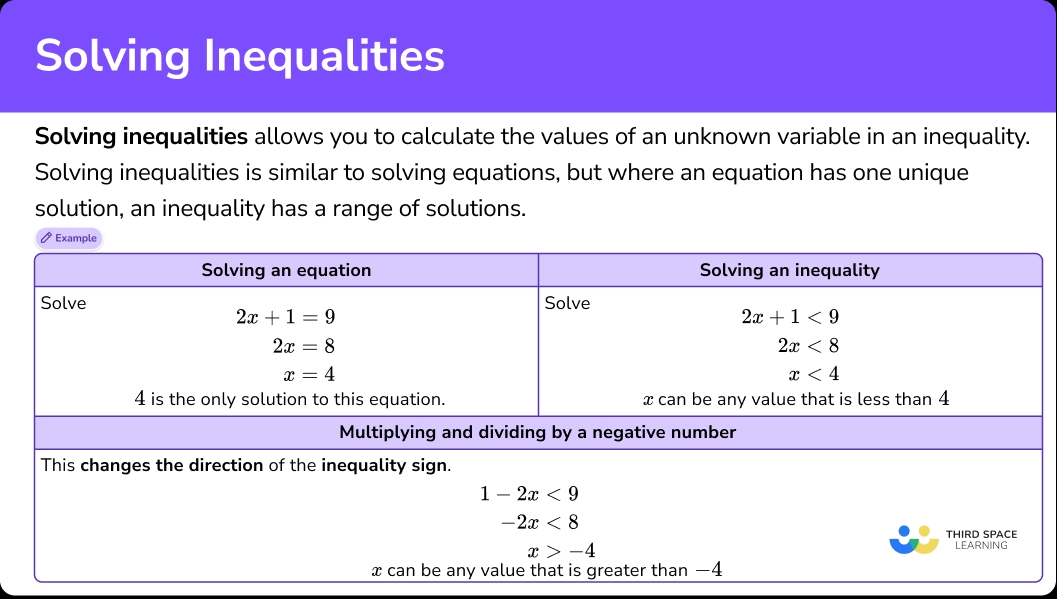
Two-step inequalities are inequalities that require two operations to solve. These inequalities typically involve addition, subtraction, multiplication, or division, and the operations must be performed in a specific order. The goal is to isolate the variable on one side of the inequality sign.
Examples of Two-Step Inequalities
Here are a few examples of two-step inequalities:
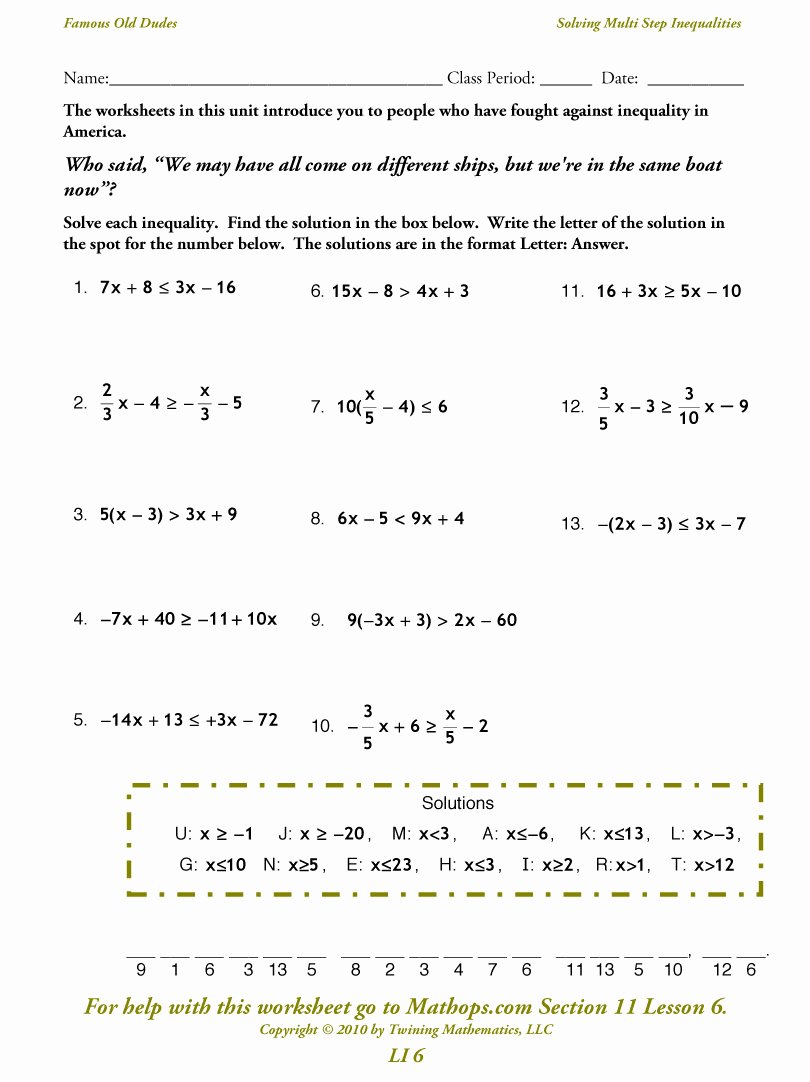
- 2x + 5 > 11
- x - 3 < 7
- 4x - 2 ≥ 16
- x/2 + 3 ≤ 9
Step-by-Step Guide to Solving Two-Step Inequalities
To solve two-step inequalities, follow these steps:
- Identify the operations involved: Look at the inequality and identify the operations that need to be performed. In the examples above, the operations involved are addition, subtraction, multiplication, and division.
- Perform the first operation: Perform the first operation to isolate the term with the variable. For example, in the inequality 2x + 5 > 11, subtract 5 from both sides to get 2x > 6.
- Perform the second operation: Perform the second operation to solve for the variable. In the inequality 2x > 6, divide both sides by 2 to get x > 3.
📝 Note: When solving inequalities, remember to flip the direction of the inequality sign when dividing or multiplying by a negative number.
More Examples and Solutions
Let’s solve a few more examples to illustrate the steps:
- Example 1: x - 3 < 7
- Add 3 to both sides: x < 10
- The solution is x < 10
- Example 2: 4x - 2 ≥ 16
- Add 2 to both sides: 4x ≥ 18
- Divide both sides by 4: x ≥ 4.5
- The solution is x ≥ 4.5
- Example 3: x/2 + 3 ≤ 9
- Subtract 3 from both sides: x/2 ≤ 6
- Multiply both sides by 2: x ≤ 12
- The solution is x ≤ 12
Using a Table to Organize Your Work
When solving two-step inequalities, it can be helpful to use a table to organize your work. Here’s an example:
| Inequality | Step 1 | Step 2 | Solution |
|---|---|---|---|
| 2x + 5 > 11 | Subtract 5 from both sides: 2x > 6 | Divide both sides by 2: x > 3 | x > 3 |
| x - 3 < 7 | Add 3 to both sides: x < 10 | - | x < 10 |
| 4x - 2 ≥ 16 | Add 2 to both sides: 4x ≥ 18 | Divide both sides by 4: x ≥ 4.5 | x ≥ 4.5 |
In Conclusion
Solving two-step inequalities requires attention to detail and a systematic approach. By following the steps outlined in this article, you can become proficient in solving these types of inequalities. Remember to identify the operations involved, perform the first operation to isolate the term with the variable, and then perform the second operation to solve for the variable.
What is the main difference between one-step and two-step inequalities?
+The main difference is that two-step inequalities require two operations to solve, whereas one-step inequalities require only one operation.
When solving inequalities, what happens when you multiply or divide by a negative number?
+When you multiply or divide by a negative number, you must flip the direction of the inequality sign.
Can you use a table to organize your work when solving two-step inequalities?
+Yes, using a table can be helpful in organizing your work and keeping track of the steps involved in solving the inequality.