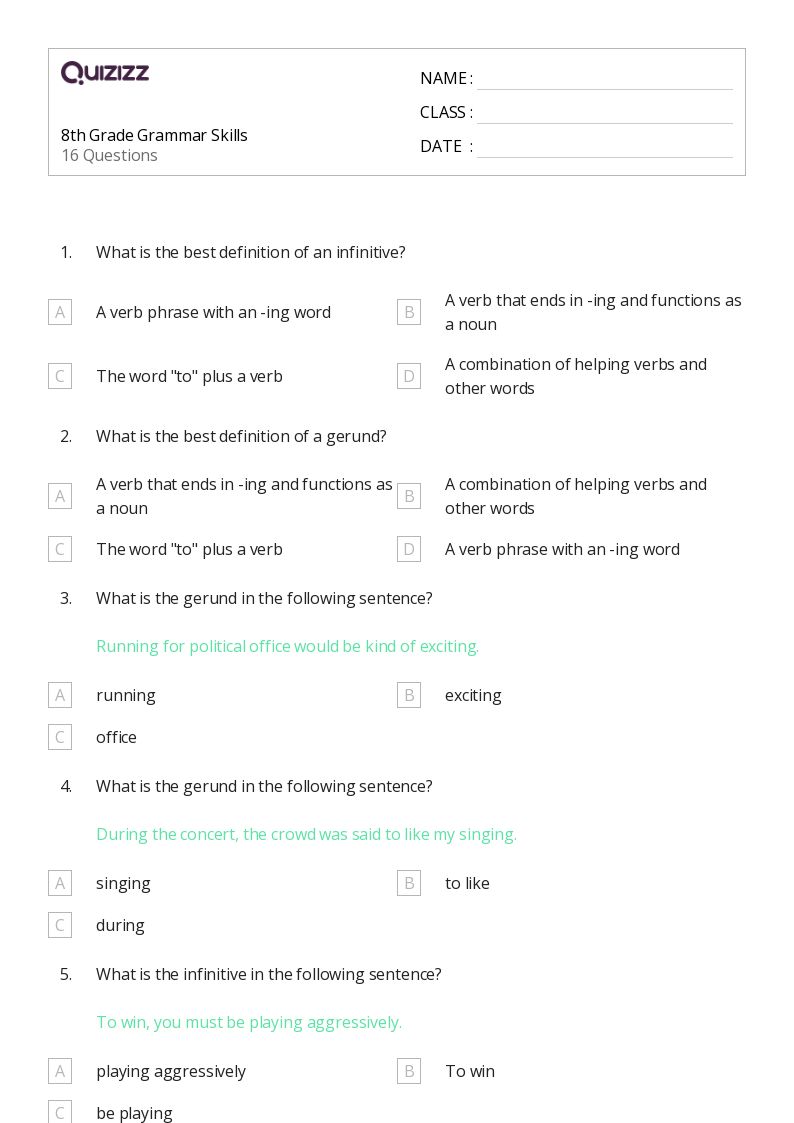
Solving Two-Step Equations Worksheets

Understanding Two-Step Equations
Two-step equations are a fundamental concept in algebra, and they can be a bit challenging for some students. However, with practice and patience, anyone can master solving two-step equations. In this blog post, we will provide a comprehensive guide on how to solve two-step equations, along with some examples and notes.
What are Two-Step Equations?
Two-step equations are linear equations that require two operations to isolate the variable. They are called “two-step” because you need to perform two mathematical operations to solve for the variable. These operations can be addition, subtraction, multiplication, or division.
How to Solve Two-Step Equations
To solve two-step equations, you need to follow the order of operations (PEMDAS):
- Parentheses: Evaluate any expressions inside parentheses.
- Exponents: Evaluate any exponential expressions.
- Multiplication and Division: Evaluate any multiplication and division operations from left to right.
- Addition and Subtraction: Evaluate any addition and subtraction operations from left to right.
Here’s a step-by-step guide on how to solve two-step equations:
Step 1: Add or Subtract to Isolate the Term with the Variable
Look at the equation and determine which operation to perform first. If the term with the variable has a coefficient (a number in front of the variable), you may need to add or subtract a constant to isolate the term.
Example: 2x + 5 = 11
In this equation, you need to subtract 5 from both sides to isolate the term with the variable.
🤔 Note: Always perform the same operation on both sides of the equation to maintain equality.
Step 2: Multiply or Divide to Solve for the Variable
Once you have isolated the term with the variable, you need to multiply or divide both sides of the equation to solve for the variable.
Example: 2x = 6
In this equation, you need to divide both sides by 2 to solve for x.
📝 Note: When multiplying or dividing both sides of an equation by a coefficient, make sure to simplify the fraction if possible.
Examples of Two-Step Equations
Here are some examples of two-step equations, along with their solutions:

| Equation | Solution |
|---|---|
| x + 3 = 7 | x = 4 |
| 2x - 2 = 6 | x = 4 |
| x/2 + 2 = 5 | x = 6 |
| 3x - 1 = 8 | x = 3 |
Worksheets for Practice
Here are some worksheets for you to practice solving two-step equations:
Worksheet 1:
Solve for x:
- x + 2 = 9
- 3x - 1 = 11
- x/2 + 3 = 7
- 2x + 5 = 13
Worksheet 2:
Solve for x:
- x - 4 = 9
- 2x + 2 = 12
- x/3 + 2 = 5
- 4x - 3 = 17
Conclusion
Solving two-step equations requires a solid understanding of algebraic concepts and the order of operations. With practice and patience, you can master solving two-step equations and improve your math skills. Remember to always follow the order of operations and perform the same operation on both sides of the equation to maintain equality.
What is the order of operations?
+The order of operations is a set of rules that tells you which operations to perform first when you have multiple operations in an expression. The order of operations is: Parentheses, Exponents, Multiplication and Division, and Addition and Subtraction.
How do I know which operation to perform first in a two-step equation?
+Look at the equation and determine which operation to perform first. If the term with the variable has a coefficient, you may need to add or subtract a constant to isolate the term.
Can I use a calculator to solve two-step equations?
+No, you should not use a calculator to solve two-step equations. Solving two-step equations requires a solid understanding of algebraic concepts and the order of operations. Using a calculator can help you check your answers, but it’s not a substitute for understanding the math.
Related Terms:
- 3 step Equations Worksheet