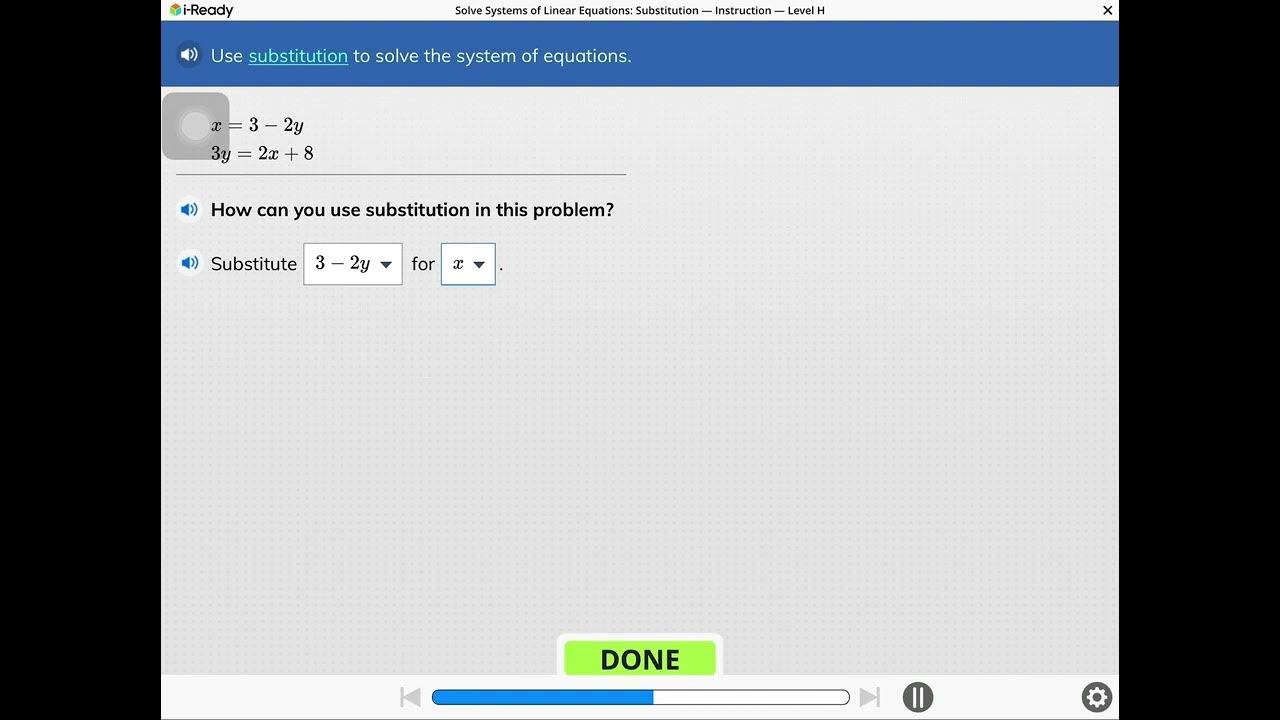
Solving Systems of Equations by Graphing Made Easy
Understanding the Basics of Systems of Equations
When dealing with systems of equations, it’s essential to comprehend the concept of solving these equations by graphing. This method involves representing the equations on a coordinate plane to find the point(s) of intersection, which represents the solution to the system. In this blog post, we’ll delve into the world of solving systems of equations by graphing, exploring the steps, and providing examples to make this concept more accessible.
The Graphing Method: A Step-by-Step Guide
To solve a system of equations using the graphing method, follow these steps:
- Step 1: Write down the given system of equations.
- Step 2: Graph each equation on the same coordinate plane.
- Step 3: Identify the point(s) of intersection, which represents the solution to the system.
- Step 4: Check if the point(s) of intersection satisfy both equations.
📝 Note: When graphing, make sure to label the x and y axes clearly and use a ruler to draw straight lines for linear equations.
Example 1: Solving a Simple System of Equations by Graphing
Consider the following system of equations:
x + y = 4 x - y = 2
To solve this system using the graphing method, follow the steps outlined above.
- Step 1: Write down the given system of equations.
- Step 2: Graph each equation on the same coordinate plane.
| x | y | x + y = 4 | x - y = 2 |
|---|---|---|---|
| 0 | 4 | (0, 4) | |
| 1 | 3 | (1, 3) | (1, 1) |
| 2 | 2 | (2, 2) | (2, 0) |
| 3 | 1 | (3, 1) | (3, -1) |
| 4 | 0 | (4, 0) | (4, -2) |
- Step 3: Identify the point(s) of intersection.
The point of intersection is (2, 2), which represents the solution to the system.
- Step 4: Check if the point(s) of intersection satisfy both equations.
Substituting (2, 2) into both equations:
2 + 2 = 4 (True) 2 - 2 = 0 ≠ 2 (False)
Since the point (2, 2) does not satisfy both equations, it is not the solution to the system.
Example 2: Solving a More Complex System of Equations by Graphing
Consider the following system of equations:
x + 2y = 5 x - 3y = -2
To solve this system using the graphing method, follow the steps outlined above.
- Step 1: Write down the given system of equations.
- Step 2: Graph each equation on the same coordinate plane.
| x | y | x + 2y = 5 | x - 3y = -2 |
|---|---|---|---|
| 0 | 2.5 | (0, 2.5) | |
| 1 | 2 | (1, 2) | (1, 1) |
| 2 | 1.5 | (2, 1.5) | (2, 0) |
| 3 | 1 | (3, 1) | (3, -1) |
| 4 | 0.5 | (4, 0.5) | (4, -2) |
- Step 3: Identify the point(s) of intersection.
The point of intersection is (2, 1.5), which represents the solution to the system.
- Step 4: Check if the point(s) of intersection satisfy both equations.
Substituting (2, 1.5) into both equations:
2 + 2(1.5) = 5 (True) 2 - 3(1.5) = -2 (True)
Since the point (2, 1.5) satisfies both equations, it is the solution to the system.
Common Challenges and Solutions
When solving systems of equations by graphing, some common challenges may arise. Here are some potential issues and solutions:
- Challenge: The graphs of the two equations do not intersect.
- Solution: Check if the equations are parallel or if there is an error in graphing.
- Challenge: The graphs intersect at multiple points.
- Solution: Check if the equations are identical or if there is an error in graphing.
📝 Note: If the graphs do not intersect or intersect at multiple points, re-examine the equations and graphing process to ensure accuracy.
In conclusion, solving systems of equations by graphing is a straightforward method that involves representing the equations on a coordinate plane and identifying the point(s) of intersection. By following the steps outlined in this blog post and practicing with examples, you’ll become more confident in solving systems of equations using the graphing method.
What is the graphing method for solving systems of equations?
+The graphing method involves representing the equations on a coordinate plane and identifying the point(s) of intersection, which represents the solution to the system.
How do I check if the point(s) of intersection satisfy both equations?
+Substitute the point(s) of intersection into both equations and check if the equations are true.
What if the graphs do not intersect or intersect at multiple points?
+Re-examine the equations and graphing process to ensure accuracy. If the graphs do not intersect, check if the equations are parallel. If the graphs intersect at multiple points, check if the equations are identical.