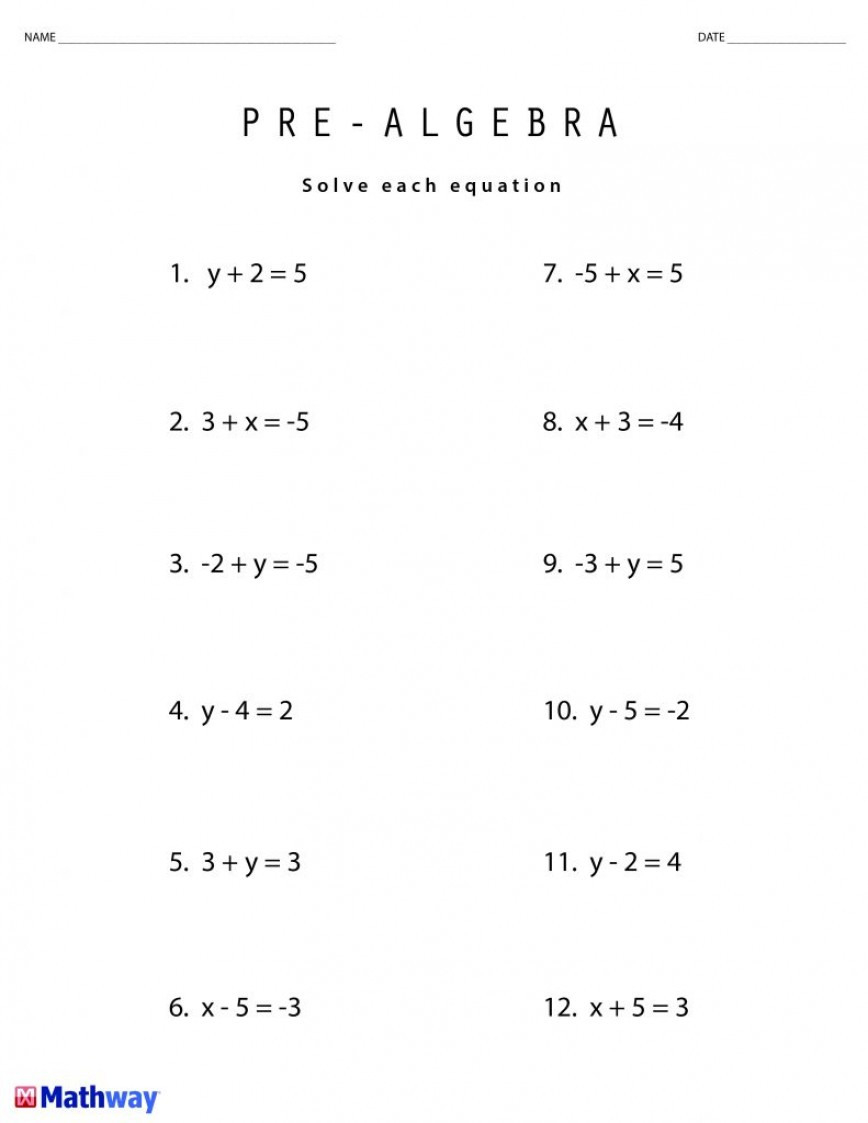Solve Systems with Ease: Equations Worksheet
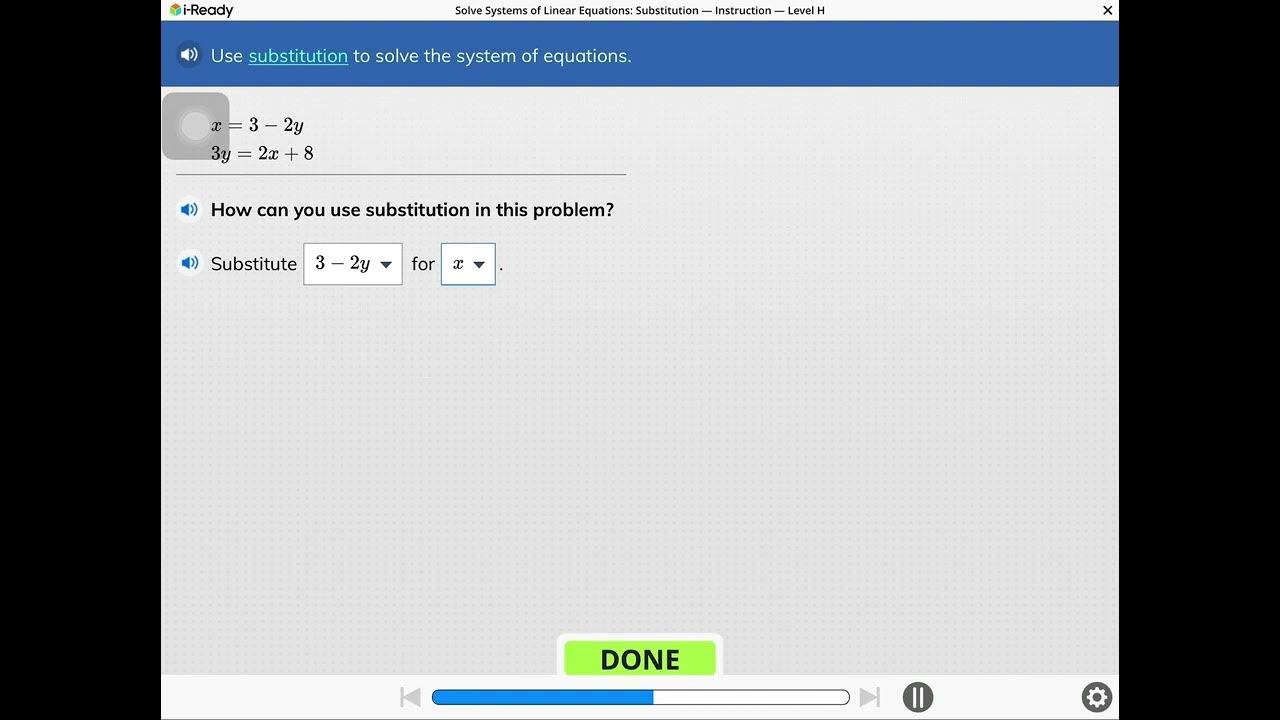
Introduction to Solving Systems of Equations
Solving systems of equations is a fundamental concept in mathematics, and it’s essential to master this skill to succeed in various fields, including physics, engineering, economics, and computer science. In this article, we’ll delve into the world of systems of equations, explore different methods for solving them, and provide a comprehensive worksheet to help you practice and reinforce your understanding.
What are Systems of Equations?
A system of equations is a collection of two or more equations that involve the same variables. These equations can be linear or nonlinear, and they can be represented graphically or algebraically. The goal is to find the values of the variables that satisfy all the equations simultaneously.
Types of Systems of Equations
There are several types of systems of equations, including:
- Linear Systems: These systems consist of linear equations, which can be represented in the form of ax + by = c, where a, b, and c are constants.
- Nonlinear Systems: These systems consist of nonlinear equations, which cannot be represented in the form of ax + by = c.
- Dependent Systems: These systems have an infinite number of solutions, as the equations are essentially the same.
- Independent Systems: These systems have a unique solution, as the equations are distinct.
- Inconsistent Systems: These systems have no solution, as the equations are contradictory.
Methods for Solving Systems of Equations
There are several methods for solving systems of equations, including:
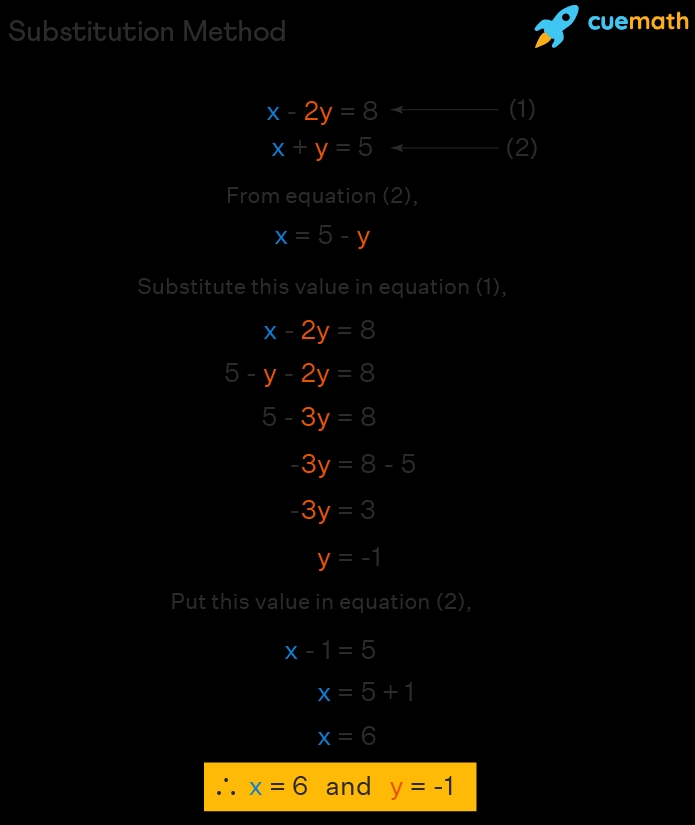
- Substitution Method: This method involves solving one equation for one variable and substituting the result into the other equation.
- Elimination Method: This method involves adding or subtracting the equations to eliminate one variable.
- Graphical Method: This method involves graphing the equations on a coordinate plane and finding the point of intersection.
- Matrices Method: This method involves using matrices to represent the system of equations and finding the inverse matrix to solve for the variables.
Worksheet: Solving Systems of Equations
Here’s a comprehensive worksheet to help you practice solving systems of equations:
| System of Equations | Method | Solution | |
|---|---|---|---|
| 2x + 3y = 7 | x - 2y = -3 | Substitution | (x, y) = (1, 2) |
| x + 2y = 4 | 3x - 2y = 5 | Elimination | (x, y) = (3, 1) |
| y = 2x - 1 | y = x + 3 | Graphical | (x, y) = (2, 3) |
| 2x + 3y = 7 | x - 2y = -3 | Matrices | (x, y) = (1, 2) |
📝 Note: For each system of equations, choose the most suitable method and solve for the variables. Check your answers and make sure they satisfy all the equations simultaneously.
Tips and Tricks
Here are some tips and tricks to help you solve systems of equations with ease:
- Read the problem carefully: Make sure you understand the system of equations and the variables involved.
- Choose the right method: Select the most suitable method for the system of equations, and make sure you understand the steps involved.
- Check your work: Verify your answers and make sure they satisfy all the equations simultaneously.
- Practice, practice, practice: The more you practice, the more comfortable you’ll become with solving systems of equations.
Systems of equations are a fundamental concept in mathematics, and mastering this skill takes practice and patience. With this comprehensive worksheet and the tips and tricks provided, you’ll be well on your way to solving systems of equations with ease.
As you continue to practice and reinforce your understanding, remember that solving systems of equations is a skill that can be applied to various real-world problems. Keep practicing, and soon you’ll become a master of solving systems of equations!
What is the difference between a linear and nonlinear system of equations?
+A linear system of equations consists of linear equations, which can be represented in the form of ax + by = c, where a, b, and c are constants. A nonlinear system of equations, on the other hand, consists of nonlinear equations, which cannot be represented in this form.
What is the substitution method for solving systems of equations?
+The substitution method involves solving one equation for one variable and substituting the result into the other equation.
What is the elimination method for solving systems of equations?
+The elimination method involves adding or subtracting the equations to eliminate one variable.