5 Ways to Solve Quadratics with Square Roots

Unlocking the Power of Square Roots in Solving Quadratic Equations

Quadratic equations are a fundamental concept in algebra, and solving them is a crucial skill for students to master. One of the most effective methods for solving quadratics is by using square roots. In this article, we will explore five ways to solve quadratics with square roots, providing you with a comprehensive understanding of this technique.

What are Quadratic Equations?

A quadratic equation is a polynomial equation of degree two, which means the highest power of the variable (usually x) is two. The general form of a quadratic equation is:

ax^2 + bx + c = 0

where a, b, and c are constants, and a cannot be zero.

What are Square Roots?

A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16. Square roots are denoted by the symbol √.

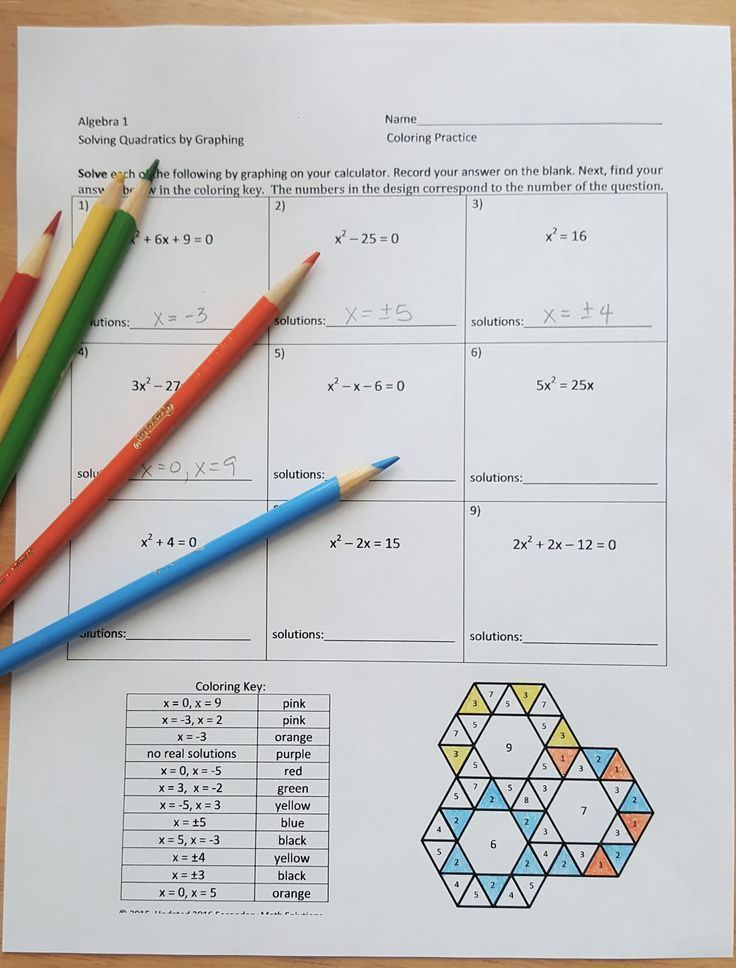
Method 1: Factoring Quadratics with Perfect Squares

One way to solve quadratics with square roots is by factoring the equation into the product of two binomials. This method works when the quadratic expression can be written as a perfect square trinomial.

For example, consider the quadratic equation:

x^2 + 6x + 9 = 0

We can factor this equation as:

(x + 3)(x + 3) = 0

Using the factored form, we can easily see that the solutions are x = -3.

Note: This method only works when the quadratic expression can be written as a perfect square trinomial.

📝 Note: When factoring quadratics, always check if the quadratic expression can be written as a perfect square trinomial.

Method 2: Using the Square Root Property

The square root property states that if x^2 = k, then x = ±√k. This property allows us to solve quadratic equations by taking the square root of both sides.

For example, consider the quadratic equation:

x^2 = 25

Using the square root property, we can take the square root of both sides:

x = ±√25

x = ±5

Therefore, the solutions are x = 5 and x = -5.

Method 3: Solving Quadratics with Square Roots and Factoring

Sometimes, we can solve quadratics by factoring the quadratic expression and then using the square root property.

For example, consider the quadratic equation:

x^2 + 5x + 6 = 0

We can factor this equation as:

(x + 3)(x + 2) = 0

Using the factored form, we can see that the solutions are x = -3 and x = -2. Alternatively, we can use the square root property to solve the equation:

x^2 + 5x + 6 = 0

x^2 + 5x = -6

x^2 = -5x - 6

x = ±√(-5x - 6)

x = ±√(-5x - 6)

In this case, the square root property does not provide a simple solution, and factoring is a more effective method.

Method 4: Using the Quadratic Formula with Square Roots

The quadratic formula is a general method for solving quadratic equations. The formula is:

x = (-b ± √(b^2 - 4ac)) / 2a

where a, b, and c are the coefficients of the quadratic equation.

For example, consider the quadratic equation:

x^2 + 2x + 1 = 0

Using the quadratic formula, we get:

x = (-2 ± √(2^2 - 4(1)(1))) / 2(1)

x = (-2 ± √(4 - 4)) / 2

x = (-2 ± √0) / 2

x = -1

Therefore, the solution is x = -1.

Method 5: Solving Quadratics with Square Roots and the Rational Root Theorem

The rational root theorem states that if a rational number p/q is a root of the polynomial, then p must be a factor of the constant term, and q must be a factor of the leading coefficient.

For example, consider the quadratic equation:

x^2 - 4x - 3 = 0

Using the rational root theorem, we can see that the possible rational roots are ±1 and ±3.

We can then use the square root property to solve the equation:

x^2 - 4x - 3 = 0

x^2 - 4x = 3

x^2 = 4x + 3

x = ±√(4x + 3)

x = ±√(4x + 3)

In this case, the square root property does not provide a simple solution, and using the rational root theorem to test possible roots is a more effective method.

In conclusion, solving quadratics with square roots is a powerful technique that can be used to solve a wide range of quadratic equations. By mastering the five methods outlined in this article, you will be able to solve quadratic equations with confidence and accuracy.