Mastering Quadratic Equations: A Comprehensive Guide
Quadratic equations are a fundamental concept in algebra, and mastering them is crucial for success in mathematics and various fields of science. In this article, we will delve into the world of quadratic equations, explore their forms, methods of solution, and provide a worksheet with answers to help you practice.
What are Quadratic Equations?
A quadratic equation is a polynomial equation of degree two, which means the highest power of the variable (usually x) is two. The general form of a quadratic equation is:
ax^2 + bx + c = 0
where a, b, and c are constants, and a cannot be zero.
Forms of Quadratic Equations
Quadratic equations can be expressed in various forms, including:
- Standard Form: ax^2 + bx + c = 0
- Factored Form: (x - r)(x - s) = 0, where r and s are the roots of the equation
- Vertex Form: a(x - h)^2 + k = 0, where (h, k) is the vertex of the parabola
Methods of Solving Quadratic Equations
There are several methods to solve quadratic equations, including:
- Factoring: expressing the equation in factored form and solving for the roots
- Quadratic Formula: using the formula x = (-b ± √(b^2 - 4ac)) / 2a to find the roots
- Graphing: graphing the equation on a coordinate plane and finding the x-intercepts
- Completing the Square: manipulating the equation to express it in vertex form and finding the roots
Quadratic Equations Worksheet
Here’s a worksheet with 10 quadratic equations for you to practice:
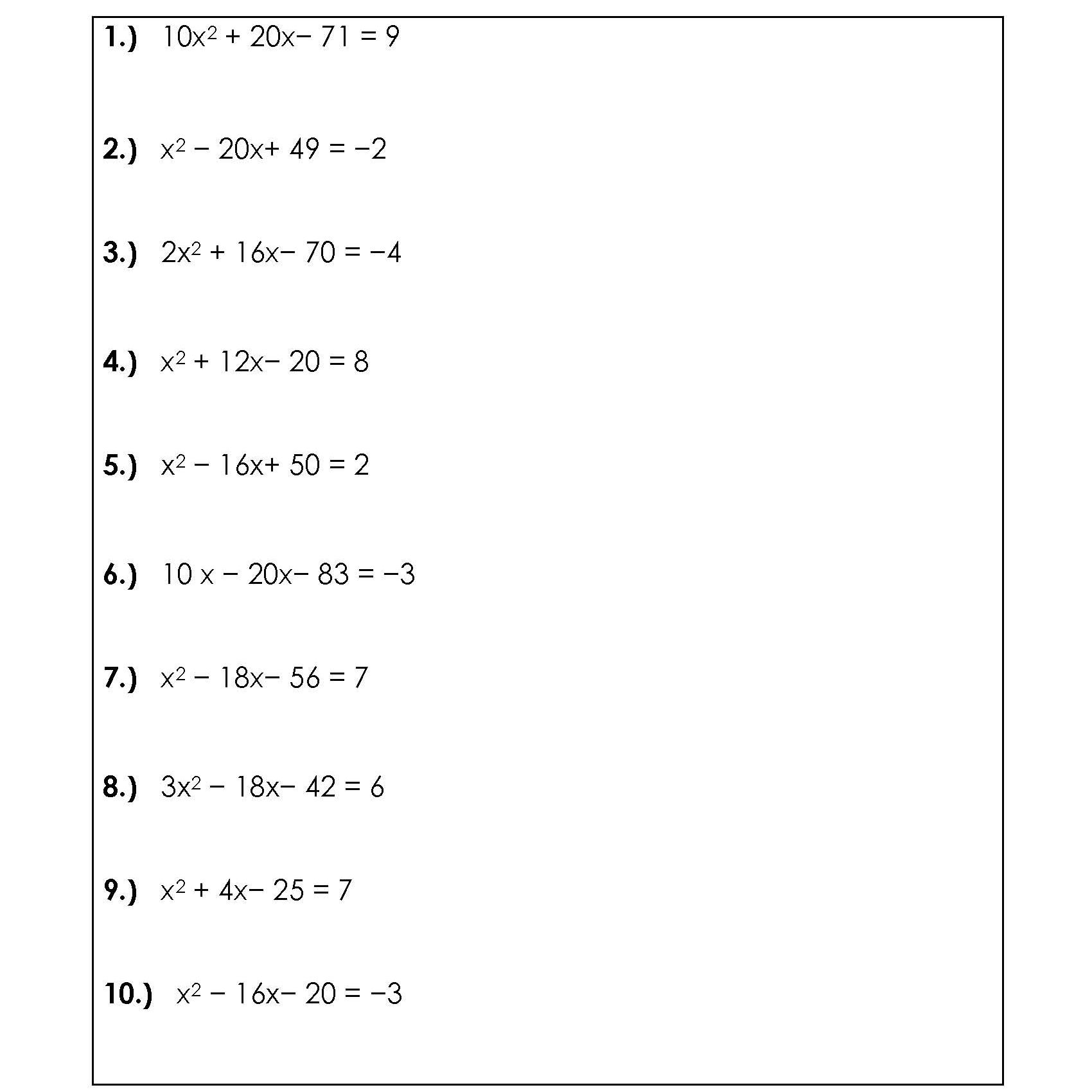
| Equation | Solution |
|---|---|
| x^2 + 5x + 6 = 0 | x = -2, x = -3 |
| x^2 - 4x - 3 = 0 | x = -1, x = 3 |
| 2x^2 + 7x + 3 = 0 | x = -1/2, x = -3 |
| x^2 - 2x - 6 = 0 | x = -2, x = 3 |
| 3x^2 + 2x - 5 = 0 | x = -1, x = 5/3 |
| x^2 + 3x - 4 = 0 | x = -4, x = 1 |
| 4x^2 - 5x - 1 = 0 | x = 1/4, x = -1 |
| 2x^2 + x - 3 = 0 | x = -3/2, x = 1 |
| x^2 - 5x + 6 = 0 | x = 2, x = 3 |
| 3x^2 + x + 2 = 0 | x = -1/3, x = -2 |
🤔 Note: Take your time to solve each equation, and use the methods discussed earlier to find the solutions.
Quadratic equations are a fundamental concept in mathematics, and mastering them is essential for success in various fields. With practice and patience, you can become proficient in solving quadratic equations and unlock the doors to more advanced mathematical concepts.
Now, let’s summarize the key points discussed in this article:
- Quadratic equations are polynomial equations of degree two.
- There are various forms of quadratic equations, including standard form, factored form, and vertex form.
- Methods of solving quadratic equations include factoring, quadratic formula, graphing, and completing the square.
- Practice is essential to become proficient in solving quadratic equations.
What is the general form of a quadratic equation?
+The general form of a quadratic equation is ax^2 + bx + c = 0, where a, b, and c are constants, and a cannot be zero.
What are the methods of solving quadratic equations?
+Methods of solving quadratic equations include factoring, quadratic formula, graphing, and completing the square.
Why is practice essential in solving quadratic equations?
+Practice is essential to become proficient in solving quadratic equations, as it helps to develop problem-solving skills and builds confidence in tackling more complex mathematical concepts.
Related Terms:
- Quadratic equation Worksheet pdf
- Quadratic equation methods
- Quadratic function Worksheet pdf
- Drawing quadratic graphs worksheet
