5 Easy Ways to Solve 2 Step Equations

Solving 2-Step Equations: A Step-by-Step Guide
When it comes to solving equations in algebra, 2-step equations are a great place to start. These equations require two operations to isolate the variable, and with the right strategies, you can solve them with ease. In this article, we’ll explore five easy ways to solve 2-step equations and provide you with examples and tips to help you master this essential math skill.
Understanding 2-Step Equations
A 2-step equation is an equation that requires two operations to isolate the variable. These operations can include addition, subtraction, multiplication, and division. For example:
2x + 5 = 11
To solve this equation, you need to perform two operations: subtract 5 from both sides and then divide both sides by 2.
Method 1: The "Inverse" Method
One way to solve 2-step equations is to use the “inverse” method. This involves performing the inverse operation of the one that is being applied to the variable. For example:
2x + 5 = 11
To solve this equation, you would subtract 5 from both sides (the inverse of adding 5) and then divide both sides by 2 (the inverse of multiplying by 2).
📝 Note: The inverse of addition is subtraction, and the inverse of multiplication is division.
Here’s the step-by-step solution:
2x + 5 = 11
Subtract 5 from both sides:
2x = 11 - 5 2x = 6
Divide both sides by 2:
x = 6 ÷ 2 x = 3
Method 2: The "Balance" Method
Another way to solve 2-step equations is to use the “balance” method. This involves performing the same operation on both sides of the equation to keep the equation balanced. For example:
2x - 3 = 7
To solve this equation, you would add 3 to both sides (to get rid of the -3) and then divide both sides by 2.
Here’s the step-by-step solution:
2x - 3 = 7
Add 3 to both sides:
2x = 7 + 3 2x = 10
Divide both sides by 2:
x = 10 ÷ 2 x = 5
Method 3: The "Working Backwards" Method
This method involves working backwards to isolate the variable. For example:
4x = 2x + 12
To solve this equation, you would subtract 2x from both sides (to get rid of the 2x) and then divide both sides by 4.
Here’s the step-by-step solution:
4x = 2x + 12
Subtract 2x from both sides:
2x = 12
Divide both sides by 2:
x = 12 ÷ 2 x = 6
Method 4: The "Using a Table" Method
This method involves using a table to organize the information and solve the equation. For example:
x - 2 = 9
To solve this equation, you would create a table with the variable and the constant term, and then use the table to find the solution.
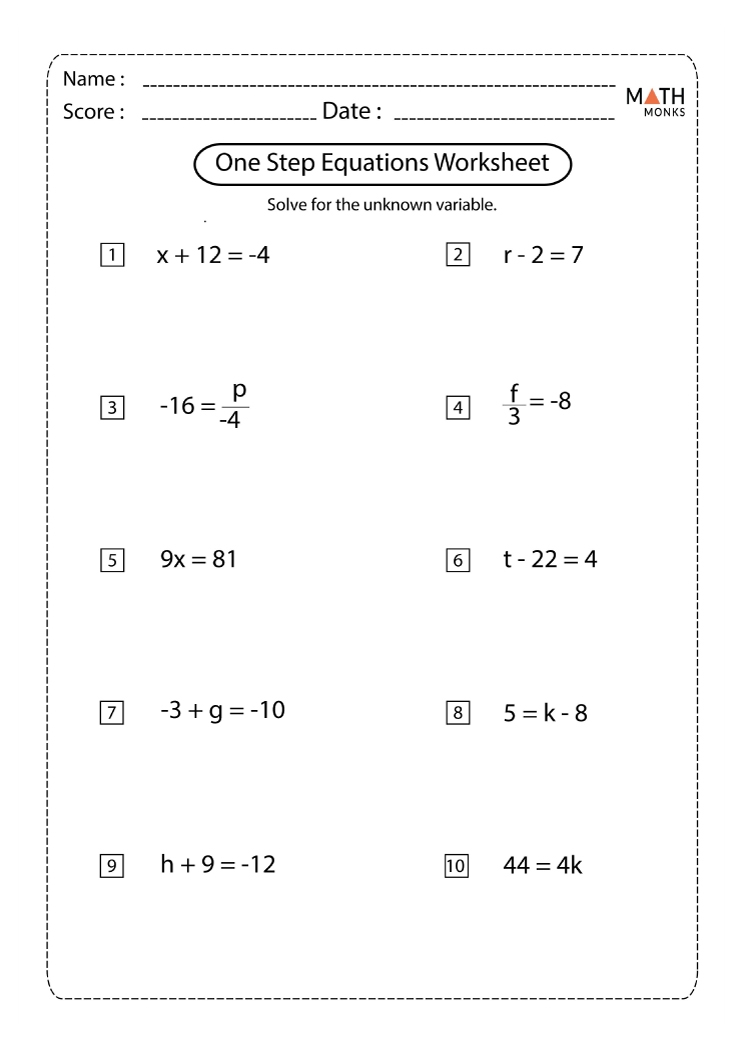
| Variable | Constant Term |
|---|---|
| x | -2 |
| x + 2 | 9 |
Using the table, you can see that the solution is x = 11.
Method 5: The "Using a Formula" Method
This method involves using a formula to solve the equation. For example:
x + 2 = 11
To solve this equation, you would use the formula:
x = 11 - 2
Here’s the step-by-step solution:
x + 2 = 11
Subtract 2 from both sides:
x = 11 - 2 x = 9
📝 Note: This method is useful when the equation has a simple solution, and you can easily spot the answer.
Conclusion
Solving 2-step equations requires a combination of skills, including understanding the equation, applying the inverse operations, and using different methods to isolate the variable. By mastering these methods, you’ll be able to solve 2-step equations with ease and confidence.
What is a 2-step equation?
+A 2-step equation is an equation that requires two operations to isolate the variable.
What are the different methods for solving 2-step equations?
+There are several methods for solving 2-step equations, including the “inverse” method, the “balance” method, the “working backwards” method, the “using a table” method, and the “using a formula” method.
How do I know which method to use?
+The method you use will depend on the specific equation and the operations involved. You can try different methods to see which one works best for you.