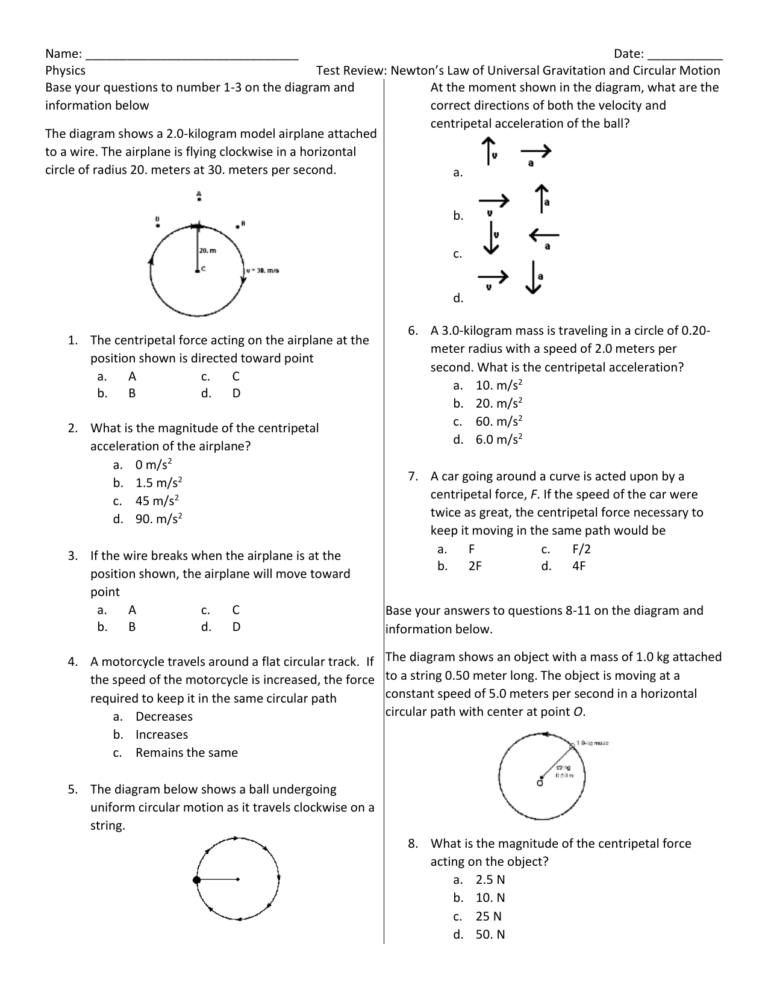
Simplify Linear Expressions Made Easy

Simplifying Linear Expressions: A Step-by-Step Guide

Simplifying linear expressions is a fundamental concept in algebra that can be a bit challenging for some students. However, with a clear understanding of the rules and steps involved, it can become a straightforward process. In this article, we will explore the world of linear expressions and provide a step-by-step guide on how to simplify them.

What is a Linear Expression?

A linear expression is a mathematical expression that consists of variables and constants combined using only addition, subtraction, and multiplication. It does not contain any exponents or roots. For example, 2x + 3, x - 4, and 5x - 2y are all linear expressions.

Rules for Simplifying Linear Expressions

To simplify a linear expression, you need to follow these basic rules:

• Combine like terms: Combine any terms that have the same variable and coefficient. For example, 2x + 3x can be combined to form 5x.
• Distribute coefficients: Distribute coefficients to the terms inside parentheses. For example, 2(x + 3) can be distributed to form 2x + 6.
• Remove parentheses: Remove any parentheses by distributing the coefficients and combining like terms.

Step-by-Step Guide to Simplifying Linear Expressions

Here is a step-by-step guide to simplifying linear expressions:

1. Combine like terms: Identify any terms that have the same variable and coefficient, and combine them.
2. Distribute coefficients: Distribute any coefficients to the terms inside parentheses.
3. Remove parentheses: Remove any parentheses by distributing the coefficients and combining like terms.
4. Simplify: Simplify the expression by combining any remaining like terms.

📝 Note: When simplifying linear expressions, it is essential to follow the order of operations (PEMDAS) to ensure accuracy.

Examples of Simplifying Linear Expressions

Let’s consider some examples of simplifying linear expressions:

• Example 1: Simplify the expression 2x + 3x - 4.
  • Combine like terms: 2x + 3x = 5x
  • Simplify: 5x - 4
• Example 2: Simplify the expression 2(x + 3) - 4.
  • Distribute coefficients: 2(x + 3) = 2x + 6
  • Simplify: 2x + 6 - 4 = 2x + 2
• Example 3: Simplify the expression x - 2(x - 3).
  • Distribute coefficients: -2(x - 3) = -2x + 6
  • Simplify: x - 2x + 6 = -x + 6

Common Mistakes to Avoid

When simplifying linear expressions, there are some common mistakes to avoid:

• Forgetting to combine like terms: Make sure to combine any terms that have the same variable and coefficient.
• Distributing coefficients incorrectly: Ensure that you distribute coefficients correctly to the terms inside parentheses.
• Not removing parentheses: Don’t forget to remove any parentheses by distributing the coefficients and combining like terms.

📝 Note: Practicing simplifying linear expressions regularly can help you become more comfortable and confident with the process.

Conclusion

Simplifying linear expressions is a fundamental concept in algebra that requires a clear understanding of the rules and steps involved. By following the step-by-step guide and avoiding common mistakes, you can become proficient in simplifying linear expressions. Remember to practice regularly to build your confidence and skills.