7 Ways to Master Circular Motion Problems
Understanding Circular Motion
Circular motion is a fundamental concept in physics that deals with the motion of objects in a circular path. It is a crucial aspect of physics, engineering, and mathematics, and is used to describe the motion of planets, electrons, and many other objects. Mastering circular motion problems requires a deep understanding of the underlying concepts and the ability to apply them to solve complex problems.
Key Concepts in Circular Motion
To master circular motion problems, you need to understand the following key concepts:
- Centripetal force: This is the force that acts towards the center of the circle, keeping the object in a circular path.
- Centripetal acceleration: This is the acceleration that occurs towards the center of the circle, and is caused by the centripetal force.
- Angular velocity: This is the rate of change of the angle of the object as it moves in a circular path.
- Tangential velocity: This is the velocity of the object as it moves in a circular path, and is perpendicular to the radius of the circle.
- Radius of curvature: This is the radius of the circle in which the object is moving.
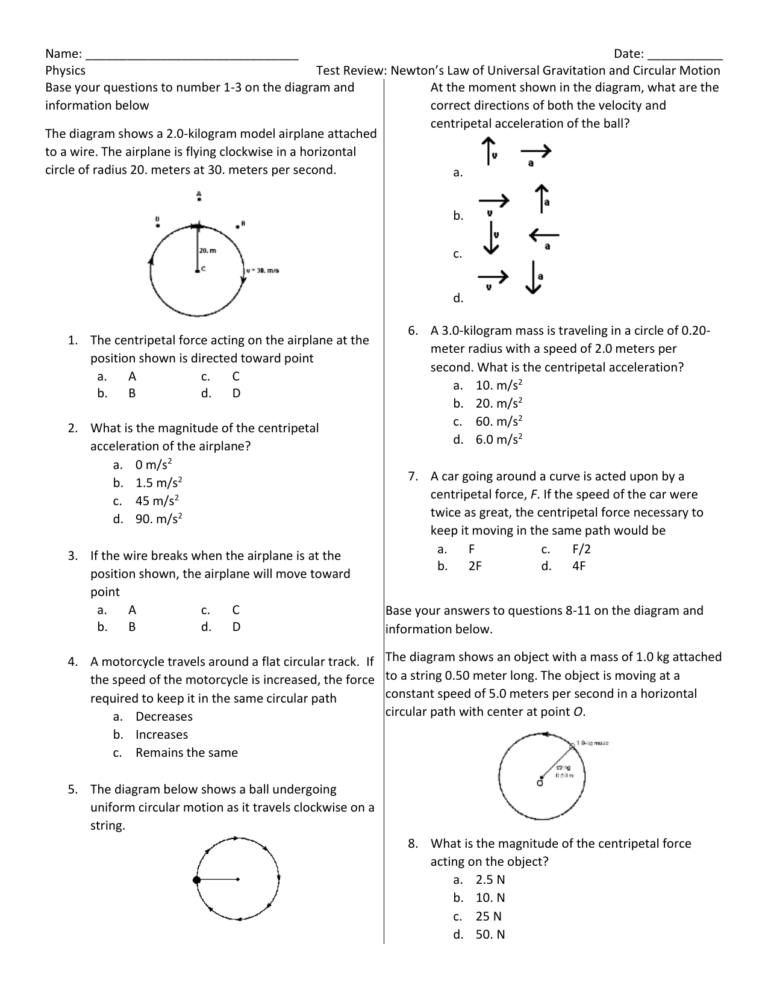
Step 1: Identify the Type of Problem
The first step in solving circular motion problems is to identify the type of problem you are dealing with. There are two main types of circular motion problems:
- Uniform circular motion: This is when the object moves in a circular path with a constant speed and radius.
- Non-uniform circular motion: This is when the object moves in a circular path with a changing speed and/or radius.
📝 Note: It's essential to identify the type of problem to apply the correct formulas and techniques.
Step 2: Draw a Diagram
Drawing a diagram is crucial in solving circular motion problems. It helps you visualize the problem and identify the key components, such as the radius of curvature, centripetal force, and tangential velocity.
- Draw the circle: Start by drawing the circle in which the object is moving.
- Label the components: Label the radius of curvature, centripetal force, and tangential velocity.
Step 3: Apply the Formulas
Once you have drawn the diagram and identified the key components, you can apply the formulas to solve the problem.
- Centripetal force formula: F = (m x v^2) / r
- Centripetal acceleration formula: a = v^2 / r
- Angular velocity formula: ω = v / r
- Tangential velocity formula: v = r x ω
💡 Note: Make sure to use the correct units and formulas to avoid errors.
Step 4: Solve for the Unknowns
Once you have applied the formulas, you can solve for the unknowns.
- Use algebra: Use algebra to solve for the unknowns, such as the radius of curvature, centripetal force, or tangential velocity.
- Check your units: Make sure to check your units to ensure that you have the correct answer.
Step 5: Check Your Answer
Finally, check your answer to ensure that it is correct.
- Use dimensional analysis: Use dimensional analysis to check that your answer has the correct units.
- Check the magnitude: Check the magnitude of your answer to ensure that it is reasonable.
Common Mistakes to Avoid
When solving circular motion problems, there are several common mistakes to avoid:
- Forgetting to convert units: Forgetting to convert units can lead to errors in your calculations.
- Using the wrong formula: Using the wrong formula can lead to incorrect answers.
- Not labeling the diagram: Not labeling the diagram can lead to confusion and errors.
🚫 Note: Avoid these common mistakes to ensure that you get the correct answer.
Practice Problems
To master circular motion problems, practice is key. Here are a few practice problems to get you started:
- A car is moving in a circular path with a radius of 20 m. If the car’s speed is 30 m/s, what is the centripetal force acting on the car?
- A bicycle is moving in a circular path with a radius of 10 m. If the bicycle’s speed is 20 m/s, what is the centripetal acceleration acting on the bicycle?
- A planet is moving in a circular path around the sun with a radius of 100 million km. If the planet’s speed is 20 km/s, what is the centripetal force acting on the planet?
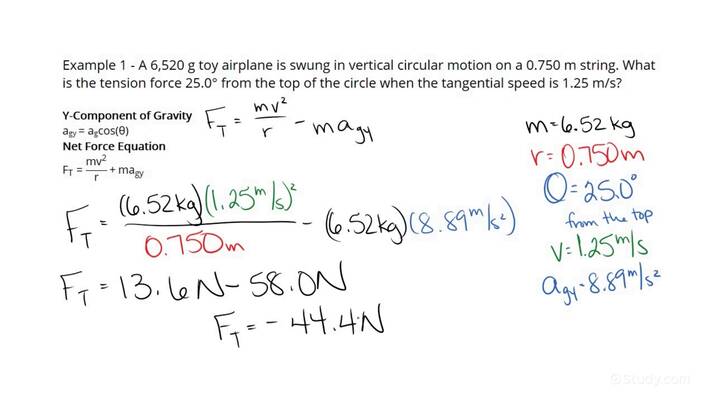
| Problem | Answer |
|---|---|
| Car in circular path | F = 450 N |
| Bicycle in circular path | a = 40 m/s^2 |
| Planet in circular path | F = 4 x 10^22 N |
In summary, mastering circular motion problems requires a deep understanding of the underlying concepts, the ability to apply formulas and techniques, and practice. By following these steps and avoiding common mistakes, you can become proficient in solving circular motion problems.
What is circular motion?
+Circular motion is the motion of an object in a circular path, such as the motion of a planet around the sun or the motion of a car around a curve.
What is centripetal force?
+Centripetal force is the force that acts towards the center of the circle, keeping the object in a circular path.
What is the formula for centripetal force?
+The formula for centripetal force is F = (m x v^2) / r, where m is the mass of the object, v is the velocity of the object, and r is the radius of the circle.
Related Terms:
- Centripetal force worksheet
- Circular motion questions
- Circular motion assignment