Simplify Expressions with Ease Worksheet Solutions
Simplifying Expressions: A Comprehensive Guide
Simplifying expressions is a fundamental concept in mathematics, and it’s essential to master this skill to solve complex equations and problems. In this post, we’ll delve into the world of simplifying expressions, explore the various techniques involved, and provide worksheet solutions to help you practice and reinforce your understanding.
What is an Expression?
An expression is a group of numbers, variables, and mathematical operations combined together. It can be a simple equation or a complex mathematical statement. Expressions can be numerical or algebraic, and they can be simplified using various techniques.
Why Simplify Expressions?
Simplifying expressions is crucial in mathematics because it helps to:
- Make complex equations more manageable
- Reduce errors and mistakes
- Improve problem-solving skills
- Enhance understanding of mathematical concepts
Techniques for Simplifying Expressions
There are several techniques involved in simplifying expressions, including:
- Combining like terms: This involves combining terms with the same variable and coefficient.
- Expanding and factoring: This involves expanding expressions using the distributive property and factoring out common terms.
- Simplifying fractions: This involves reducing fractions to their simplest form.
- Using order of operations: This involves following the correct order of operations to simplify expressions.
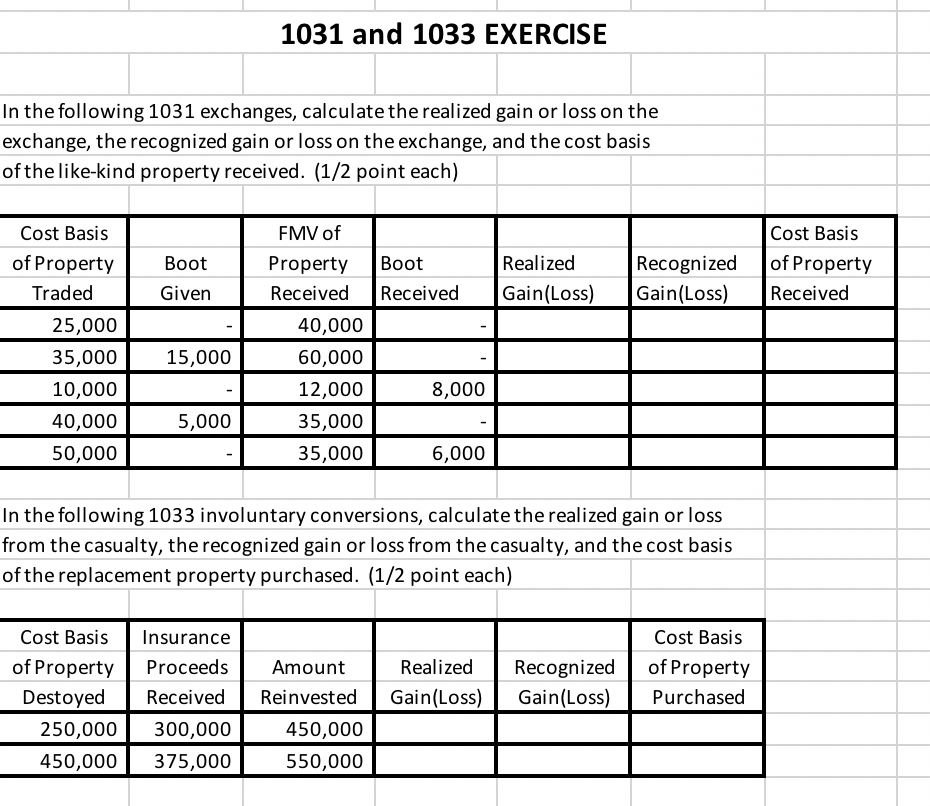
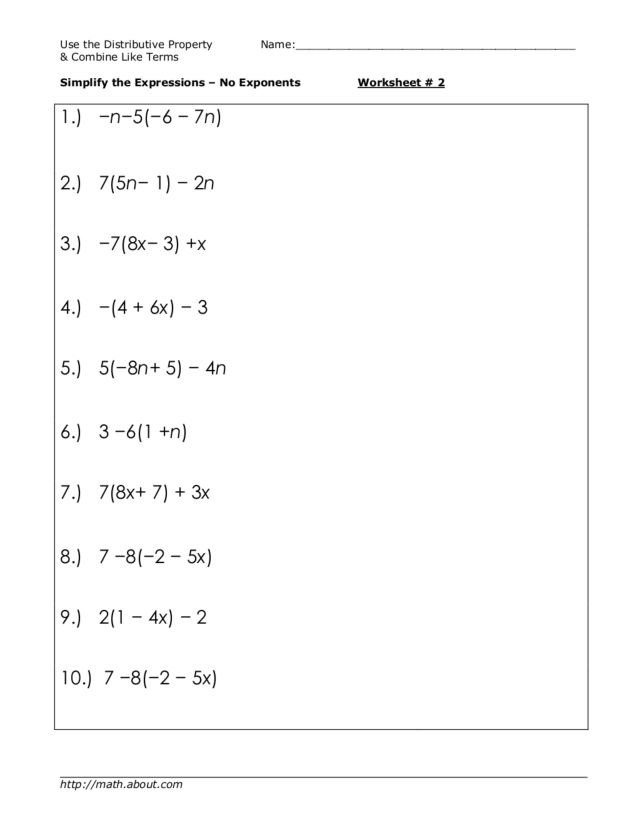
Worksheet Solutions
Here are some worksheet solutions to help you practice simplifying expressions:
Question 1: Simplify the expression: 2x + 5x - 3
Solution:
- Combine like terms: 2x + 5x = 7x
- Subtract 3: 7x - 3
Question 2: Simplify the expression: (3x + 2) + (2x - 1)
Solution:
- Expand and simplify: 3x + 2 + 2x - 1 = 5x + 1
Question 3: Simplify the expression: (2x^2 + 3x) / (x + 1)
Solution:
- Factor out common terms: (2x^2 + 3x) = x(2x + 3)
- Simplify fraction: x(2x + 3) / (x + 1) = (2x + 3)
Question 4: Simplify the expression: 2(x + 3) - 5
Solution:
- Expand and simplify: 2x + 6 - 5 = 2x + 1
Important Notes
📝 Note: When simplifying expressions, make sure to follow the order of operations and combine like terms carefully.
📝 Note: Always simplify fractions by reducing them to their simplest form.
Common Mistakes to Avoid
- Not combining like terms correctly: Make sure to combine terms with the same variable and coefficient.
- Not following the order of operations: Always follow the correct order of operations to simplify expressions.
- Not simplifying fractions correctly: Reduce fractions to their simplest form.
Conclusion
Simplifying expressions is an essential skill in mathematics, and with practice, you can master this skill. Remember to combine like terms, expand and factor, simplify fractions, and follow the order of operations. By avoiding common mistakes and practicing regularly, you’ll become proficient in simplifying expressions in no time.
What is an expression in mathematics?
+An expression is a group of numbers, variables, and mathematical operations combined together.
Why is simplifying expressions important in mathematics?
+Simplifying expressions helps to make complex equations more manageable, reduces errors and mistakes, improves problem-solving skills, and enhances understanding of mathematical concepts.
What are the techniques involved in simplifying expressions?
+The techniques involved in simplifying expressions include combining like terms, expanding and factoring, simplifying fractions, and using the order of operations.