Understanding Exponents: A Comprehensive Guide for Students
Exponents are a fundamental concept in mathematics, and mastering them is crucial for students to excel in various mathematical disciplines. However, many students find exponents challenging, and it’s not uncommon for them to struggle with understanding the basics. In this blog post, we will break down the concept of exponents, provide a step-by-step guide on how to work with them, and offer a free worksheet for students to practice.
What are Exponents?
Exponents are shorthand notation for repeated multiplication. For example, instead of writing 2 × 2 × 2 × 2, we can simply write 2^4. The number 2 is called the base, and the number 4 is called the exponent. In general, the expression a^n represents the product of a multiplied by itself n times.
Types of Exponents
There are several types of exponents, including:
- Positive exponents: These are the most common type of exponent and represent repeated multiplication. For example, 2^3 = 2 × 2 × 2 = 8.
- Negative exponents: These represent repeated division. For example, 2^-3 = 1 ÷ 2 ÷ 2 ÷ 2 = 1⁄8.
- Zero exponents: Any number raised to the power of zero is equal to 1. For example, 2^0 = 1.
- Fractional exponents: These represent a combination of multiplication and division. For example, 2^1⁄2 = √2.
How to Work with Exponents
Working with exponents involves a few simple rules:
- Product of powers: When multiplying two numbers with the same base, add the exponents. For example, 2^2 × 2^3 = 2^(2+3) = 2^5.
- Power of a power: When raising a number with an exponent to another power, multiply the exponents. For example, (2^2)^3 = 2^(2×3) = 2^6.
- Quotient of powers: When dividing two numbers with the same base, subtract the exponents. For example, 2^3 ÷ 2^2 = 2^(3-2) = 2^1.
📝 Note: It's essential to follow the order of operations (PEMDAS) when working with exponents.
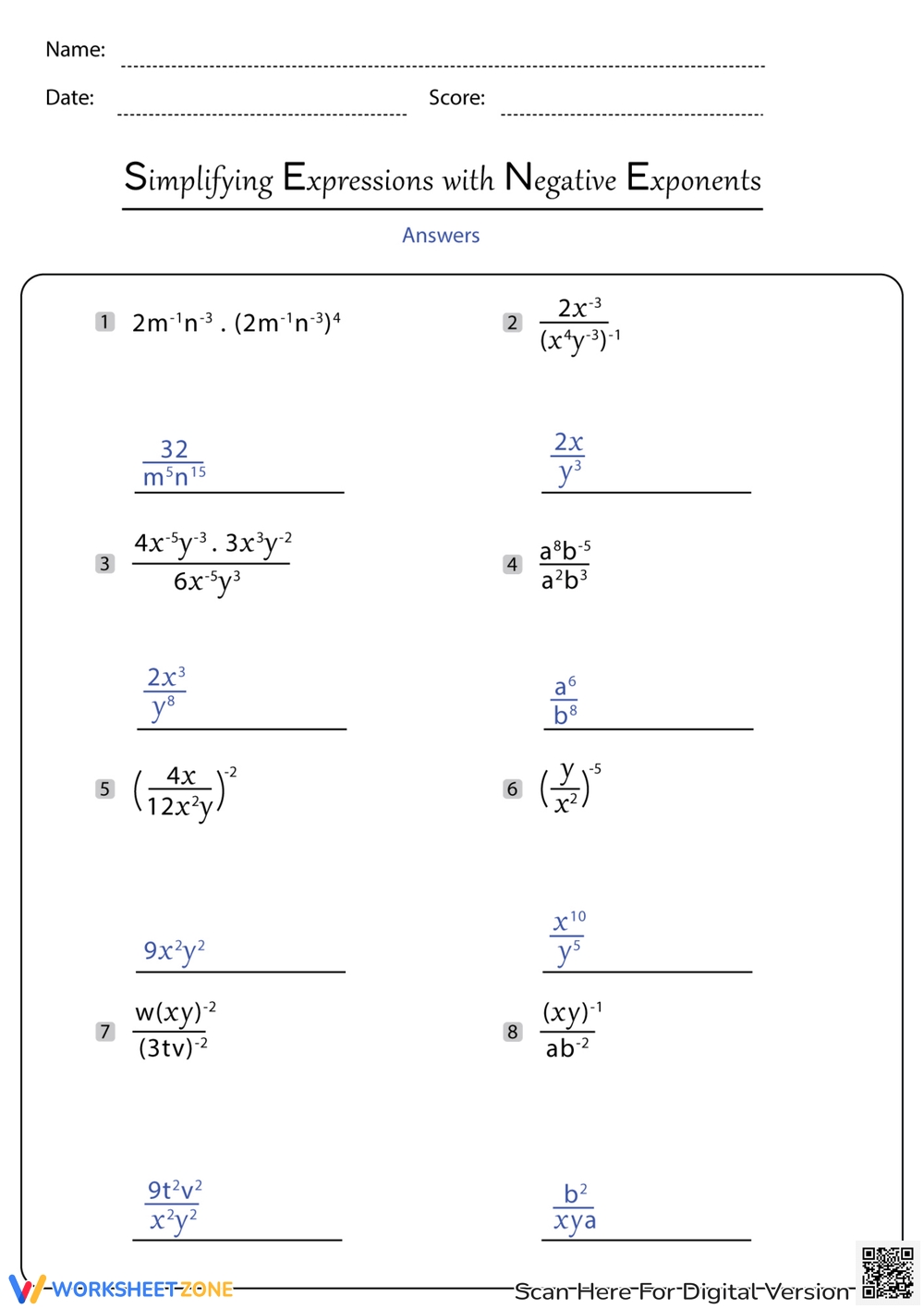
Free Worksheet for Students
Here is a free worksheet for students to practice working with exponents:
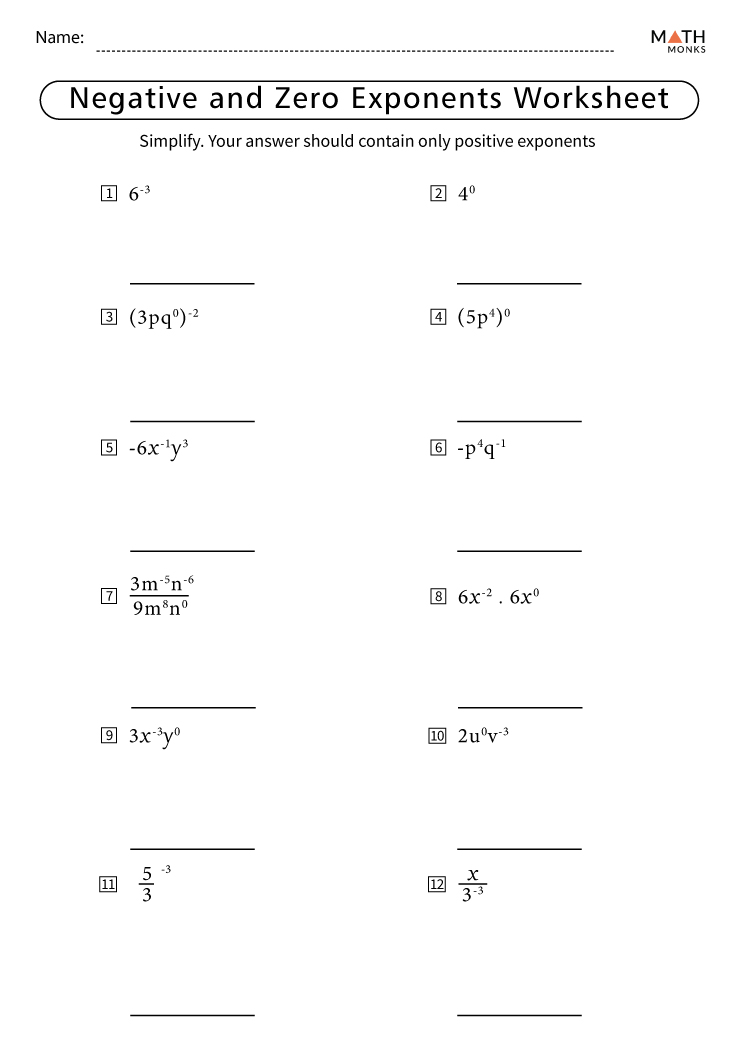
| Expression | Answer |
|---|---|
| 2^3 | ________ |
| 4^2 | ________ |
| 2^-2 | ________ |
| (3^2)^3 | ________ |
| 5^0 | ________ |
Conclusion
Exponents are a fundamental concept in mathematics, and mastering them is crucial for students to excel in various mathematical disciplines. By following the rules outlined in this blog post and practicing with the free worksheet, students can become proficient in working with exponents. Remember to always follow the order of operations and take your time when working with exponents.
What is the difference between positive and negative exponents?
+Positive exponents represent repeated multiplication, while negative exponents represent repeated division.
How do I simplify an expression with multiple exponents?
+Follow the order of operations and apply the rules of exponents, such as the product of powers and the power of a power.
Can I use a calculator to work with exponents?
+Yes, most calculators have an exponent key that allows you to easily calculate expressions with exponents.
