7 Significant Figures Rules to Master Accuracy

Understanding Significant Figures: A Guide to Mastering Accuracy

When working with numerical data, accuracy is crucial to ensure the reliability of the results. Significant figures are a fundamental concept in mathematics, science, and engineering that help maintain accuracy in calculations and measurements. In this article, we will delve into the world of significant figures, exploring their definition, importance, and the rules that govern their application.

What are Significant Figures?

Significant figures, also known as significant digits, are the digits in a numerical value that are known to be reliable and accurate. They are the digits that are certain and reliable, and they play a crucial role in determining the precision of a measurement or calculation.

Why are Significant Figures Important?

Significant figures are essential in various fields, including science, engineering, and mathematics, as they help maintain accuracy and precision in calculations and measurements. By using significant figures, individuals can:

• Ensure the reliability of results
• Avoid rounding errors
• Maintain consistency in calculations
• Improve communication and collaboration

7 Significant Figures Rules to Master Accuracy

To ensure accuracy and precision in calculations and measurements, it is essential to follow the rules of significant figures. Here are 7 significant figures rules to master:

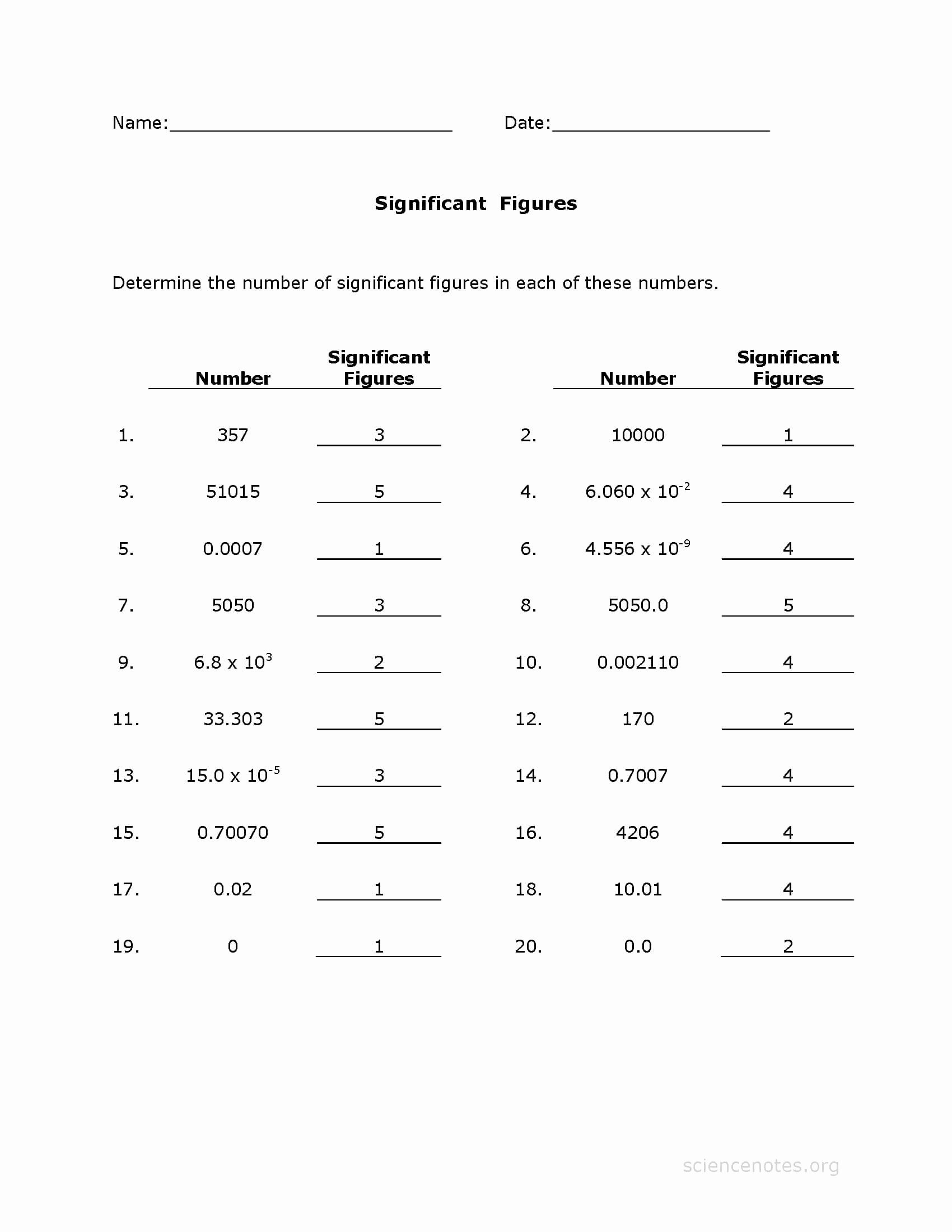
Rule 1: Non-Zero Digits are Always Significant

All non-zero digits in a numerical value are considered significant figures. For example, in the number 456, all three digits are significant.

Rule 2: Zeros Between Non-Zero Digits are Significant

Zeros that appear between non-zero digits are considered significant figures. For example, in the number 405, the zero is significant.

Rule 3: Leading Zeros are Not Significant

Leading zeros in a numerical value are not considered significant figures. For example, in the number 0.045, the leading zeros are not significant.

Rule 4: Trailing Zeros are Significant if the Number Contains a Decimal Point

Trailing zeros in a numerical value are considered significant figures if the number contains a decimal point. For example, in the number 45.00, the trailing zeros are significant.

Rule 5: Trailing Zeros are Not Significant if the Number Does Not Contain a Decimal Point

Trailing zeros in a numerical value are not considered significant figures if the number does not contain a decimal point. For example, in the number 4500, the trailing zeros are not significant.

Rule 6: Rounding Numbers to the Correct Number of Significant Figures

When rounding numbers, it is essential to round to the correct number of significant figures. For example, if a number has 3 significant figures, it should be rounded to 3 significant figures.

Rule 7: Significant Figures in Calculations

In calculations, the number of significant figures in the result is determined by the number of significant figures in the original numbers. For example, if two numbers with 3 significant figures are multiplied, the result should have 3 significant figures.

📝 Note: When performing calculations, it is essential to keep track of the number of significant figures in each step to ensure accuracy and precision.

Conclusion

Mastering significant figures is crucial in maintaining accuracy and precision in calculations and measurements. By understanding the 7 significant figures rules outlined in this article, individuals can ensure the reliability of their results and avoid errors. Remember, significant figures are not just a trivial concept; they are a fundamental aspect of mathematics, science, and engineering.

Related Terms:

• Significant figures exercises With answers
• Significant figures exam
• Significant figures Corbettmaths answers
• 107.854 significant figures