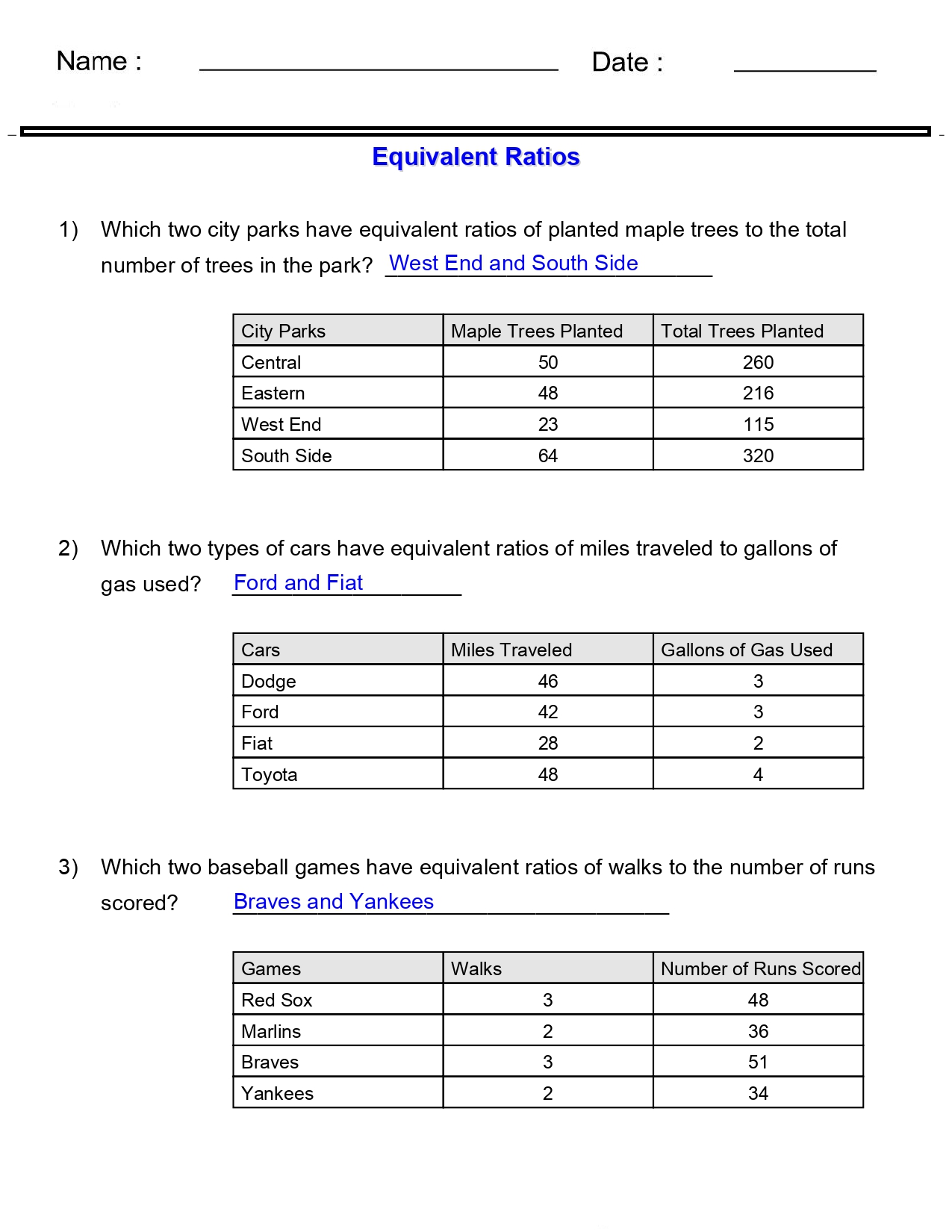
5 Ways to Master Rotations with Answer Key

Mastering Rotations: A Comprehensive Guide
Rotations are a fundamental concept in mathematics, particularly in geometry and trigonometry. Understanding how to perform rotations is crucial for problem-solving in various fields, including physics, engineering, and computer science. In this article, we will explore five ways to master rotations, providing a comprehensive guide for students and professionals alike.
Understanding the Basics of Rotations
Before diving into the methods for mastering rotations, it’s essential to understand the basics. A rotation is a transformation that turns a figure around a fixed point, known as the rotation center. The amount of rotation is measured in degrees, with 360 degrees representing a full rotation.
Method 1: Using Rotation Matrices
One way to master rotations is by using rotation matrices. A rotation matrix is a mathematical representation of a rotation transformation. It’s a 2x2 matrix that can be used to rotate a point or a vector around the origin.
| Rotation Matrix | Description |
|---|---|
| Rotation matrix for 90 degrees counterclockwise | |
| Rotation matrix for 180 degrees counterclockwise |
By multiplying a point or a vector by a rotation matrix, you can perform the corresponding rotation transformation.
📝 Note: Rotation matrices can be used to perform rotations in 2D and 3D spaces.
Method 2: Using Trigonometry
Another way to master rotations is by using trigonometry. Trigonometric functions, such as sine and cosine, can be used to calculate the coordinates of a point after a rotation.
These formulas can be used to rotate a point by an angle θ counterclockwise.
Method 3: Using Complex Numbers
Complex numbers can also be used to master rotations. A complex number can be represented as a point in the complex plane, and rotations can be performed by multiplying the complex number by a complex exponential.
This formula can be used to rotate a complex number by an angle θ counterclockwise.
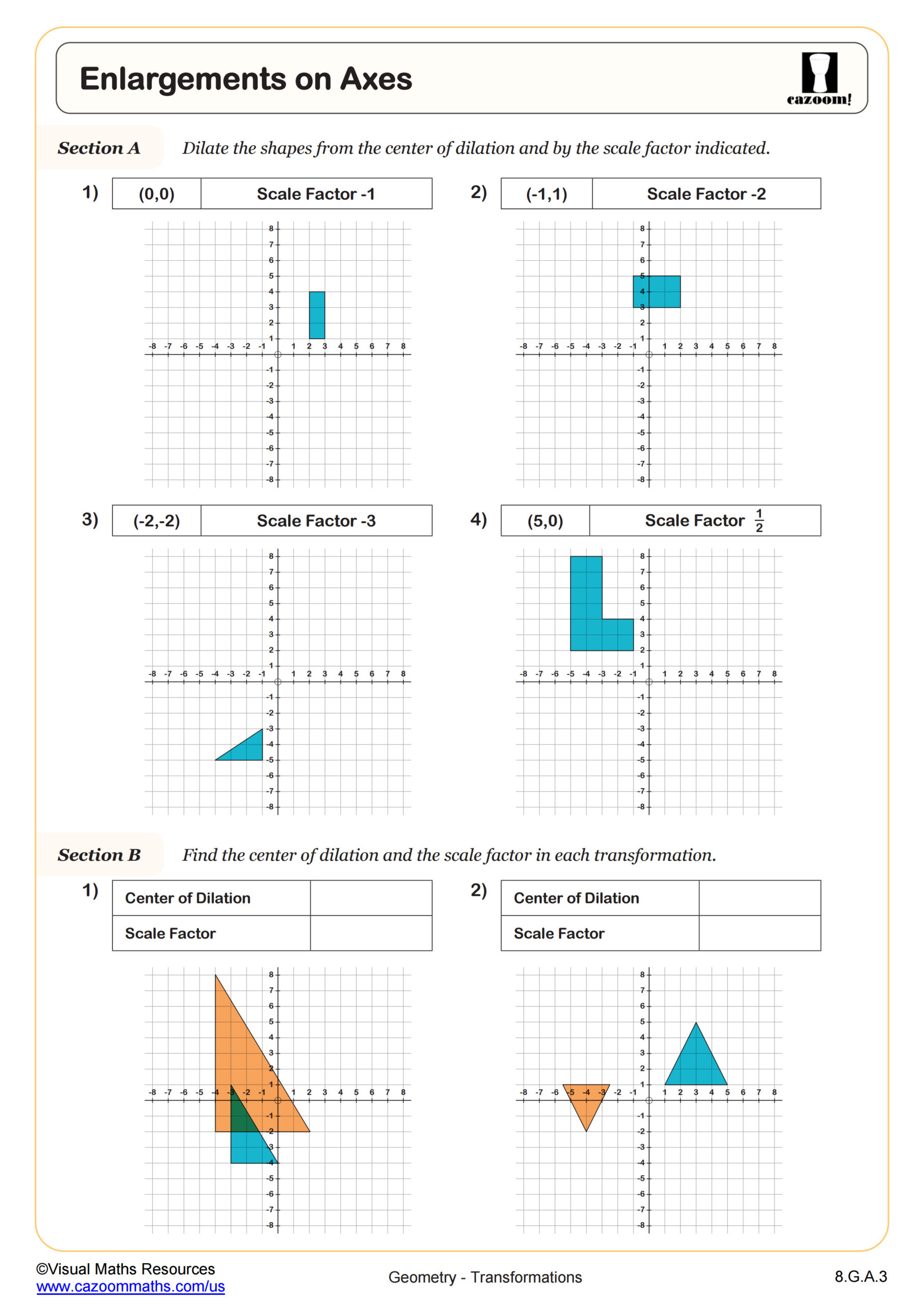
Method 4: Using Geometric Transformations
Geometric transformations, such as reflections and translations, can also be used to master rotations. A rotation can be performed by reflecting a point across a line, followed by a translation.
📝 Note: Geometric transformations can be used to perform rotations in 2D and 3D spaces.
Method 5: Using Computer Algebra Systems
Computer algebra systems, such as Mathematica or Maple, can also be used to master rotations. These systems can be used to perform symbolic calculations and visualize rotations.
📝 Note: Computer algebra systems can be used to perform rotations in 2D and 3D spaces.
In conclusion, mastering rotations requires a combination of mathematical techniques and geometric transformations. By using rotation matrices, trigonometry, complex numbers, geometric transformations, and computer algebra systems, you can develop a deep understanding of rotations and apply them to various fields.
What is the difference between a rotation matrix and a rotation transformation?
+A rotation matrix is a mathematical representation of a rotation transformation. A rotation transformation is the actual process of rotating a point or a vector.
Can rotations be performed in 3D space?
+What is the relationship between rotations and reflections?
+Rotations and reflections are both geometric transformations. A rotation can be performed by reflecting a point across a line, followed by a translation.
Related Terms:
- Dilation worksheet with answers pdf
- Transformation Worksheet pdf
- Reflection worksheet
- Rotation formula
- IGCSE transformation worksheet pdf