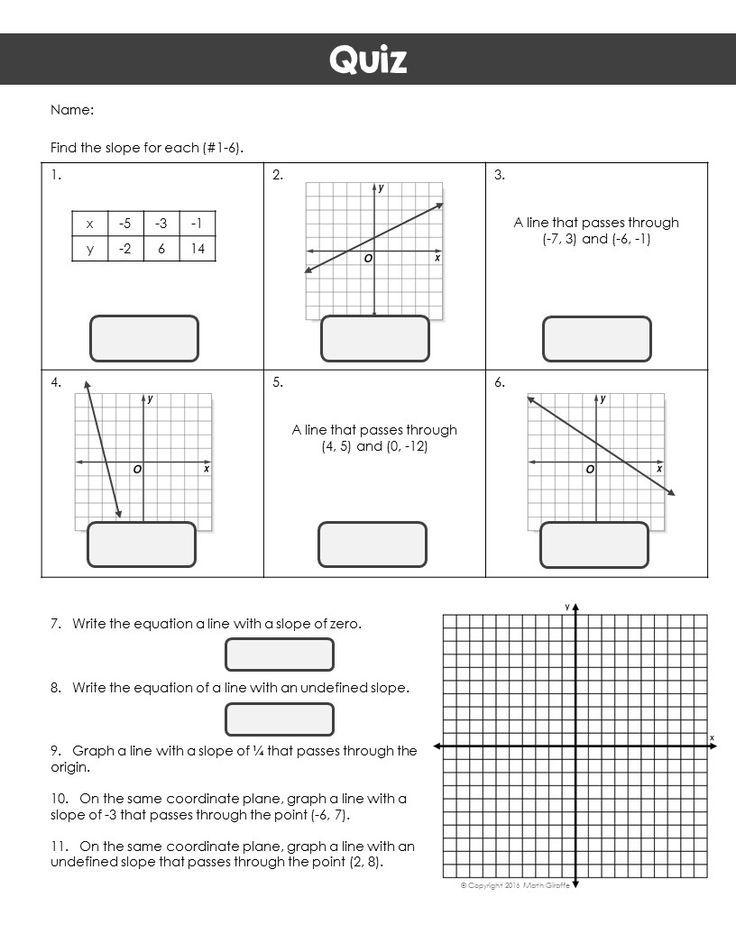
Rigid Transformation Worksheets for Math Students
Understanding Rigid Transformations: A Comprehensive Guide for Math Students
Rigid transformations are a fundamental concept in mathematics, particularly in geometry and spatial reasoning. These transformations involve moving objects in a plane or space without changing their shape or size. In this article, we will delve into the world of rigid transformations, exploring their definition, types, and importance in mathematics. We will also provide worksheets and examples to help students understand and apply these concepts.
What are Rigid Transformations?
Rigid transformations are movements that preserve the shape and size of an object. These transformations can be described as:
- Translations: Moving an object from one location to another without rotating or reflecting it.
- Rotations: Turning an object around a fixed point without changing its shape or size.
- Reflections: Flipping an object over a line or plane without altering its shape or size.
These transformations are called “rigid” because they do not change the object’s shape or size, only its position or orientation.
Types of Rigid Transformations
There are three primary types of rigid transformations:
Translations
Translations involve moving an object from one location to another without rotating or reflecting it. This can be represented by a vector, which shows the direction and distance of the movement.
- Example: Moving a triangle 3 units to the right and 2 units up.
Rotations
Rotations involve turning an object around a fixed point without changing its shape or size. The angle of rotation can be measured in degrees.
- Example: Rotating a square by 90 degrees clockwise around its center.
Reflections
Reflections involve flipping an object over a line or plane without altering its shape or size.
- Example: Reflecting a triangle over the x-axis.
Importance of Rigid Transformations
Rigid transformations are essential in mathematics and real-life applications, such as:
- Geometry: Rigid transformations help us understand and describe the properties of shapes and objects.
- Computer-Aided Design (CAD): Rigid transformations are used to create and manipulate 3D models.
- Physics and Engineering: Rigid transformations are used to describe the motion of objects in space.
Rigid Transformation Worksheets
Here are some worksheets to help students practice and apply rigid transformations:
Worksheet 1: Translations
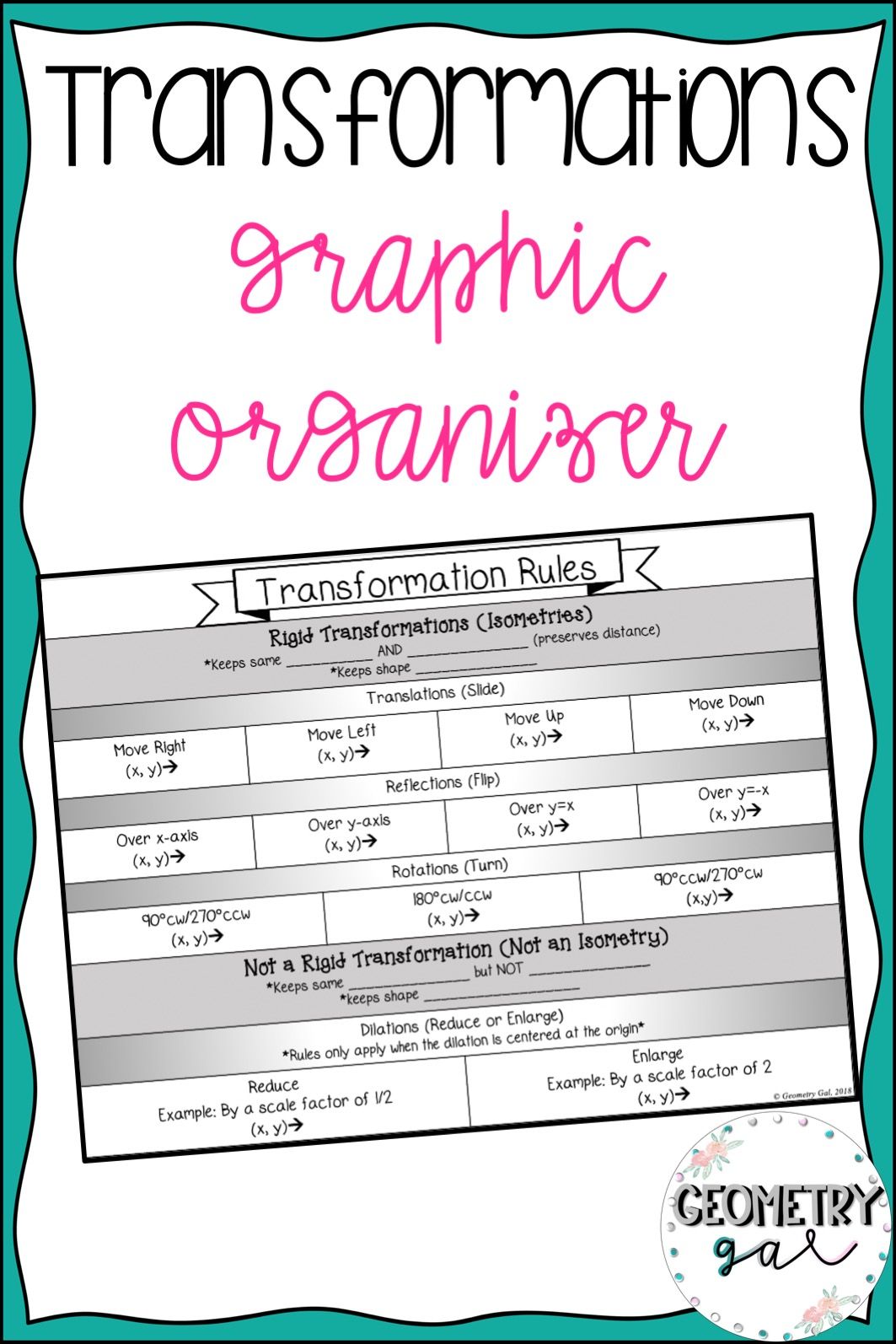
| Object | Translation Vector | New Position |
|---|---|---|
| Triangle | (3, 2) | (3 + x, 2 + y) |
| Square | (-2, 1) | (-2 + x, 1 + y) |
| Circle | (1, -1) | (1 + x, -1 + y) |
Worksheet 2: Rotations
| Object | Angle of Rotation | New Position |
|---|---|---|
| Triangle | 90 degrees | (x, -y) |
| Square | 180 degrees | (-x, -y) |
| Circle | 270 degrees | (-y, x) |
Worksheet 3: Reflections
| Object | Line of Reflection | New Position |
|---|---|---|
| Triangle | x-axis | (x, -y) |
| Square | y-axis | (-x, y) |
| Circle | x = 2 | (4 - x, y) |
💡 Note: These worksheets are meant to be used as a starting point for students to practice and apply rigid transformations. Teachers can adjust the complexity and difficulty level to suit their students' needs.
Conclusion
Rigid transformations are a fundamental concept in mathematics that can seem daunting at first, but with practice and application, students can master these concepts. By understanding translations, rotations, and reflections, students can develop their spatial reasoning and problem-solving skills. We hope these worksheets and examples have helped students and teachers alike to explore and apply rigid transformations in mathematics.
What is the difference between a translation and a rotation?
+A translation involves moving an object from one location to another without rotating or reflecting it, while a rotation involves turning an object around a fixed point without changing its shape or size.
Can a reflection be considered a type of rotation?
+No, a reflection is not considered a type of rotation. While both transformations involve changing the orientation of an object, a reflection involves flipping an object over a line or plane, whereas a rotation involves turning an object around a fixed point.
How are rigid transformations used in real-life applications?
+Rigid transformations are used in various real-life applications, such as computer-aided design (CAD), physics, engineering, and architecture. They help us understand and describe the properties of shapes and objects, and are essential for creating and manipulating 3D models.