Radius And Diameter Worksheet

Understanding Radius and Diameter: A Comprehensive Guide

Geometry is an essential part of mathematics, and understanding the concepts of radius and diameter is crucial for solving various problems. In this article, we will delve into the world of radius and diameter, explore their definitions, and provide a worksheet to help you practice and reinforce your knowledge.

What is Radius?

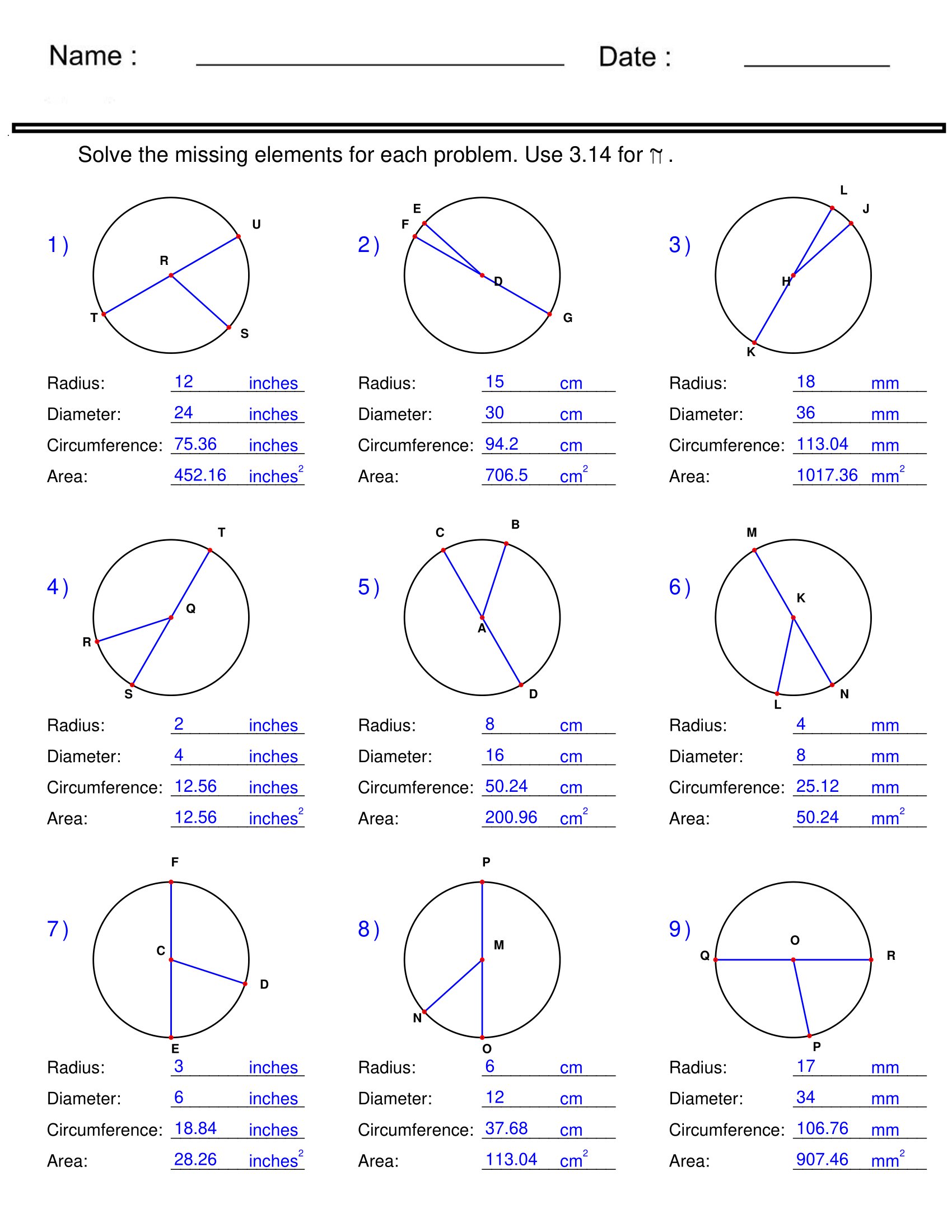
The radius of a circle is the distance from the center of the circle to any point on the circumference. It is a fundamental concept in geometry and is used to calculate the area, circumference, and other properties of a circle. The radius is usually denoted by the symbol ‘r’ or ‘R’.

What is Diameter?

The diameter of a circle is the distance across the circle, passing through its center. It is twice the length of the radius and is denoted by the symbol ’d’ or ’D’. The diameter is the longest chord of a circle and is used to calculate the circumference and other properties of a circle.

Relationship between Radius and Diameter

The radius and diameter of a circle are related by a simple formula:

Diameter (d) = 2 × Radius ®

or

Radius ® = Diameter (d) / 2

This relationship is essential to understand and will help you solve various problems involving radius and diameter.

Key Concepts and Formulas

Here are some key concepts and formulas related to radius and diameter:

• Circumference of a Circle: The circumference of a circle is given by the formula: C = 2πr or C = πd
• Area of a Circle: The area of a circle is given by the formula: A = πr^2
• Relationship between Radius and Diameter: d = 2r or r = d/2

Worksheet: Radius and Diameter

Now, let’s practice what we’ve learned with a worksheet. Solve the following problems and check your answers:

Section 1: Multiple Choice Questions

1. What is the radius of a circle with a diameter of 14 cm? a) 7 cm b) 10 cm c) 12 cm d) 16 cm

Answer: a) 7 cm

1. What is the diameter of a circle with a radius of 9 cm? a) 18 cm b) 16 cm c) 14 cm d) 12 cm

Answer: a) 18 cm

1. What is the circumference of a circle with a radius of 5 cm? a) 20π cm b) 30π cm c) 40π cm d) 50π cm

Answer: b) 30π cm

Section 2: Short Answer Questions

1. If the diameter of a circle is 20 cm, find its radius.

Answer: 10 cm

1. If the radius of a circle is 8 cm, find its diameter.

Answer: 16 cm

1. If the circumference of a circle is 40π cm, find its radius.

Answer: 20 cm

Section 3: Word Problems

1. A bicycle wheel has a diameter of 26 inches. Find its radius.

Answer: 13 inches

1. A circle has a circumference of 60π cm. Find its diameter.

Answer: 30 cm

1. A circular garden has a radius of 15 meters. Find its diameter.

Answer: 30 meters

Notes

• Always check your units when solving problems involving radius and diameter.
• Make sure to use the correct formulas and relationships between radius and diameter.
• Practice, practice, practice! The more you practice, the better you’ll become at solving problems involving radius and diameter.

In this article, we’ve explored the concepts of radius and diameter, and provided a worksheet to help you practice and reinforce your knowledge. Remember to check your units, use the correct formulas, and practice regularly to become proficient in solving problems involving radius and diameter.

Without adding a conclusion header, here’s a summary of the key points:

• Radius is the distance from the center of a circle to any point on the circumference.
• Diameter is the distance across a circle, passing through its center.
• The relationship between radius and diameter is given by the formula: d = 2r or r = d/2.
• Circumference and area of a circle can be calculated using the formulas: C = 2πr or C = πd, and A = πr^2.