Mastering Radical Operations with Ease Worksheet

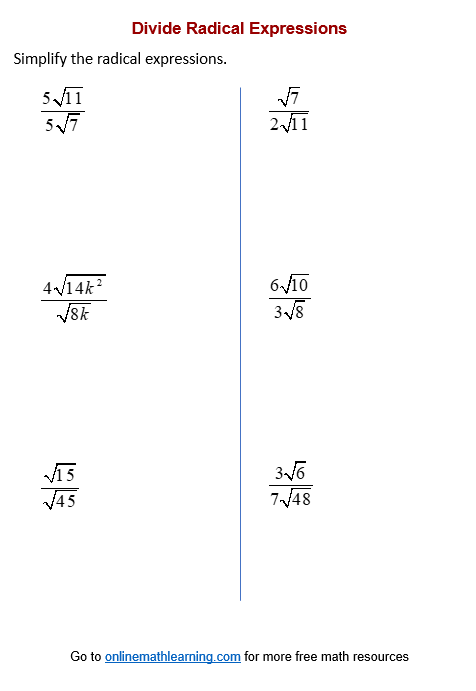
Unlocking the Power of Radical Operations

Radical operations are a fundamental concept in mathematics, and mastering them is crucial for success in various fields, including algebra, geometry, and calculus. However, many students struggle to grasp these concepts, leading to frustration and confusion. In this comprehensive guide, we will break down the basics of radical operations, provide step-by-step examples, and offer practical tips to help you become a pro in no time.

What are Radical Operations?

Radical operations involve working with square roots, cube roots, and other roots. These operations are used to simplify expressions, solve equations, and manipulate algebraic expressions. The most common radical operations include:

• Square Roots: finding the number that, when multiplied by itself, gives a specified value. For example, √16 = 4, because 4 × 4 = 16.
• Cube Roots: finding the number that, when multiplied by itself twice, gives a specified value. For example, ³√27 = 3, because 3 × 3 × 3 = 27.
• Nth Roots: finding the number that, when multiplied by itself n times, gives a specified value. For example, ⁵√32 = 2, because 2 × 2 × 2 × 2 × 2 = 32.

Basic Radical Operations

Now that we’ve covered the basics, let’s dive into the most common radical operations:

Multiplication and Division

When multiplying or dividing radicals, we can combine the operations using the following rules:

• Multiplication: √a × √b = √(a × b)
• Division: √a ÷ √b = √(a ÷ b)

Example:

√12 × √3 = √(12 × 3) = √36 = 6

Addition and Subtraction

When adding or subtracting radicals, we can only combine like radicals (i.e., radicals with the same index).

• Addition: √a + √b = √(a + b)
• Subtraction: √a - √b = √(a - b)

Example:

√12 + √3 = √(12 + 3) = √15

Simplifying Radicals

Simplifying radicals involves reducing the expression to its simplest form. We can do this by factoring out perfect squares or perfect cubes.

Example:

√48 = √(16 × 3) = √16 × √3 = 4√3

Advanced Radical Operations

Now that we’ve covered the basics, let’s move on to more advanced radical operations:

Rationalizing the Denominator

When working with rational expressions, we often need to rationalize the denominator. This involves multiplying the numerator and denominator by the conjugate of the denominator.

Example:

1 / √2 = (1 × √2) / (√2 × √2) = √2 / 2

Simplifying Complex Radicals

Complex radicals involve working with radicals that have a negative sign or a fraction under the radical sign.

Example:

√(-16) = √(-1) × √16 = 4i (where i is the imaginary unit)

Practical Tips and Tricks

Here are some practical tips and tricks to help you master radical operations:

• Use flashcards: Create flashcards to help you memorize the basic radical operations.
• Practice, practice, practice: Practice simplifying radicals and performing radical operations to become more comfortable with the concepts.
• Use online resources: Utilize online resources, such as math apps and websites, to supplement your learning.
• Watch video tutorials: Watch video tutorials to visualize the concepts and get a better understanding of radical operations.

📝 Note: Radical operations can be challenging, but with practice and patience, you can become a pro in no time.

Common Mistakes to Avoid

Here are some common mistakes to avoid when working with radical operations:

• Incorrectly combining radicals: Make sure to only combine like radicals.
• Forgetting to simplify: Always simplify radicals to their simplest form.
• Misapplying rules: Make sure to apply the correct rules for multiplication, division, addition, and subtraction.

Real-World Applications

Radical operations have numerous real-world applications, including:

• Physics: radical operations are used to calculate distances, velocities, and accelerations.
• Engineering: radical operations are used to design and optimize systems, such as bridges and electronic circuits.
• Computer Science: radical operations are used in algorithms and data structures.

Conclusion

Mastering radical operations takes time and practice, but with the right resources and mindset, you can become a pro in no time. Remember to practice regularly, use online resources, and watch video tutorials to supplement your learning. With persistence and dedication, you’ll be solving radical equations and simplifying complex radicals like a pro.

Related Terms:

• Multiplying and Dividing radicals Worksheet
• Multiplying Radicals Worksheet PDF