Pythagorean Theorem Worksheet With Answer Key
Understanding the Pythagorean Theorem
The Pythagorean Theorem is a fundamental concept in geometry, which is used to calculate the length of the hypotenuse of a right-angled triangle. The theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. The formula for the Pythagorean Theorem is:
a² + b² = c²
where a and b are the lengths of the two sides that form the right angle, and c is the length of the hypotenuse.
Applying the Pythagorean Theorem
To apply the Pythagorean Theorem, you need to follow these steps:
- Identify the lengths of the two sides that form the right angle.
- Square the lengths of these two sides.
- Add the squared lengths together.
- Take the square root of the result to find the length of the hypotenuse.
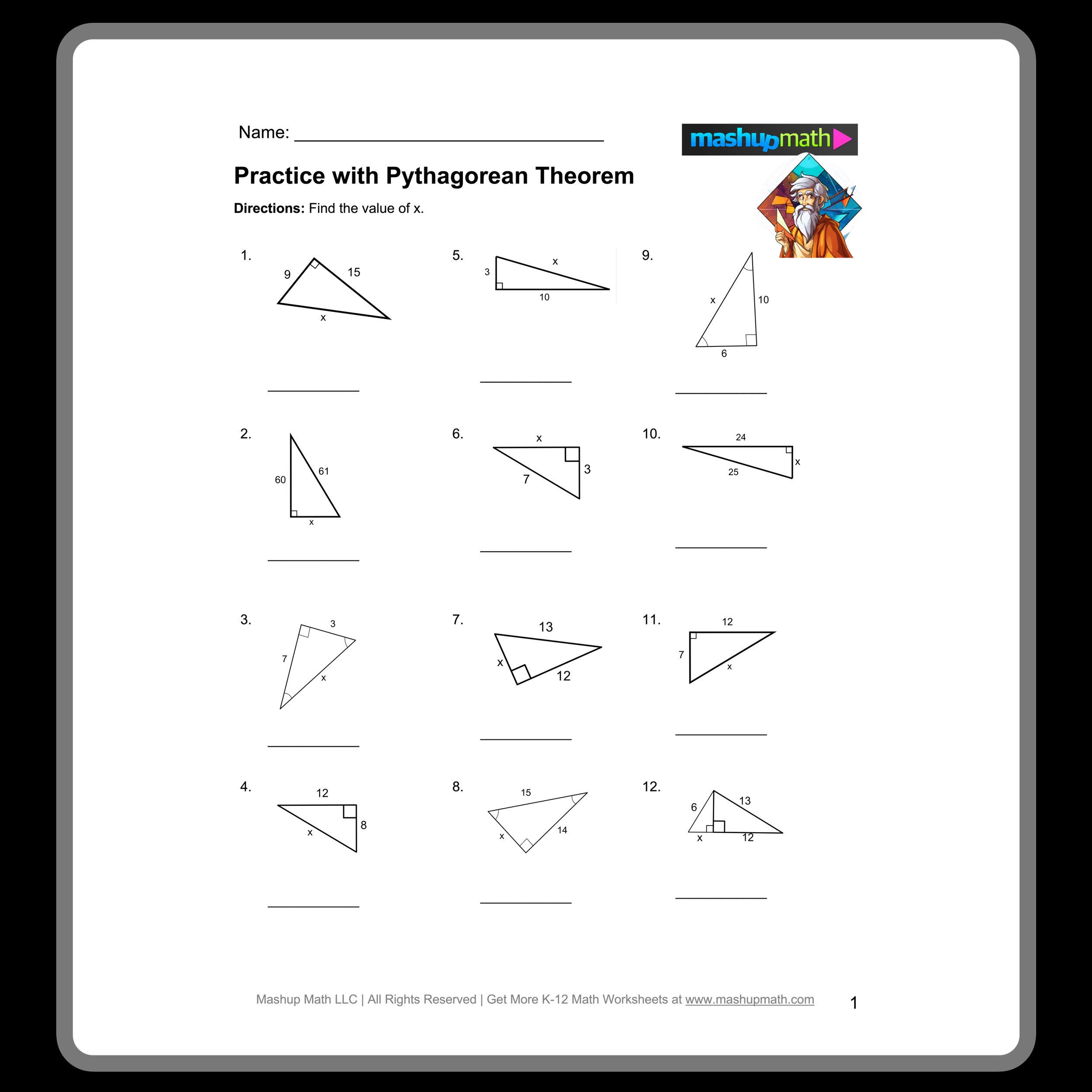
Pythagorean Theorem Worksheet
Here are some examples to help you practice applying the Pythagorean Theorem:
Example 1 In a right-angled triangle, the length of one side is 3 inches and the length of the other side is 4 inches. What is the length of the hypotenuse?
| Side 1 | Side 2 | Hypotenuse |
|---|---|---|
| 3 | 4 | ? |
Example 2 In a right-angled triangle, the length of the hypotenuse is 10 inches and the length of one side is 6 inches. What is the length of the other side?
| Hypotenuse | Side 1 | Side 2 |
|---|---|---|
| 10 | 6 | ? |
Example 3 In a right-angled triangle, the length of one side is 5 inches and the length of the other side is 12 inches. What is the length of the hypotenuse?
| Side 1 | Side 2 | Hypotenuse |
|---|---|---|
| 5 | 12 | ? |
Answer Key
Example 1 Using the Pythagorean Theorem formula:
a² + b² = c² 3² + 4² = c² 9 + 16 = c² 25 = c² c = √25 c = 5 inches
Example 2 Using the Pythagorean Theorem formula:
a² + b² = c² 6² + b² = 10² 36 + b² = 100 b² = 100 - 36 b² = 64 b = √64 b = 8 inches
Example 3 Using the Pythagorean Theorem formula:
a² + b² = c² 5² + 12² = c² 25 + 144 = c² 169 = c² c = √169 c = 13 inches
📝 Note: You can use a calculator to check your answers and make sure you understand the concept.
Real-World Applications of the Pythagorean Theorem
The Pythagorean Theorem has many real-world applications, such as:
- Building design: Architects use the theorem to calculate the height of buildings and the length of shadows.
- Physics: Physicists use the theorem to calculate distances and velocities of objects in motion.
- Engineering: Engineers use the theorem to calculate the stress and strain on materials.
- Navigation: Pilots and sailors use the theorem to calculate distances and directions.
Conclusion
The Pythagorean Theorem is a powerful tool for calculating the length of the hypotenuse of a right-angled triangle. By understanding the formula and applying it to different scenarios, you can solve a wide range of problems in mathematics, science, and engineering. With practice and patience, you can become proficient in using the Pythagorean Theorem to solve complex problems.
What is the Pythagorean Theorem?
+The Pythagorean Theorem is a mathematical formula that calculates the length of the hypotenuse of a right-angled triangle.
How do I apply the Pythagorean Theorem?
+To apply the Pythagorean Theorem, you need to identify the lengths of the two sides that form the right angle, square the lengths, add the squared lengths together, and take the square root of the result.
What are some real-world applications of the Pythagorean Theorem?
+The Pythagorean Theorem has many real-world applications, such as building design, physics, engineering, and navigation.
Related Terms:
- Pythagorean Theorem Worksheet pdf
- Pythagorean Theorem review Worksheet