7 Ways to Apply the Pythagorean Theorem
The Pythagorean Theorem is a fundamental concept in geometry and trigonometry, and its applications extend far beyond the classroom. In this article, we will explore 7 ways to apply the Pythagorean Theorem in real-life scenarios.
What is the Pythagorean Theorem?
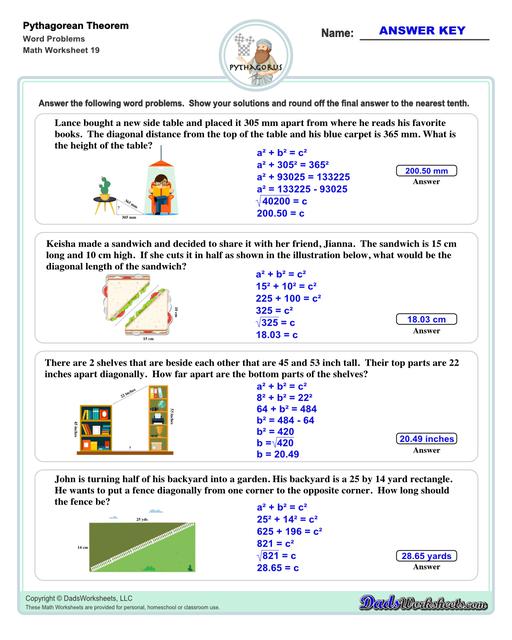
The Pythagorean Theorem is a mathematical concept that describes the relationship between the lengths of the sides of a right-angled triangle. The theorem states that the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. This can be expressed mathematically as:
a² + b² = c²
where a and b are the lengths of the two sides, and c is the length of the hypotenuse.
7 Ways to Apply the Pythagorean Theorem
1. Building Design and Construction
Architects and builders use the Pythagorean Theorem to calculate the height of buildings, bridges, and other structures. By measuring the length of the base and the height of the structure, they can use the theorem to calculate the length of the diagonal support beams.
2. Physics and Engineering
Physicists and engineers use the Pythagorean Theorem to calculate distances, velocities, and forces in complex systems. For example, when calculating the trajectory of a projectile, they can use the theorem to determine the distance traveled by the projectile.
3. Navigation and Surveying
Navigators and surveyors use the Pythagorean Theorem to calculate distances and angles between landmarks. By measuring the distance between two points and the angle between them, they can use the theorem to calculate the distance to a third point.
4. Computer Graphics and Game Development
Computer graphics and game developers use the Pythagorean Theorem to create 3D models and simulate real-world physics. By calculating the distances and angles between objects, they can create realistic animations and interactions.
5. Medical Imaging and Diagnostics
Medical professionals use the Pythagorean Theorem to calculate distances and angles in medical imaging and diagnostics. For example, when calculating the distance between a tumor and a surrounding organ, they can use the theorem to determine the best course of treatment.
6. Astronomy and Space Exploration
Astronomers and space explorers use the Pythagorean Theorem to calculate distances and trajectories in space. By measuring the distance between celestial bodies and their velocities, they can use the theorem to determine the best course for space travel.
7. GPS and Mapping
GPS and mapping systems use the Pythagorean Theorem to calculate distances and angles between locations. By measuring the distance between satellites and receivers, they can use the theorem to determine the user’s location and provide accurate directions.
📝 Note: These are just a few examples of the many ways the Pythagorean Theorem is applied in real-life scenarios. The theorem is a fundamental concept that has far-reaching implications in various fields.
How to Use the Pythagorean Theorem in Real-Life Scenarios
To use the Pythagorean Theorem in real-life scenarios, follow these steps:
- Identify the right-angled triangle in the problem.
- Measure the lengths of the two sides (a and b).
- Use the theorem to calculate the length of the hypotenuse ©.
- Apply the calculated value to the problem.
By following these steps, you can apply the Pythagorean Theorem to a wide range of problems and scenarios.
What is the Pythagorean Theorem?
+The Pythagorean Theorem is a mathematical concept that describes the relationship between the lengths of the sides of a right-angled triangle.
How is the Pythagorean Theorem used in real-life scenarios?
+The Pythagorean Theorem is used in various fields such as building design, physics, navigation, computer graphics, medical imaging, astronomy, and GPS and mapping.
How do I apply the Pythagorean Theorem to a problem?
+Identify the right-angled triangle, measure the lengths of the two sides, use the theorem to calculate the length of the hypotenuse, and apply the calculated value to the problem.
By understanding and applying the Pythagorean Theorem, you can solve a wide range of problems and make informed decisions in various fields. Remember to identify the right-angled triangle, measure the lengths of the two sides, use the theorem to calculate the length of the hypotenuse, and apply the calculated value to the problem.
Related Terms:
- Pythagorean Theorem Worksheet pdf
- Pythagoras Word Problems worksheet pdf