Division Made Easy for 5th Graders
Understanding Division: A Guide for 5th Graders
Division is a fundamental concept in mathematics that can be a bit tricky to grasp at first, but with practice and the right approach, it can become a breeze. In this article, we will break down the concept of division in a way that is easy to understand for 5th graders.
What is Division?
Division is the process of sharing a certain number of items into equal groups or parts. It is the opposite of multiplication, where we add groups together. Think of division like distributing cookies among your friends. If you have 12 cookies and you want to share them equally among 4 of your friends, how many cookies will each friend get?
Division Symbol
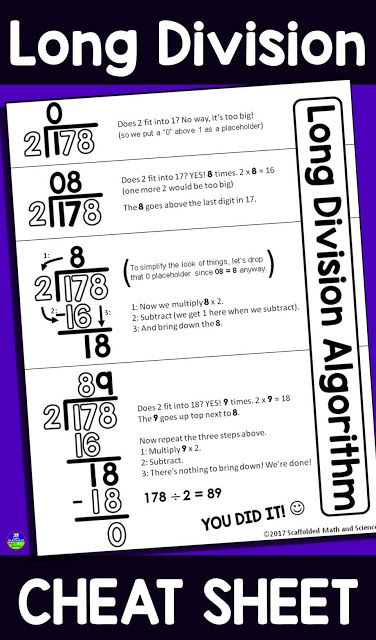
The division symbol is ÷. It is used to indicate that we are dividing one number by another. For example, 12 ÷ 4 = 3. This means that 12 cookies divided among 4 friends will give each friend 3 cookies.
Key Concepts in Division
There are several key concepts that you need to understand when it comes to division:
- Dividend: The number being divided. In our cookie example, 12 is the dividend.
- Divisor: The number by which we are dividing. In our example, 4 is the divisor.
- Quotient: The result of the division. In our example, 3 is the quotient.
- Remainder: The amount left over after dividing. If we divide 12 cookies among 4 friends, each friend will get 3 cookies, and there will be no remainder.
Types of Division
There are two main types of division:
- Exact Division: When the dividend can be divided evenly by the divisor, with no remainder. For example, 12 ÷ 4 = 3.
- Inexact Division: When the dividend cannot be divided evenly by the divisor, resulting in a remainder. For example, 13 ÷ 4 = 3 with a remainder of 1.
Division Strategies
Here are some strategies to help you solve division problems:
- Repeated Subtraction: Subtract the divisor from the dividend repeatedly until you get a quotient. For example, 12 - 4 - 4 - 4 = 0, so 12 ÷ 4 = 3.
- Arrays: Draw an array of dots or blocks to represent the dividend. Divide the array into equal groups to find the quotient. For example, if you have 12 dots, you can divide them into 4 groups of 3 dots each.
- Base-Ten Blocks: Use base-ten blocks to represent the dividend. Divide the blocks into equal groups to find the quotient.
Real-World Applications of Division
Division is used in many real-world situations, such as:
- Sharing: Dividing toys or candies among friends.
- Cooking: Measuring ingredients for a recipe.
- Shopping: Dividing a bill among friends.
- Science: Measuring the size of objects or the distance between them.
Practice Makes Perfect
Practice is key to mastering division. Try solving these division problems on your own:
- 18 ÷ 3 =
- 24 ÷ 6 =
- 9 ÷ 3 =
💡 Note: You can use the strategies mentioned above to help you solve these problems.
Conclusion
Division may seem daunting at first, but with practice and the right approach, it can become easy. Remember to understand the key concepts, use division strategies, and practice solving problems. With time and effort, you will become a division master!
What is the difference between exact and inexact division?
+Exact division is when the dividend can be divided evenly by the divisor, with no remainder. Inexact division is when the dividend cannot be divided evenly by the divisor, resulting in a remainder.
How can I use real-world applications to help me understand division?
+Think about how division is used in your everyday life, such as sharing toys or candies among friends, measuring ingredients for a recipe, or dividing a bill among friends.
What is the dividend, divisor, quotient, and remainder in a division problem?
+The dividend is the number being divided, the divisor is the number by which we are dividing, the quotient is the result of the division, and the remainder is the amount left over after dividing.