Proportional Graphs Worksheet for Math Mastery

Unlocking the Secrets of Proportional Graphs
Proportional graphs are a fundamental concept in mathematics, and mastering them is crucial for solving problems in various fields, including science, engineering, and economics. In this article, we will delve into the world of proportional graphs, exploring their definition, types, and applications. We will also provide a comprehensive worksheet to help you practice and reinforce your understanding of proportional graphs.
What are Proportional Graphs?
A proportional graph is a graphical representation of a proportional relationship between two variables. In a proportional relationship, the ratio of the two variables remains constant, even as the values of the variables change. This means that as one variable increases or decreases, the other variable increases or decreases at a constant rate.
Types of Proportional Graphs
There are two main types of proportional graphs:
- Direct Proportion: In a direct proportion, the ratio of the two variables is constant and positive. This means that as one variable increases, the other variable also increases at a constant rate.
- Inverse Proportion: In an inverse proportion, the ratio of the two variables is constant and negative. This means that as one variable increases, the other variable decreases at a constant rate.
Key Features of Proportional Graphs
Proportional graphs have several key features that distinguish them from other types of graphs:
- Constant Ratio: The ratio of the two variables remains constant, even as the values of the variables change.
- Straight Line: Proportional graphs are always straight lines, either positive or negative.
- No Intercepts: Proportional graphs do not have intercepts, as the line extends infinitely in both directions.
Applications of Proportional Graphs
Proportional graphs have numerous applications in various fields, including:
- Science: Proportional graphs are used to model the relationship between physical quantities, such as the distance traveled by an object and the time taken.
- Engineering: Proportional graphs are used to design and optimize systems, such as electrical circuits and mechanical systems.
- Economics: Proportional graphs are used to model the relationship between economic variables, such as the demand for a product and its price.
Worksheet: Proportional Graphs
Now that we have explored the basics of proportional graphs, it’s time to practice! The following worksheet provides a range of questions to help you reinforce your understanding of proportional graphs.
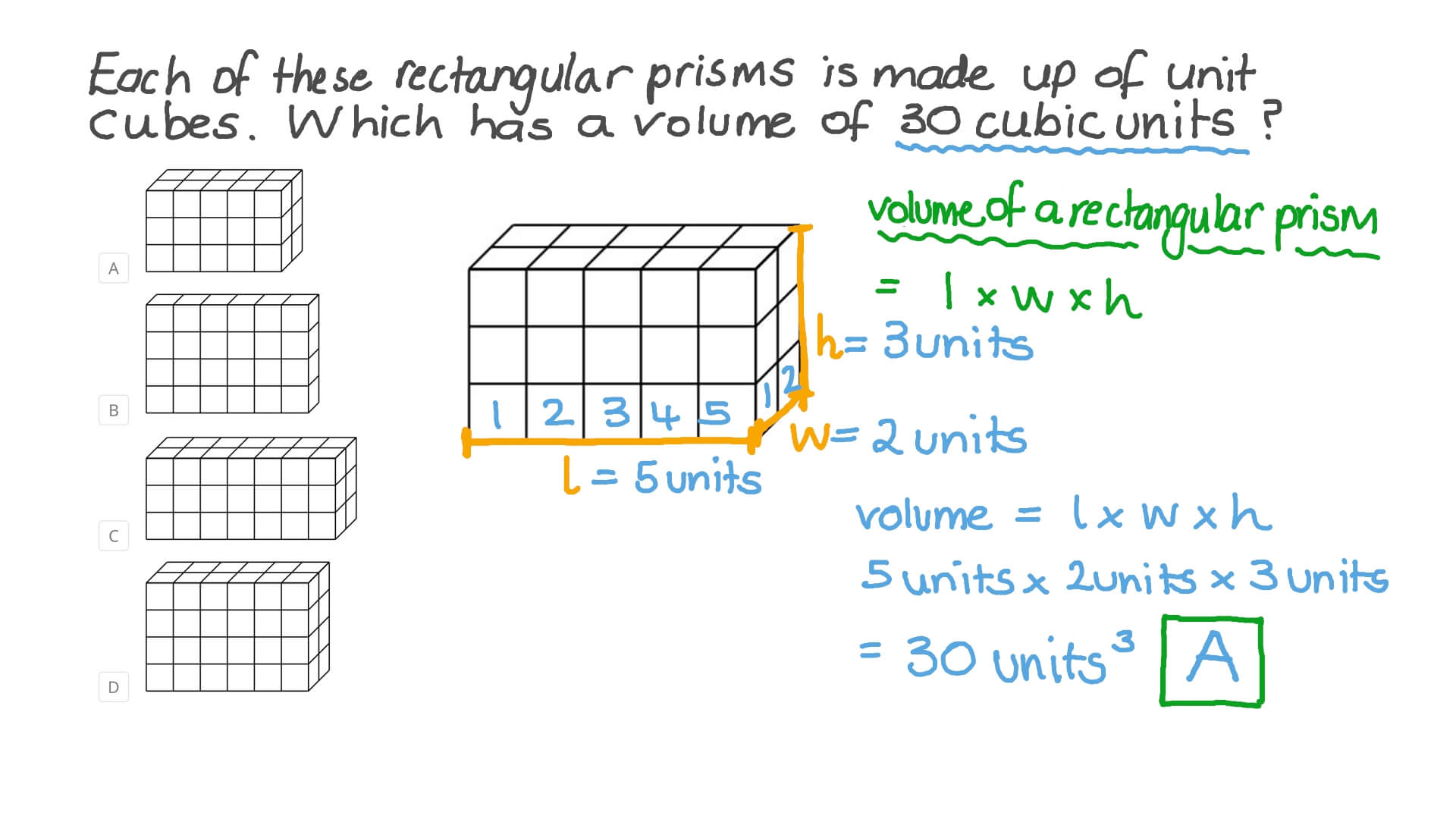
| Question | Problem | Solution |
|---|---|---|
| 1 | A car travels 250 miles in 5 hours. If the car travels at a constant speed, what is the proportional relationship between the distance traveled and the time taken? | Let x be the distance traveled and y be the time taken. The proportional relationship is x = 50y. |
| 2 | A bakery sells 200 loaves of bread at $2 each. If the bakery increases the price of each loaf by 10%, what is the new price of each loaf? | Let x be the original price and y be the new price. The proportional relationship is y = 1.1x. Therefore, the new price of each loaf is $2.20. |
| 3 | A company produces 1000 units of a product in 10 hours. If the company increases production by 20%, what is the new number of units produced? | Let x be the original number of units produced and y be the new number of units produced. The proportional relationship is y = 1.2x. Therefore, the new number of units produced is 1200. |
| 4 | A person invests $1000 in a savings account that earns 5% interest per year. If the interest rate remains constant, what is the proportional relationship between the amount invested and the interest earned? | Let x be the amount invested and y be the interest earned. The proportional relationship is y = 0.05x. |
| 5 | A water tank can hold 1000 liters of water. If 200 liters of water are added to the tank every hour, what is the proportional relationship between the amount of water added and the time taken? | Let x be the amount of water added and y be the time taken. The proportional relationship is x = 200y. |
📝 Note: The solutions to the worksheet questions are provided in the table above. Make sure to work through each problem carefully and check your answers against the solutions.
What is the difference between a direct proportion and an inverse proportion?
+A direct proportion is a relationship where the ratio of the two variables is constant and positive. An inverse proportion is a relationship where the ratio of the two variables is constant and negative.
How do I identify a proportional graph?
+A proportional graph is a straight line that passes through the origin (0, 0). The ratio of the two variables remains constant, even as the values of the variables change.
What are some real-world applications of proportional graphs?
+Proportional graphs have numerous applications in various fields, including science, engineering, and economics. They are used to model the relationship between physical quantities, design and optimize systems, and analyze economic data.
In conclusion, proportional graphs are a fundamental concept in mathematics that have numerous applications in various fields. By understanding the definition, types, and applications of proportional graphs, you can develop a strong foundation in math and problem-solving skills. Practice the worksheet questions and review the FAQs to reinforce your understanding of proportional graphs.
Related Terms:
- teacher synergy llc
- IXL
- Khan Academy
- BrainPOP
- Udacity
- Duolingo