Probability of Compound Events Made Easy

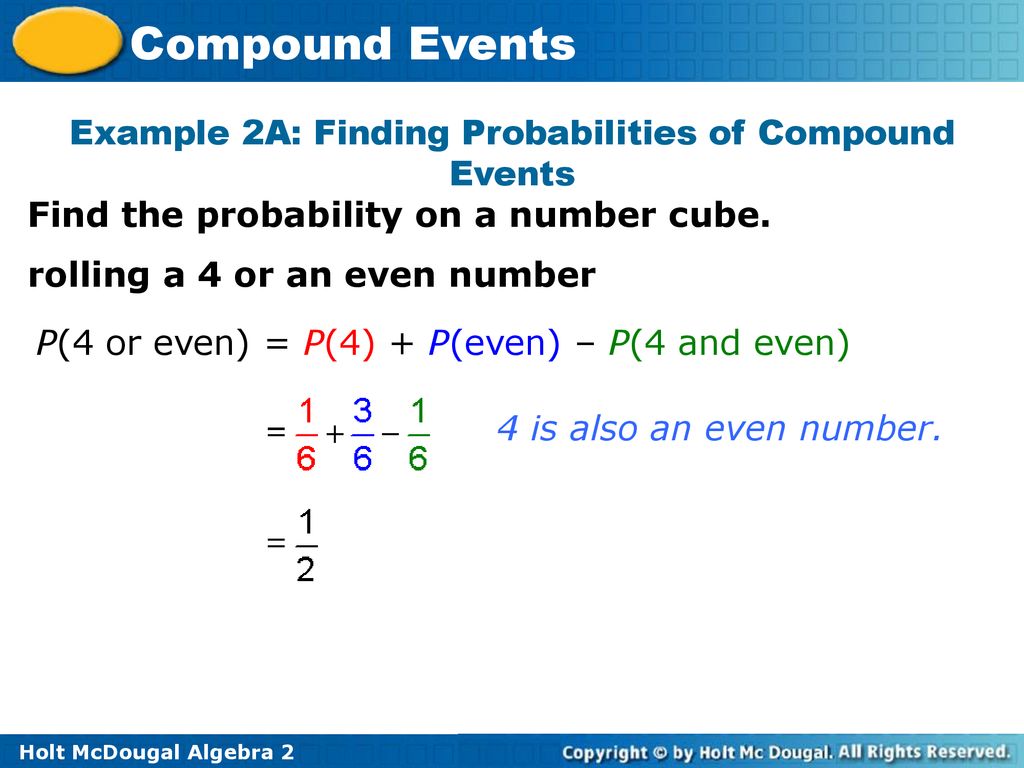
Understanding Compound Events in Probability

When it comes to probability, understanding compound events is crucial. Compound events occur when two or more events happen together, and calculating their probabilities can be a bit more complex than calculating the probability of a single event. In this article, we’ll break down the concept of compound events, explore the different types of compound events, and provide examples to help you understand how to calculate their probabilities.

What are Compound Events?

A compound event is an event that consists of two or more events happening together. These events can be independent or dependent, and they can happen simultaneously or in sequence. For example, flipping two coins and getting heads on both is a compound event, as is drawing two cards from a deck and getting a pair.

Types of Compound Events

There are two main types of compound events: independent events and dependent events.

Independent Events

Independent events are events that do not affect each other. The outcome of one event does not influence the outcome of the other event. For example, flipping a coin and rolling a die are independent events. The outcome of the coin flip does not affect the outcome of the die roll.

Dependent Events

Dependent events are events that affect each other. The outcome of one event influences the outcome of the other event. For example, drawing a card from a deck and then drawing another card without replacing the first card are dependent events. The outcome of the first draw affects the outcome of the second draw.

Calculating Probabilities of Compound Events

Calculating the probability of a compound event involves multiplying the probabilities of the individual events. The formula for calculating the probability of a compound event is:

P(A and B) = P(A) x P(B)

Where:

• P(A) is the probability of event A
• P(B) is the probability of event B
• P(A and B) is the probability of both events A and B occurring

For example, let’s say we want to calculate the probability of getting heads on both flips of a coin. The probability of getting heads on one flip is ¹⁄₂, so the probability of getting heads on both flips is:

P(Heads and Heads) = P(Heads) x P(Heads) = ¹⁄₂ x ¹⁄₂ = ¹⁄₄

🤔 Note: When calculating the probability of a compound event, make sure to multiply the probabilities of the individual events, not add them.

Examples of Compound Events

Here are a few more examples of compound events:

• Flipping a coin and rolling a die, and getting heads on the coin and a 6 on the die.
• Drawing two cards from a deck, and getting a pair of aces.
• Tossing a fair six-sided die twice, and getting a 3 on the first toss and a 4 on the second toss.

For each of these examples, we can calculate the probability of the compound event by multiplying the probabilities of the individual events.

Real-World Applications of Compound Events

Understanding compound events has many real-world applications. For example:

• Insurance companies use compound events to calculate the probability of multiple accidents occurring, and to determine insurance premiums.
• Medical researchers use compound events to calculate the probability of multiple genetic factors contributing to a disease.
• Financial analysts use compound events to calculate the probability of multiple economic factors affecting stock prices.

Conclusion

Compound events are an important concept in probability, and understanding how to calculate their probabilities is crucial in many real-world applications. By multiplying the probabilities of individual events, we can calculate the probability of a compound event. Remember to always multiply the probabilities, not add them, and to consider whether the events are independent or dependent.