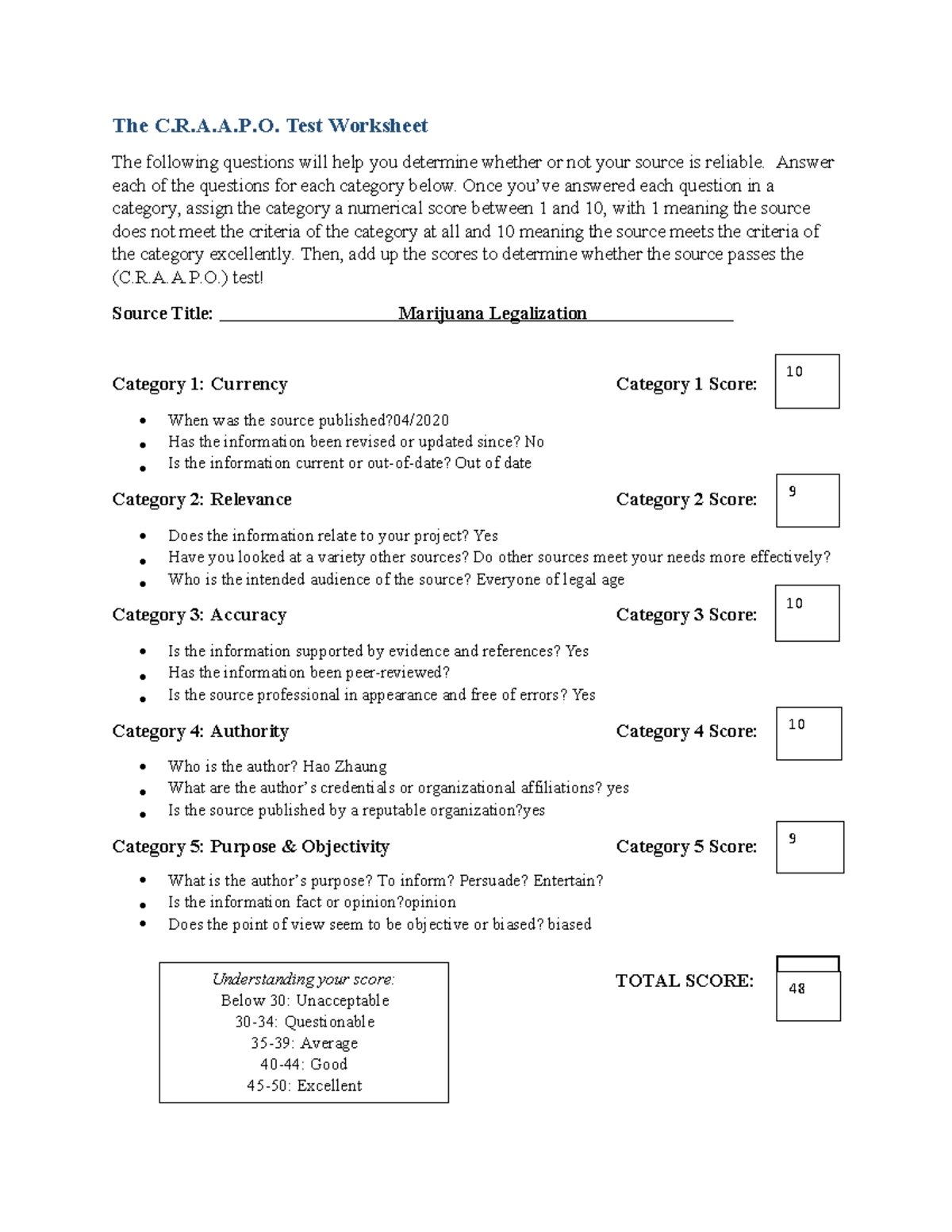
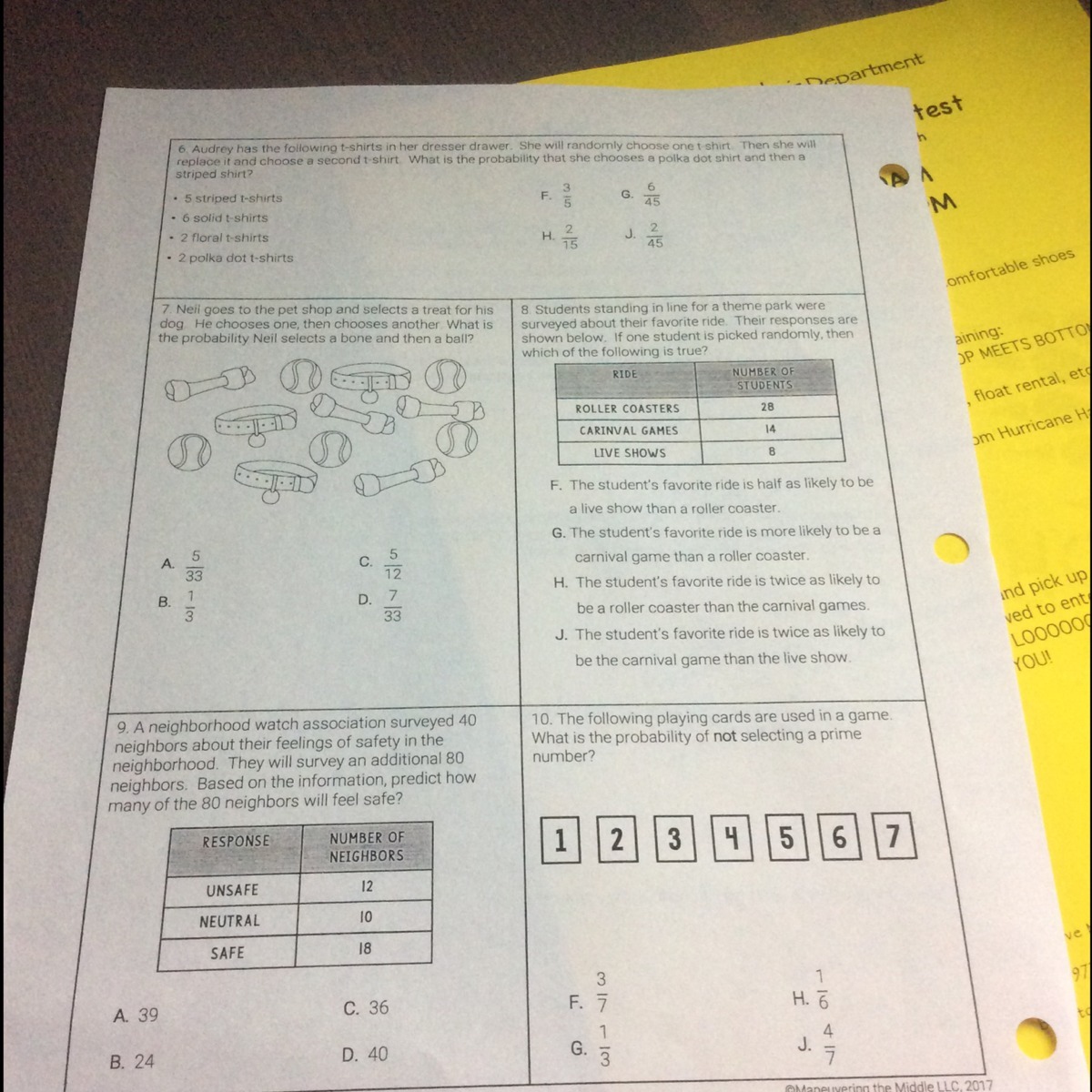
6 Steps to Master Dividing Polynomials

Dividing Polynomials: A Comprehensive Guide
Polynomial division is a fundamental concept in algebra that can seem intimidating at first, but with practice and patience, it can become a manageable task. In this article, we will break down the process of dividing polynomials into six manageable steps, making it easier for you to master this essential skill.
Step 1: Understand the Basics of Polynomial Division
Polynomial division is the process of dividing a polynomial by another polynomial. It is similar to long division of numbers, but instead of dividing numbers, we are dividing polynomials. The dividend is the polynomial being divided, and the divisor is the polynomial by which we are dividing.
📝 Note: It's essential to understand the terminology used in polynomial division. Familiarize yourself with terms like dividend, divisor, quotient, and remainder.
Step 2: Write the Dividend and Divisor in the Correct Format
To begin the division process, write the dividend and divisor in the correct format. The dividend should be written on top of a line, and the divisor should be written below it. The divisor should be aligned with the term of the dividend that has the highest degree.
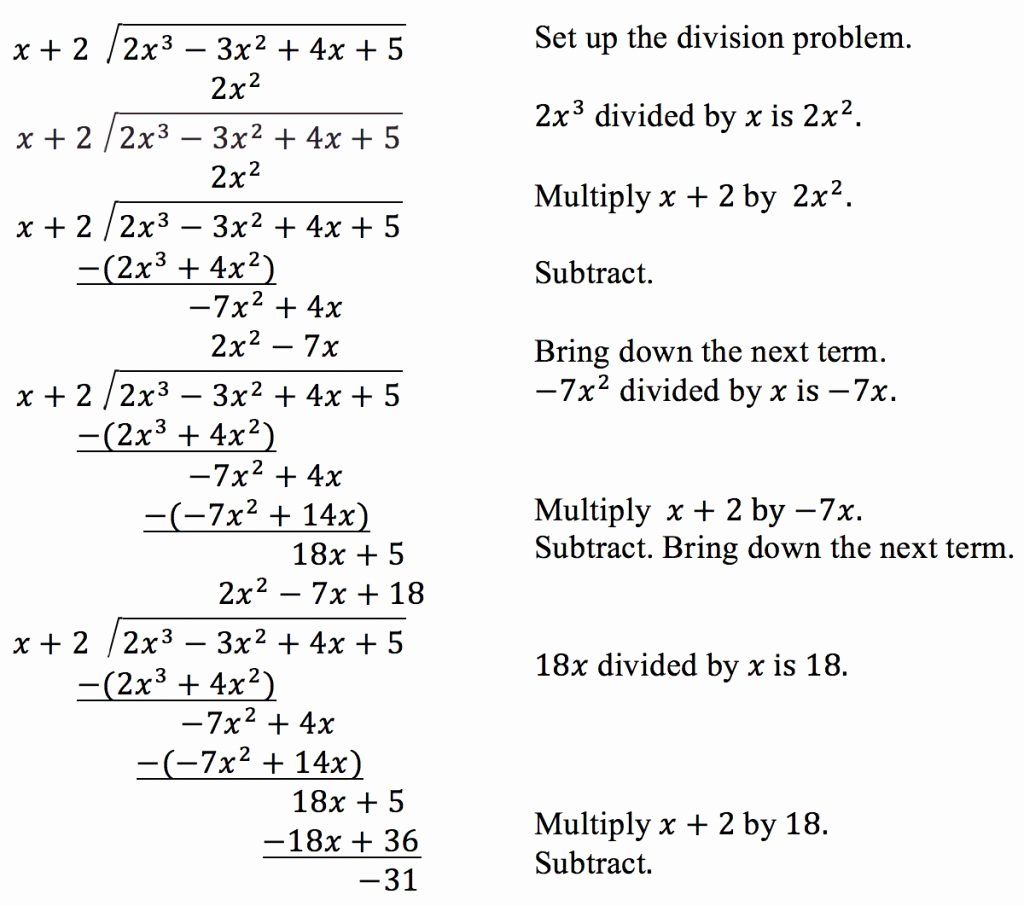
| Dividend | Divisor |
|---|---|
| x^2 + 3x - 4 | x - 2 |
Step 3: Divide the Leading Term of the Dividend by the Leading Term of the Divisor
Divide the leading term of the dividend (x^2) by the leading term of the divisor (x). This will give you the first term of the quotient.
x^2 ÷ x = x
Step 4: Multiply the Divisor by the Quotient Term and Subtract
Multiply the divisor (x - 2) by the quotient term (x), and subtract the result from the dividend.
(x - 2) × x = x^2 - 2x x^2 + 3x - 4 - (x^2 - 2x) = 5x - 4
Step 5: Repeat the Process Until You Reach the Remainder
Repeat the process of dividing the leading term of the remaining polynomial by the leading term of the divisor, multiplying the divisor by the quotient term, and subtracting.
5x - 4 ÷ x = 5 (x - 2) × 5 = 5x - 10 5x - 4 - (5x - 10) = 6
Step 6: Write the Final Quotient and Remainder
The final quotient is x + 5, and the remainder is 6.
x^2 + 3x - 4 = (x - 2)(x + 5) + 6
By following these six steps, you can master the art of dividing polynomials. Remember to practice regularly to build your confidence and fluency.
To recap, the key to dividing polynomials is to follow the correct format, divide the leading terms, multiply and subtract, and repeat the process until you reach the remainder.
What is the main difference between polynomial division and numerical division?
+The main difference is that polynomial division involves dividing polynomials, whereas numerical division involves dividing numbers.
How do I determine the degree of a polynomial?
+The degree of a polynomial is determined by the highest power of the variable in the polynomial. For example, the degree of x^2 + 3x - 4 is 2.
What is the purpose of the remainder in polynomial division?
+The remainder represents the amount left over after the division process is complete. It can be used to check the accuracy of the division.
Related Terms:
- Dividing Polynomials worksheet Algebra 1
- Dividing Polynomials Worksheet PDF
- Dividing polynomials no remainder worksheet