Master Metric Conversions with Our Practice Worksheet
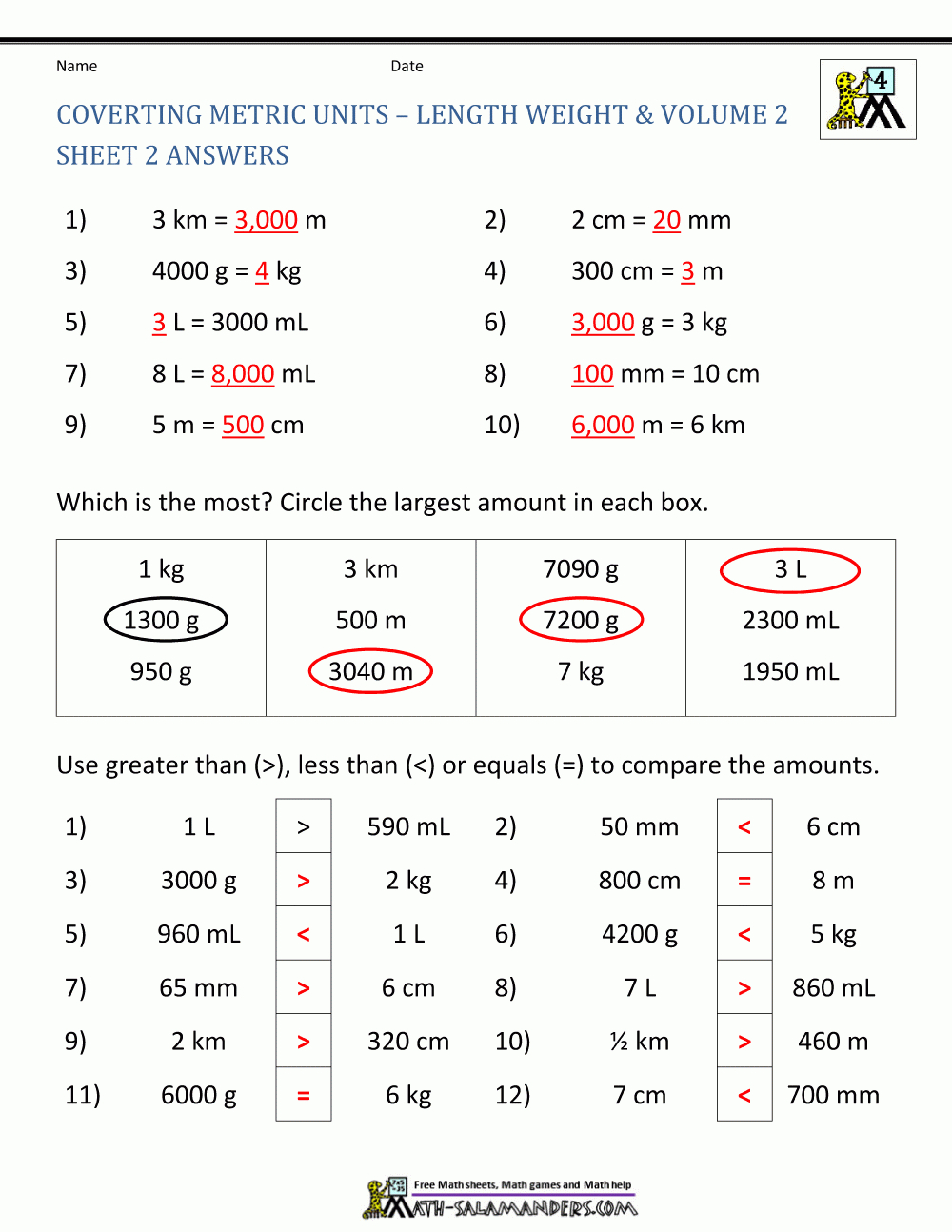
Mastering Metric Conversions: A Step-by-Step Guide
Converting between different units of measurement is a crucial skill in various fields, including science, engineering, and mathematics. The metric system, also known as the International System of Units (SI), is the most widely used system of measurement globally. In this article, we will provide a comprehensive guide on how to master metric conversions using a practice worksheet.
Understanding the Metric System
The metric system is based on the decimal system, with seven fundamental units of measurement:
- Meter (m) - length
- Gram (g) - mass
- Liter (L) - volume
- Second (s) - time
- Kelvin (K) - temperature
- Ampere (A) - electric current
- Mole (mol) - amount of substance
These fundamental units can be combined to form derived units, such as area (square meters), volume (cubic meters), and speed (meters per second).
Basic Conversion Techniques
To master metric conversions, you need to learn the basic conversion techniques. Here are some essential techniques:
- Multiplication and Division: To convert between units, you can multiply or divide by a conversion factor. For example, to convert from meters to kilometers, you can multiply by 0.001 (since there are 1000 meters in 1 kilometer).
- Conversion Charts: You can use conversion charts to quickly look up conversion factors. These charts usually list the units and their corresponding conversion factors.
- Conversion Formulas: You can use formulas to convert between units. For example, to convert from Celsius to Kelvin, you can use the formula: K = °C + 273.15.
Common Metric Conversions
Here are some common metric conversions that you should know:
- Length:
- Meter (m) to kilometer (km): 1 km = 1000 m
- Meter (m) to centimeter (cm): 1 m = 100 cm
- Meter (m) to millimeter (mm): 1 m = 1000 mm
- Mass:
- Gram (g) to kilogram (kg): 1 kg = 1000 g
- Gram (g) to milligram (mg): 1 g = 1000 mg
- Volume:
- Liter (L) to milliliter (mL): 1 L = 1000 mL
- Liter (L) to cubic meter (m³): 1 m³ = 1000 L
- Temperature:
- Celsius (°C) to Kelvin (K): K = °C + 273.15
- Fahrenheit (°F) to Celsius (°C): °C = (°F - 32) × 5⁄9
Practice Worksheet
Here is a practice worksheet to help you master metric conversions:
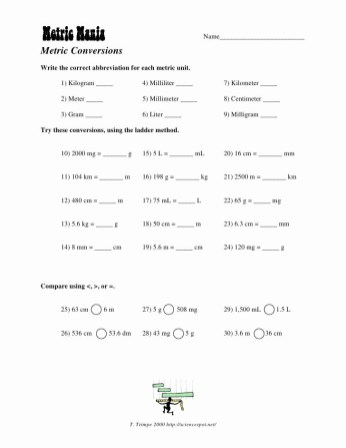
| Conversion | Problem | Answer |
|---|---|---|
| Length: meter to kilometer | 500 m =? km | 0.5 km |
| Mass: gram to kilogram | 250 g =? kg | 0.25 kg |
| Volume: liter to milliliter | 2 L =? mL | 2000 mL |
| Temperature: Celsius to Kelvin | 25 °C =? K | 298.15 K |
| Speed: meters per second to kilometers per hour | 10 m/s =? km/h | 36 km/h |
💡 Note: You can use the conversion formulas and techniques mentioned earlier to solve these problems.
Tips and Tricks
Here are some tips and tricks to help you master metric conversions:
- Practice, practice, practice: Practice converting between units regularly to become more confident and proficient.
- Use conversion charts and formulas: Use conversion charts and formulas to quickly look up conversion factors and perform conversions.
- Check your units: Always check your units to ensure that you are converting between the correct units.
- Use dimensional analysis: Use dimensional analysis to convert between units. This involves canceling out units and multiplying by conversion factors.
In conclusion, mastering metric conversions requires practice, patience, and attention to detail. By following the techniques and tips outlined in this article, you can become proficient in converting between different units of measurement. Remember to practice regularly and use conversion charts and formulas to help you solve problems.
What is the most common unit of measurement in the metric system?
+The most common unit of measurement in the metric system is the meter (m) for length, gram (g) for mass, and liter (L) for volume.
How do I convert between Celsius and Kelvin?
+To convert from Celsius to Kelvin, add 273.15 to the Celsius temperature. To convert from Kelvin to Celsius, subtract 273.15 from the Kelvin temperature.
What is dimensional analysis?
+Dimensional analysis is a technique used to convert between units by canceling out units and multiplying by conversion factors.
Related Terms:
- Metric conversion Worksheet PDF
- Metric Conversion Worksheet high school
- Conversion Practice Worksheet with answers