Polygon Angle Sum Theorem Worksheet
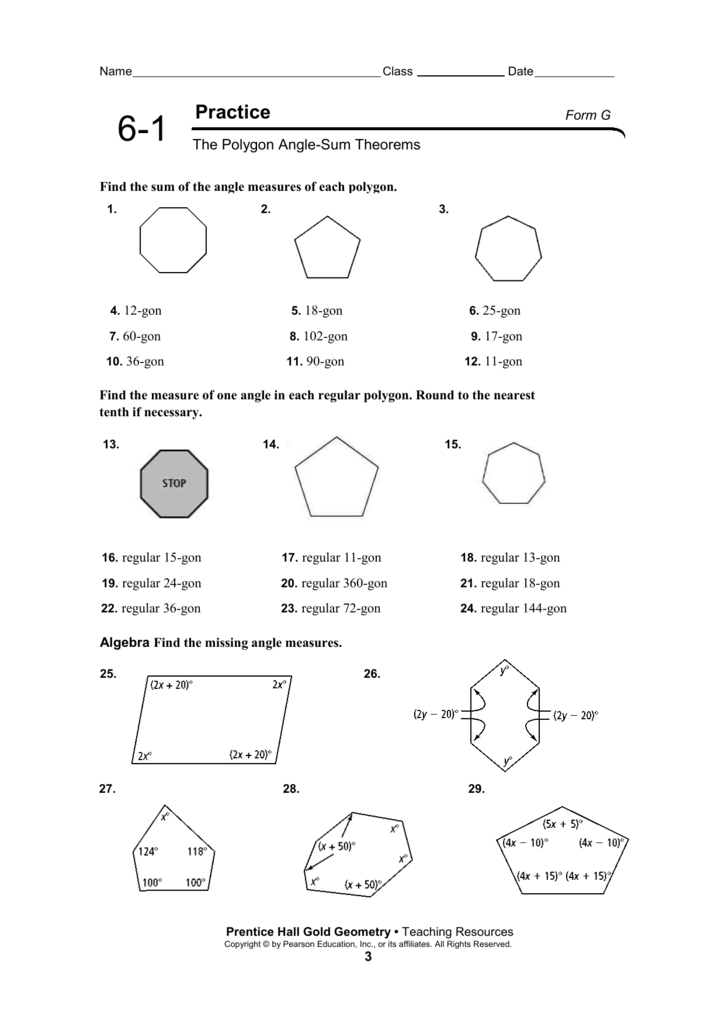
Polygon Angle Sum Theorem: A Comprehensive Guide
The Polygon Angle Sum Theorem is a fundamental concept in geometry that helps us understand the relationship between the interior angles of a polygon. In this article, we will delve into the world of polygons, explore the Polygon Angle Sum Theorem, and provide a worksheet to help you practice and reinforce your understanding of this concept.
What is a Polygon?
A polygon is a two-dimensional shape with at least three sides. It can be convex or concave, and its sides can be equal or unequal in length. Polygons can be classified into different types based on the number of sides they have. For example, a triangle is a polygon with three sides, a quadrilateral is a polygon with four sides, and so on.
What is the Polygon Angle Sum Theorem?
The Polygon Angle Sum Theorem states that the sum of the interior angles of a polygon is equal to (n-2) × 180 degrees, where n is the number of sides of the polygon. This theorem applies to all polygons, regardless of the number of sides they have.
How to Apply the Polygon Angle Sum Theorem
To apply the Polygon Angle Sum Theorem, you need to know the number of sides of the polygon. Once you have this information, you can plug it into the formula (n-2) × 180 degrees to find the sum of the interior angles.
For example, let’s say we have a pentagon, which is a polygon with five sides. To find the sum of the interior angles of the pentagon, we can plug n = 5 into the formula:
(5-2) × 180 degrees = 3 × 180 degrees = 540 degrees
Therefore, the sum of the interior angles of a pentagon is 540 degrees.
Worksheet: Practice Applying the Polygon Angle Sum Theorem
Here’s a worksheet to help you practice applying the Polygon Angle Sum Theorem:

| Polygon | Number of Sides | Sum of Interior Angles |
|---|---|---|
| Triangle | 3 | |
| Quadrilateral | 4 | |
| Pentagon | 5 | |
| Hexagon | 6 | |
| Heptagon | 7 | |
| Octagon | 8 | |
| Nonagon | 9 | |
| Decagon | 10 |
Use the Polygon Angle Sum Theorem to find the sum of the interior angles for each polygon. Write your answers in the table above.
📝 Note: You can use the formula (n-2) × 180 degrees to find the sum of the interior angles for each polygon.
Solutions to the Worksheet
Here are the solutions to the worksheet:
| Polygon | Number of Sides | Sum of Interior Angles |
|---|---|---|
| Triangle | 3 | (3-2) × 180 degrees = 180 degrees |
| Quadrilateral | 4 | (4-2) × 180 degrees = 360 degrees |
| Pentagon | 5 | (5-2) × 180 degrees = 540 degrees |
| Hexagon | 6 | (6-2) × 180 degrees = 720 degrees |
| Heptagon | 7 | (7-2) × 180 degrees = 900 degrees |
| Octagon | 8 | (8-2) × 180 degrees = 1080 degrees |
| Nonagon | 9 | (9-2) × 180 degrees = 1260 degrees |
| Decagon | 10 | (10-2) × 180 degrees = 1440 degrees |
In Conclusion
In this article, we explored the Polygon Angle Sum Theorem and its application in finding the sum of the interior angles of a polygon. We also provided a worksheet to help you practice and reinforce your understanding of this concept. Remember, the Polygon Angle Sum Theorem is a powerful tool that can help you solve a wide range of geometry problems.
What is the Polygon Angle Sum Theorem?
+The Polygon Angle Sum Theorem states that the sum of the interior angles of a polygon is equal to (n-2) × 180 degrees, where n is the number of sides of the polygon.
How do I apply the Polygon Angle Sum Theorem?
+To apply the Polygon Angle Sum Theorem, you need to know the number of sides of the polygon. Once you have this information, you can plug it into the formula (n-2) × 180 degrees to find the sum of the interior angles.
What is a polygon?
+A polygon is a two-dimensional shape with at least three sides. It can be convex or concave, and its sides can be equal or unequal in length.
Related Terms:
- Exterior angles of polygons worksheet
- Interior angle of polygon
- Polygon exercise pdf
- Polygon geometry
- Angles Relationships worksheet pdf