Perpendicular and Angle Bisectors Worksheet Answers
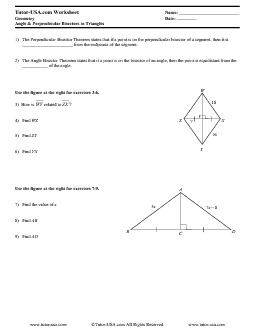
Perpendicular and Angle Bisectors: A Comprehensive Review
In geometry, perpendicular lines and angle bisectors are two fundamental concepts that are crucial in understanding various geometric shapes and properties. In this blog post, we will delve into the world of perpendicular lines and angle bisectors, discussing their definitions, properties, and applications. We will also provide a worksheet with answers to help reinforce your understanding of these concepts.
Perpendicular Lines
Perpendicular lines are two lines that intersect at a 90-degree angle. This means that the angle formed by the intersection of the two lines is a right angle. Perpendicular lines have several key properties:
- Slope: The slope of perpendicular lines are negative reciprocals of each other. This means that if the slope of one line is m, the slope of the other line is -1/m.
- Intersection: Perpendicular lines always intersect at a single point, unless they are parallel.
- Angle: The angle formed by the intersection of perpendicular lines is always 90 degrees.
Angle Bisectors
An angle bisector is a line that divides an angle into two equal parts. Angle bisectors have several key properties:
- Division: An angle bisector divides an angle into two equal parts, each measuring half the original angle.
- Intersection: An angle bisector intersects the opposite side of the angle at a single point.
- Concurrency: The angle bisectors of a triangle are concurrent, meaning they intersect at a single point.
Applications of Perpendicular Lines and Angle Bisectors
Perpendicular lines and angle bisectors have numerous applications in geometry and real-world problems. Some of these applications include:
- Geometry: Perpendicular lines and angle bisectors are used to construct various geometric shapes, such as squares, rectangles, and triangles.
- Trigonometry: Perpendicular lines and angle bisectors are used to solve trigonometric problems, such as finding the length of a side of a triangle.
- Physics: Perpendicular lines and angle bisectors are used to describe the motion of objects in physics, such as the path of a projectile.
Worksheet: Perpendicular Lines and Angle Bisectors
Now that we have reviewed the concepts of perpendicular lines and angle bisectors, it’s time to practice! Here is a worksheet with 10 problems to help reinforce your understanding of these concepts.

| Problem | Answer |
|---|---|
| 1. Find the slope of a line perpendicular to the line 2x - 3y = 5. | 3/2 |
| 2. Find the equation of a line that bisects the angle between the lines y = 2x and y = -3x. | y = (2/5)x |
| 3. In a triangle, the angle bisector of the vertex angle intersects the opposite side at a point that divides it into segments of length 3 and 5. Find the length of the third side of the triangle. | 8 |
| 4. Find the coordinates of the point of intersection of the lines y = 2x and y = -3x. | (0, 0) |
| 5. Find the slope of a line perpendicular to the line x + 2y = 3. | -1/2 |
| 6. Find the equation of a line that bisects the angle between the lines y = x and y = -2x. | y = (1/3)x |
| 7. In a triangle, the perpendicular bisector of the base intersects the vertex angle at a point that divides it into two equal angles. Find the measure of the vertex angle. | 60 degrees |
| 8. Find the coordinates of the point of intersection of the lines y = x + 2 and y = 2x - 3. | (5, 7) |
| 9. Find the slope of a line perpendicular to the line 3x - 2y = 1. | 2/3 |
| 10. Find the equation of a line that bisects the angle between the lines y = x + 1 and y = 2x - 1. | y = (3/5)x + 1/5 |
📝 Note: The answers to the worksheet problems are provided for reference only. It is recommended that you try to solve the problems on your own before checking the answers.
In conclusion, perpendicular lines and angle bisectors are fundamental concepts in geometry that have numerous applications in various fields. By understanding the properties and applications of these concepts, you can develop a deeper appreciation for the beauty and complexity of geometric shapes.
What is the difference between a perpendicular line and an angle bisector?
+A perpendicular line is a line that intersects another line at a 90-degree angle, while an angle bisector is a line that divides an angle into two equal parts.
How do I find the slope of a line perpendicular to a given line?
+To find the slope of a line perpendicular to a given line, you can take the negative reciprocal of the slope of the given line.
What is the concurrency of angle bisectors in a triangle?
+The angle bisectors of a triangle are concurrent, meaning they intersect at a single point.
Related Terms:
- Perpendicular bisector worksheet grade 7
- Angle bisector Worksheet PDF