5 Simple Ways to Solve Percent Problems
When solving percent problems, it’s essential to understand the concept of percentages and how they relate to fractions and decimals. Percentages are used to represent a fraction of a whole as a part of 100. In this article, we’ll explore five simple ways to solve percent problems.
Understanding Percentages
Before we dive into solving percent problems, let’s quickly review what percentages are. Percentages are a way to express a fraction as a part of 100. For example, 25% is equal to 25⁄100 or 1⁄4. To convert a percentage to a decimal, divide by 100. For instance, 25% becomes 0.25 when converted to a decimal.
Method 1: Finding a Percentage of a Number
One common type of percent problem is finding a percentage of a number. To solve this type of problem, follow these steps:
- Convert the percentage to a decimal by dividing by 100.
- Multiply the decimal by the number.
For example, find 25% of 120.
- Convert 25% to a decimal: 25 ÷ 100 = 0.25
- Multiply 0.25 by 120: 0.25 × 120 = 30
Therefore, 25% of 120 is 30.
📝 Note: You can also use the formula: Percentage × Number = Result
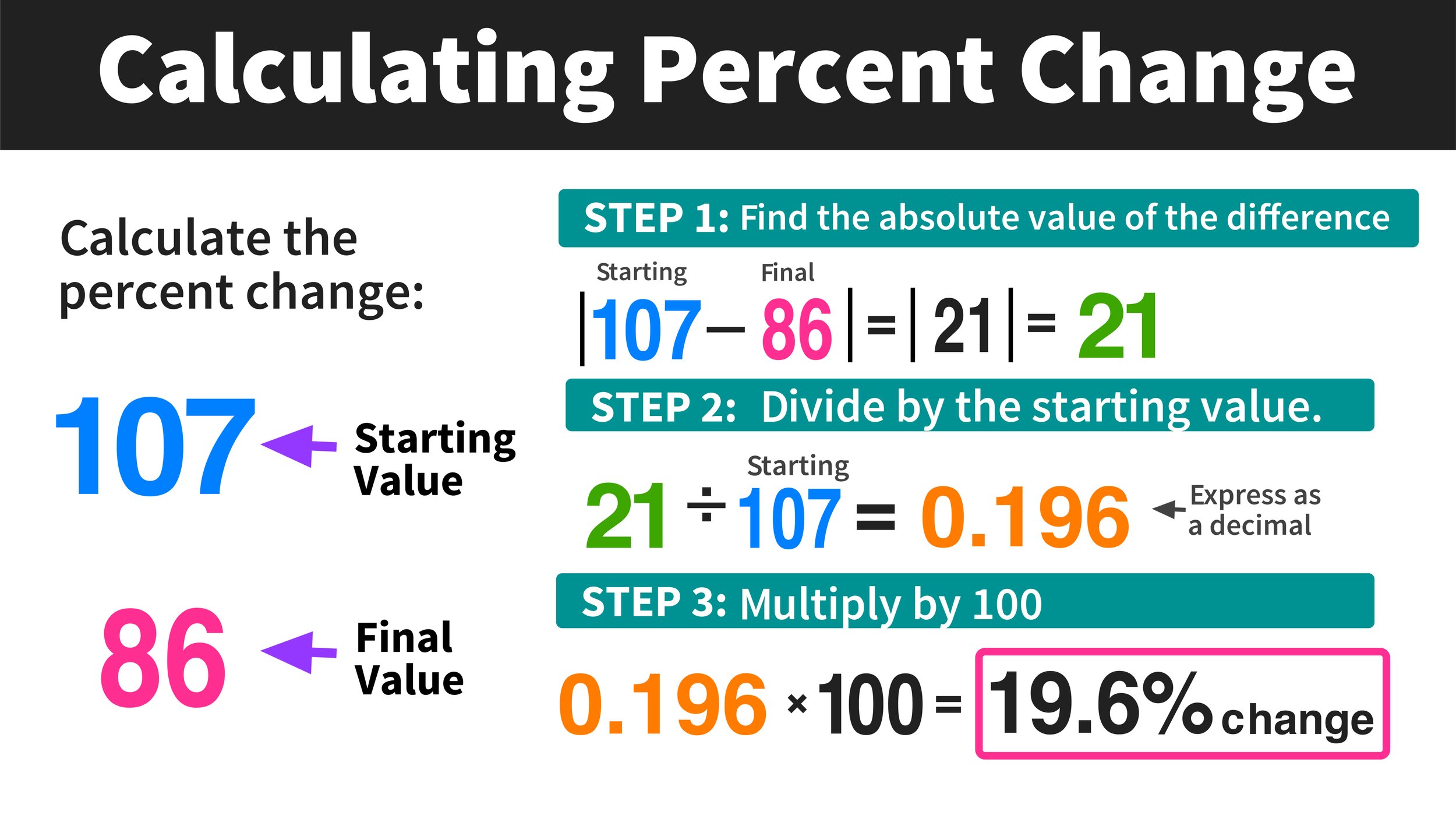
Method 2: Finding the Percentage Increase or Decrease
Another type of percent problem is finding the percentage increase or decrease between two numbers. To solve this type of problem, follow these steps:
- Find the difference between the two numbers.
- Divide the difference by the original number.
- Multiply by 100 to convert to a percentage.
For example, a shirt was originally priced at 80 and is now on sale for 64. What is the percentage decrease?
- Find the difference: 80 - 64 = $16
- Divide the difference by the original number: 16 ÷ 80 = 0.2
- Multiply by 100: 0.2 × 100 = 20%
Therefore, the percentage decrease is 20%.
Method 3: Finding the Original Number
In some cases, you may be given a percentage and a result, and you need to find the original number. To solve this type of problem, follow these steps:
- Convert the percentage to a decimal.
- Divide the result by the decimal.
For example, 25% of a number is 30. What is the original number?
- Convert 25% to a decimal: 25 ÷ 100 = 0.25
- Divide 30 by 0.25: 30 ÷ 0.25 = 120
Therefore, the original number is 120.
Method 4: Finding the Percentage of a Multi-Part Problem
Some percent problems involve multiple parts. To solve this type of problem, follow these steps:
- Break down the problem into smaller parts.
- Solve each part separately.
- Combine the results.
For example, a bakery sells 250 loaves of bread at 15% discount and 150 loaves at 20% discount. What is the total percentage of loaves sold at a discount?
- Calculate the number of loaves sold at 15% discount: 250 × 0.15 = 37.5
- Calculate the number of loaves sold at 20% discount: 150 × 0.20 = 30
- Combine the results: 37.5 + 30 = 67.5
Therefore, the total percentage of loaves sold at a discount is 67.5%.
Method 5: Using a Table to Solve Percent Problems
Sometimes, it’s helpful to use a table to solve percent problems. This method is particularly useful when dealing with multiple percentages. To use this method, follow these steps:
- Create a table with columns for the percentage, decimal, and result.
- Fill in the table with the given information.
- Use the table to calculate the desired result.
For example, a store offers a 10% discount on all items during a sale. If you purchase two items, one for 50 and one for 75, what is the total discount?
| Percentage | Decimal | Result |
|---|---|---|
| 10% | 0.10 | |
| 50 × 0.10 = 5 | ||
| 75 × 0.10 = 7.50 |
Therefore, the total discount is 5 + 7.50 = $12.50.
In conclusion, solving percent problems can be straightforward if you understand the concept of percentages and use the right methods. By using these five simple methods, you can easily solve a variety of percent problems.
What is the formula for finding a percentage of a number?
+The formula for finding a percentage of a number is: Percentage × Number = Result
How do I convert a percentage to a decimal?
+To convert a percentage to a decimal, divide by 100. For example, 25% becomes 0.25 when converted to a decimal.
What is the difference between a percentage increase and a percentage decrease?
+A percentage increase is a percentage that is added to a number, while a percentage decrease is a percentage that is subtracted from a number.