5 Essential Steps for Mastering Order of Operations

Mastering the Order of Operations: A Step-by-Step Guide
The order of operations is a fundamental concept in mathematics that helps individuals solve mathematical expressions in a logical and consistent manner. It is a set of rules that dictates the order in which mathematical operations should be performed when there are multiple operations in an expression. Mastering the order of operations is crucial for anyone who wants to excel in mathematics, and in this article, we will break down the essential steps to help you achieve this goal.
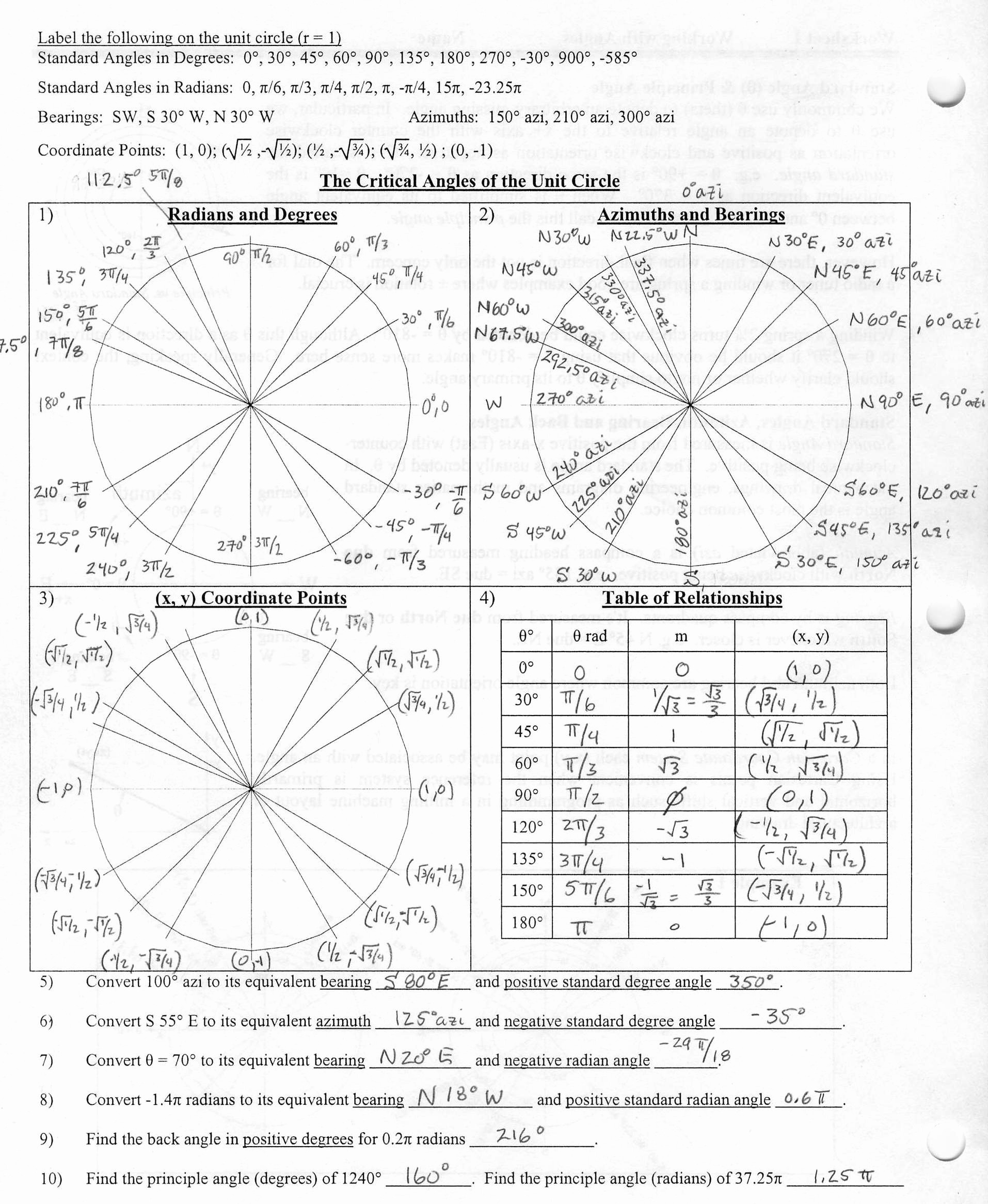
Step 1: Understand the Basics of the Order of Operations
The order of operations is commonly referred to as PEMDAS, which stands for Parentheses, Exponents, Multiplication and Division, and Addition and Subtraction. This acronym helps individuals remember the correct order in which mathematical operations should be performed. The rules are as follows:
- Parentheses: Evaluate expressions inside parentheses first. This means that any calculations within parentheses should be performed before moving on to the next step.
- Exponents: Evaluate any exponential expressions next. This includes any roots, such as square roots or cube roots.
- Multiplication and Division: Evaluate any multiplication and division operations from left to right. In other words, perform any multiplication operations before moving on to division operations.
- Addition and Subtraction: Finally, evaluate any addition and subtraction operations from left to right.
Step 2: Practice with Simple Expressions
Once you understand the basics of the order of operations, it’s time to practice with simple expressions. Start with expressions that involve only one or two operations, such as:
- 2 + 3 × 4
- 10 - 3 + 2
Evaluate these expressions using the order of operations, and check your answers to ensure you’re getting the correct results.
📝 Note: Make sure to write down each step as you evaluate the expression. This will help you visualize the process and ensure you're following the correct order.
Step 3: Move on to More Complex Expressions
Once you’re comfortable with simple expressions, it’s time to move on to more complex expressions that involve multiple operations. For example:
- 2 × (3 + 4) - 5
- 10 - 3 + 2 × (5 - 2)
Evaluate these expressions using the order of operations, and check your answers to ensure you’re getting the correct results.
📝 Note: When dealing with more complex expressions, make sure to follow the order of operations carefully. It's easy to get confused and perform operations out of order.
Step 4: Use Real-World Examples
To make the order of operations more relevant and interesting, try using real-world examples. For example:
- A bakery sells 250 loaves of bread per day. If each loaf costs $2, and the bakery operates 7 days a week, how much money does it make in a week?
- A car travels 250 miles in 5 hours. If the car travels at a constant speed, how many miles does it travel per hour?
Use the order of operations to evaluate these expressions, and check your answers to ensure you’re getting the correct results.
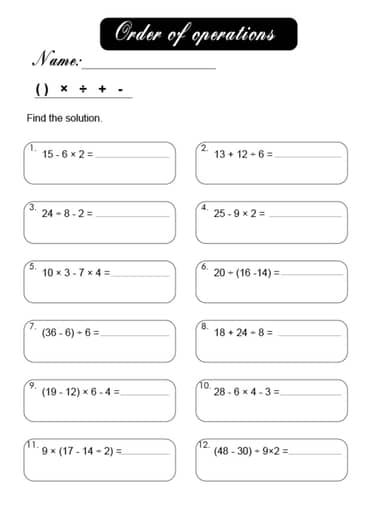
Step 5: Practice, Practice, Practice
The final step to mastering the order of operations is to practice regularly. Try solving different types of expressions, and gradually increase the difficulty level as you become more confident.
Here’s a table with some examples of expressions you can practice with:
| Expression | Answer |
|---|---|
| 2 + 3 × 4 | 14 |
| 10 - 3 + 2 | 9 |
| 2 × (3 + 4) - 5 | 9 |
| 10 - 3 + 2 × (5 - 2) | 12 |
By following these 5 essential steps, you’ll be well on your way to mastering the order of operations. Remember to practice regularly, and don’t be afraid to make mistakes. With time and practice, you’ll become more confident and proficient in your ability to solve mathematical expressions.
In conclusion, mastering the order of operations is a crucial skill for anyone who wants to excel in mathematics. By following these 5 essential steps, you’ll be able to evaluate mathematical expressions with ease and accuracy. Remember to practice regularly, and don’t be afraid to make mistakes. With time and practice, you’ll become a master of the order of operations.
What is the order of operations?
+The order of operations is a set of rules that dictates the order in which mathematical operations should be performed when there are multiple operations in an expression. The acronym PEMDAS helps individuals remember the correct order: Parentheses, Exponents, Multiplication and Division, and Addition and Subtraction.
Why is the order of operations important?
+The order of operations is important because it helps individuals solve mathematical expressions in a logical and consistent manner. Without a standardized order of operations, mathematical expressions can be ambiguous and lead to incorrect results.
How can I practice the order of operations?
+You can practice the order of operations by solving different types of expressions, gradually increasing the difficulty level as you become more confident. You can also use online resources, such as worksheets and practice tests, to help you practice.
Related Terms:
- Order of Operations worksheets pdf
- Fraction Worksheet Grade 5
- Parenthesis worksheet for grade 5