Simplifying Radicals Made Easy With This Essential Worksheet

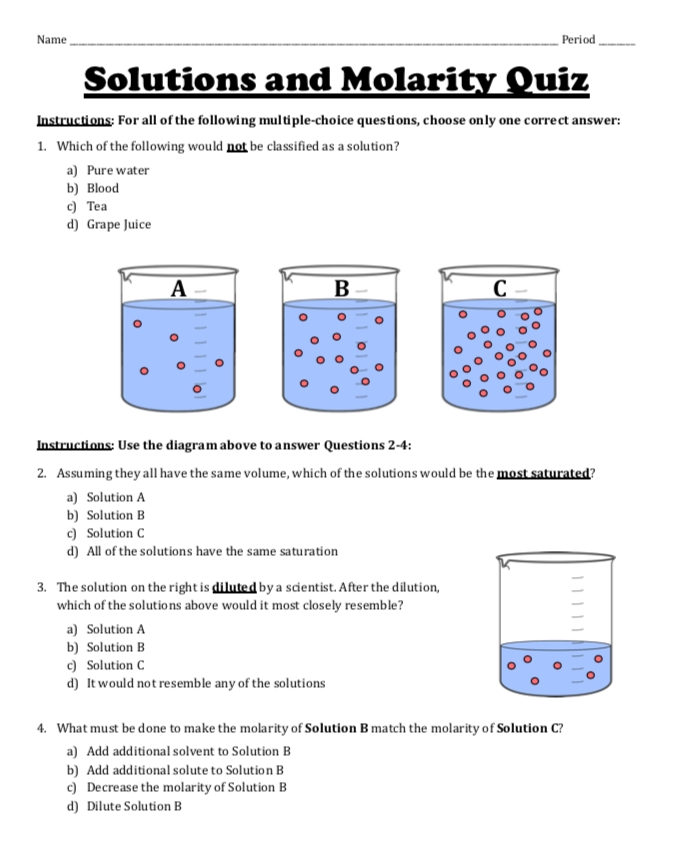
Unlocking the Secrets of Radicals: A Comprehensive Guide
Radicals, also known as roots, are a fundamental concept in mathematics, and understanding how to simplify them is crucial for success in various mathematical operations. Simplifying radicals can seem daunting at first, but with the right approach and practice, it can become second nature. In this article, we will delve into the world of radicals, explore the concept of simplifying radicals, and provide you with an essential worksheet to help you master this skill.
What are Radicals?
Radicals are mathematical expressions that contain a root symbol (√). They represent a number that, when multiplied by itself, gives a specified value. For example, √16 represents a number that, when multiplied by itself, equals 16. In this case, the answer is 4, since 4 × 4 = 16.
Why Simplify Radicals?
Simplifying radicals is essential in mathematics because it allows us to:
- Simplify complex expressions: Radicals can be used to simplify complex mathematical expressions, making them easier to work with.
- Perform calculations: Simplifying radicals enables us to perform calculations, such as addition, subtraction, multiplication, and division, with ease.
- Understand mathematical concepts: Simplifying radicals is a fundamental skill required to understand various mathematical concepts, including algebra, geometry, and calculus.
How to Simplify Radicals
Simplifying radicals involves factoring out the largest perfect square from the radicand (the number inside the square root symbol). Here’s a step-by-step guide:
- Identify the radicand: Determine the number inside the square root symbol.
- Find the largest perfect square: Identify the largest perfect square that divides the radicand.
- Factor out the perfect square: Rewrite the radicand as a product of the perfect square and another factor.
- Simplify the radical: Simplify the radical by taking the square root of the perfect square.
Example: Simplify √48.
- Identify the radicand: 48
- Find the largest perfect square: 16
- Factor out the perfect square: 48 = 16 × 3
- Simplify the radical: √48 = √(16 × 3) = 4√3
Common Mistakes to Avoid
When simplifying radicals, it’s essential to avoid common mistakes, including:
- Not factoring out the largest perfect square: Failing to factor out the largest perfect square can result in an incorrect answer.
- Not simplifying the radical: Failing to simplify the radical can lead to a complex expression.
Essential Worksheet: Simplifying Radicals
Practice makes perfect! To help you master the skill of simplifying radicals, we’ve created an essential worksheet. Download the worksheet and practice simplifying radicals to improve your mathematical skills.
Worksheet: Simplifying Radicals
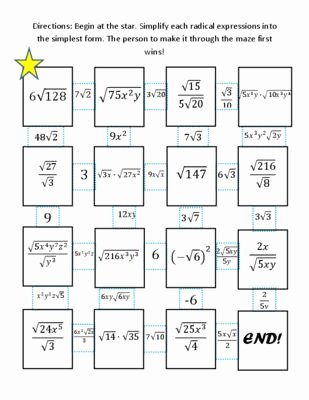
| Radical | Simplified Form |
|---|---|
| √12 | |
| √48 | |
| √75 | |
| √200 | |
| √300 |
Answer Key:
| Radical | Simplified Form |
|---|---|
| √12 | 2√3 |
| √48 | 4√3 |
| √75 | 5√3 |
| √200 | 10√2 |
| √300 | 10√3 |
Notes
📝 Note: When simplifying radicals, it's essential to check your work by plugging the simplified form back into the original expression.
Conclusion
Simplifying radicals is a fundamental skill in mathematics that requires practice and patience. By following the steps outlined in this article and practicing with the essential worksheet, you’ll become proficient in simplifying radicals in no time. Remember to avoid common mistakes and always check your work to ensure accuracy.
What is the purpose of simplifying radicals?
+Simplifying radicals allows us to simplify complex expressions, perform calculations, and understand mathematical concepts.
How do I simplify a radical?
+To simplify a radical, identify the radicand, find the largest perfect square, factor out the perfect square, and simplify the radical.
What are common mistakes to avoid when simplifying radicals?
+Common mistakes to avoid include not factoring out the largest perfect square and not simplifying the radical.