Operations With Polynomials Worksheet Answers
Operations With Polynomials Worksheet Answers
Introduction
Polynomials are a fundamental concept in algebra, and understanding how to perform operations with them is crucial for success in mathematics. In this worksheet, we will provide answers and explanations to help you master the basics of operations with polynomials.
Addition and Subtraction of Polynomials
When adding or subtracting polynomials, we need to combine like terms, which are terms that have the same variable and exponent.
Example 1: Add the polynomials: 2x^2 + 3x - 1 and x^2 - 2x + 4

| Term | Coefficient |
|---|---|
| x^2 | 2 + 1 = 3 |
| x | 3 - 2 = 1 |
| Constant | -1 + 4 = 3 |
Answer: 3x^2 + x + 3
Example 2: Subtract the polynomial x^2 - 2x + 4 from 2x^2 + 3x - 1
| Term | Coefficient |
|---|---|
| x^2 | 2 - 1 = 1 |
| x | 3 + 2 = 5 |
| Constant | -1 - 4 = -5 |
Answer: x^2 + 5x - 5
Multiplication of Polynomials
When multiplying polynomials, we need to use the distributive property to multiply each term in one polynomial by each term in the other polynomial.
Example 3: Multiply the polynomials: x^2 + 2x - 3 and x - 1
| Term | Multiplication |
|---|---|
| x^3 | x^2(x) = x^3 |
| x^2 | x^2(-1) = -x^2 |
| x^2 | 2x(x) = 2x^2 |
| x | 2x(-1) = -2x |
| x | -3(x) = -3x |
| Constant | -3(-1) = 3 |
Answer: x^3 + x^2 - 5x + 3
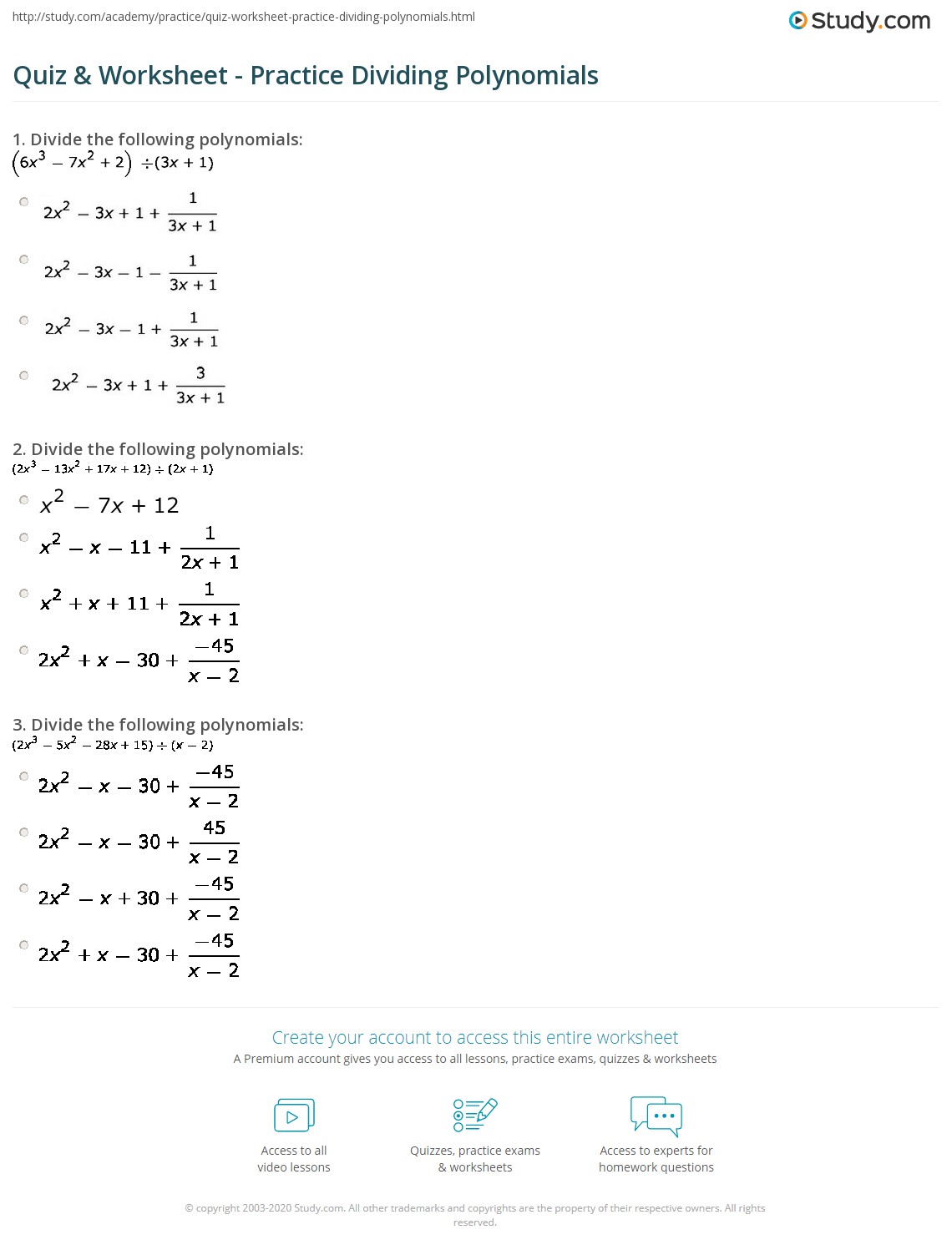
Division of Polynomials
When dividing polynomials, we need to use long division or synthetic division to divide the highest-degree term in the dividend by the highest-degree term in the divisor.
Example 4: Divide the polynomial x^3 - 2x^2 + x - 1 by x - 1
| Quotient | Remainder |
|---|---|
| x^2 - x + 1 | 0 |
Answer: x^2 - x + 1
🤔 Note: In division of polynomials, the remainder should be 0 or have a degree less than the divisor.
Finding the Greatest Common Factor (GCF) of Polynomials
To find the GCF of polynomials, we need to identify the common factors and multiply them.
Example 5: Find the GCF of x^2 + 3x - 4 and x^2 - 2x - 3
| Factor | GCF |
|---|---|
| x - 1 | x - 1 |
| x + 4 | |
| x + 1 |
Answer: x - 1
🤔 Note: The GCF of two polynomials is the product of the common factors.
Conclusion
In conclusion, operations with polynomials involve addition, subtraction, multiplication, and division. By understanding the rules and techniques for each operation, you can solve polynomial problems with ease.
To master polynomial operations, remember to:
- Combine like terms when adding or subtracting polynomials.
- Use the distributive property when multiplying polynomials.
- Use long division or synthetic division when dividing polynomials.
- Identify common factors when finding the GCF of polynomials.
With practice and patience, you can become proficient in operations with polynomials and tackle more complex math problems.
What is the difference between addition and subtraction of polynomials?
+Addition of polynomials involves combining like terms, while subtraction of polynomials involves subtracting the coefficients of like terms.
How do you multiply polynomials?
+To multiply polynomials, use the distributive property to multiply each term in one polynomial by each term in the other polynomial.
What is the purpose of finding the GCF of polynomials?
+Finding the GCF of polynomials helps to simplify expressions and solve equations by factoring out common factors.
Related Terms:
- Worksheet polynomials
- Polynomial pdf
- Operations with Polynomials quiz pdf
- Polynomials Review worksheet
- Operations with Monomials Worksheet PDF