Master Complex Numbers with Easy Operations Worksheet
Unlocking the Power of Complex Numbers: A Comprehensive Guide
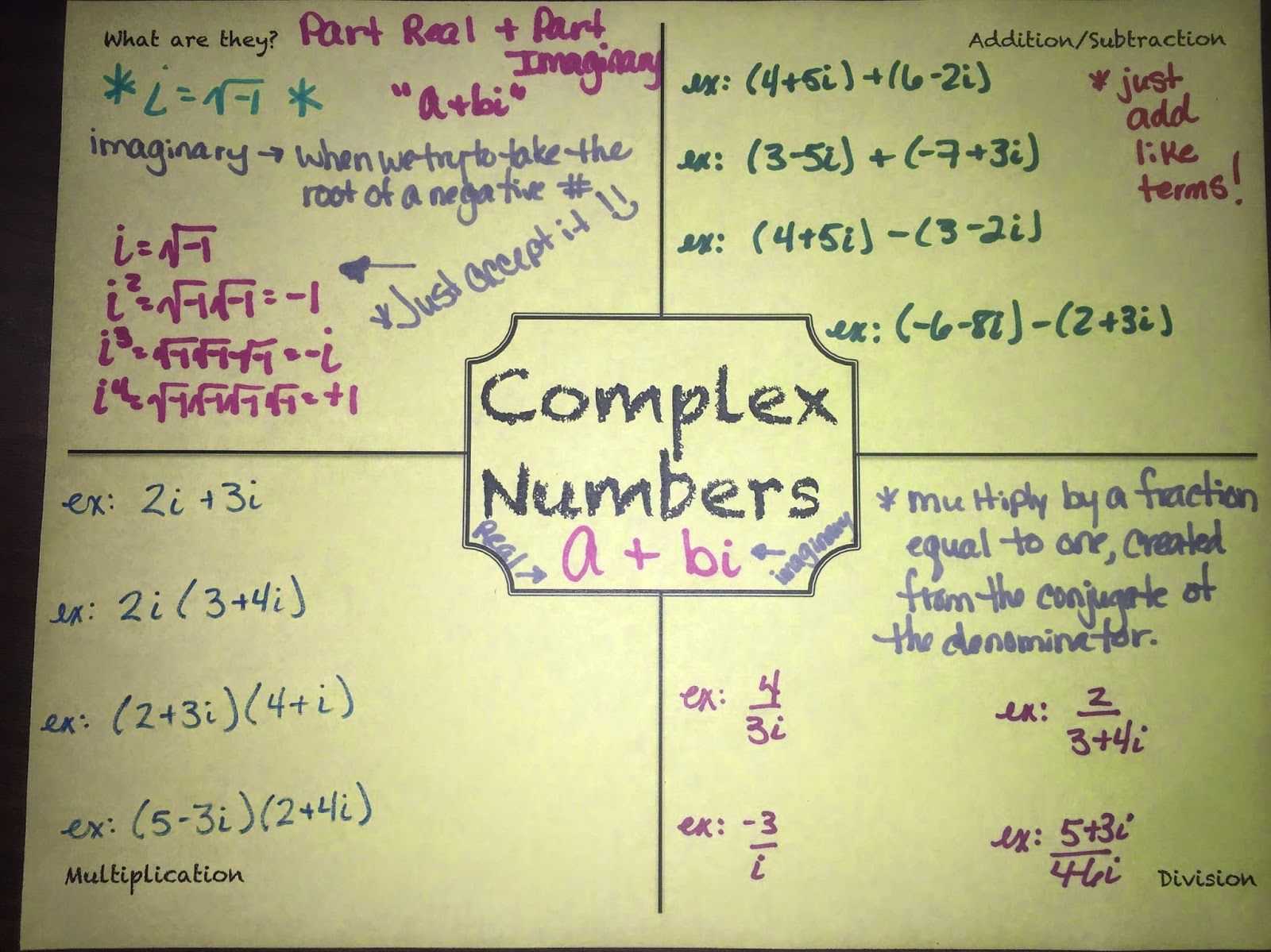
Complex numbers are a fundamental concept in mathematics, extending the real number system to include imaginary numbers. They have numerous applications in various fields, including engineering, physics, and computer science. In this article, we will delve into the world of complex numbers, exploring their definition, properties, and operations. With the help of an easy-to-use worksheet, you will master complex numbers in no time.
What are Complex Numbers?
A complex number is a number that can be expressed in the form:
a + bi
where:
- a is the real part of the complex number
- b is the imaginary part of the complex number
- i is the imaginary unit, satisfying the equation i^2 = -1
In other words, complex numbers are numbers that have both real and imaginary parts.
Properties of Complex Numbers
Complex numbers have several important properties that make them useful in various mathematical and scientific applications. Some of these properties include:
- Addition and Multiplication: Complex numbers can be added and multiplied, just like real numbers.
- Conjugates: The conjugate of a complex number a + bi is a - bi.
- Magnitude: The magnitude (or modulus) of a complex number a + bi is √(a^2 + b^2).
- Argument: The argument (or angle) of a complex number a + bi is the angle θ between the positive real axis and the line connecting the origin to the point (a, b) in the complex plane.
Operations with Complex Numbers
Now that we have explored the properties of complex numbers, let’s dive into the various operations that can be performed with them.
Addition and Subtraction
To add or subtract complex numbers, simply add or subtract the real parts and the imaginary parts separately.
- (a + bi) + (c + di) = (a + c) + (b + d)i
- (a + bi) - (c + di) = (a - c) + (b - d)i
Multiplication
To multiply complex numbers, use the distributive property and the fact that i^2 = -1.
- (a + bi) × (c + di) = (ac - bd) + (ad + bc)i
Division
To divide complex numbers, multiply the numerator and denominator by the conjugate of the denominator.
- (a + bi) ÷ (c + di) = (a + bi)(c - di) / (c^2 + d^2)
Exponentiation
To raise a complex number to a power, use De Moivre’s theorem, which states that:
- (cosθ + isinθ)^n = cos(nθ) + isin(nθ)
🤔 Note: This is a simplified explanation of De Moivre's theorem. For a more detailed explanation, please consult a mathematics textbook.
Easy Operations Worksheet
Now that we have explored the operations with complex numbers, it’s time to practice with an easy-to-use worksheet.
Exercise 1: Addition and Subtraction
| Complex Number 1 | Complex Number 2 | Result |
|---|---|---|
| 2 + 3i | 4 + 5i | |
| 3 - 2i | 1 + 4i |
Exercise 2: Multiplication
| Complex Number 1 | Complex Number 2 | Result |
|---|---|---|
| 2 + 3i | 4 + 5i | |
| 3 - 2i | 1 + 4i |
Exercise 3: Division
| Complex Number 1 | Complex Number 2 | Result |
|---|---|---|
| 2 + 3i | 4 + 5i | |
| 3 - 2i | 1 + 4i |
Exercise 4: Exponentiation
| Complex Number | Power | Result |
|---|---|---|
| 2 + 3i | 2 | |
| 3 - 2i | 3 |
To check your answers, simply follow the operations outlined above.
Conclusion
Mastering complex numbers requires practice and patience. With this comprehensive guide and easy-to-use worksheet, you are well on your way to becoming proficient in complex number operations. Remember to always follow the rules of arithmetic and algebra, and don’t be afraid to practice with different types of complex numbers.
What is the imaginary unit i?
+The imaginary unit i is a number that satisfies the equation i^2 = -1.
How do I add complex numbers?
+To add complex numbers, simply add the real parts and the imaginary parts separately.
What is De Moivre’s theorem?
+De Moivre’s theorem states that (cosθ + isinθ)^n = cos(nθ) + isin(nθ).