Mastering Negative Exponents with Easy Worksheet
Understanding Negative Exponents
Negative exponents can be a daunting concept for many students, but with a solid understanding of the basics and plenty of practice, you’ll be a pro in no time. In this article, we’ll explore the world of negative exponents, including what they are, how to work with them, and some easy-to-use worksheets to help you master the concept.
What are Negative Exponents?
A negative exponent is an exponent that is less than zero. In other words, it’s an exponent that represents a fraction or a decimal. For example, in the expression 2^(-3), the -3 is a negative exponent. Negative exponents are often used to represent very small or very large numbers in a more manageable way.
Rules for Working with Negative Exponents
Here are some basic rules to keep in mind when working with negative exponents:
- Negative Exponent Rule: a^(-n) = 1/a^n
- Product Rule: a^(-m) × a^(-n) = a^(-(m+n))
- Quotient Rule: a^(-m) ÷ a^(-n) = a^(-(m-n))
🤔 Note: When working with negative exponents, it's essential to remember that a negative exponent is equivalent to taking the reciprocal of the base raised to the positive exponent.
How to Simplify Negative Exponents
Simplifying negative exponents can be a bit tricky, but with practice, you’ll get the hang of it. Here’s a step-by-step guide on how to simplify negative exponents:
- Step 1: Identify the negative exponent and the base.
- Step 2: Apply the negative exponent rule (a^(-n) = 1/a^n).
- Step 3: Simplify the resulting fraction.
Example:
Simplify: 2^(-3)
- Step 1: Identify the negative exponent (-3) and the base (2).
- Step 2: Apply the negative exponent rule: 2^(-3) = 1⁄2^3
- Step 3: Simplify the resulting fraction: 1⁄2^3 = 1⁄8
Common Mistakes to Avoid
When working with negative exponents, here are some common mistakes to avoid:
- Mistake 1: Forgetting to apply the negative exponent rule.
- Mistake 2: Not simplifying the resulting fraction.
- Mistake 3: Confusing negative exponents with positive exponents.
📝 Note: Practice, practice, practice! The more you practice working with negative exponents, the more comfortable you'll become with the concept.
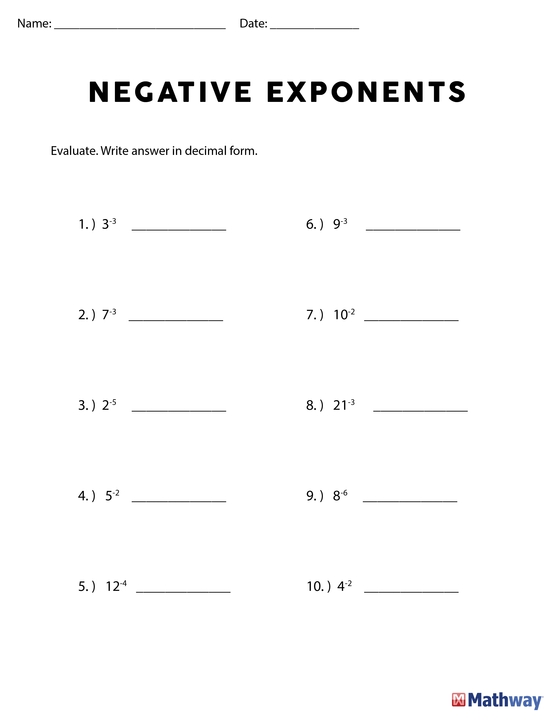
Negative Exponents Worksheet
Here’s a worksheet to help you practice working with negative exponents:
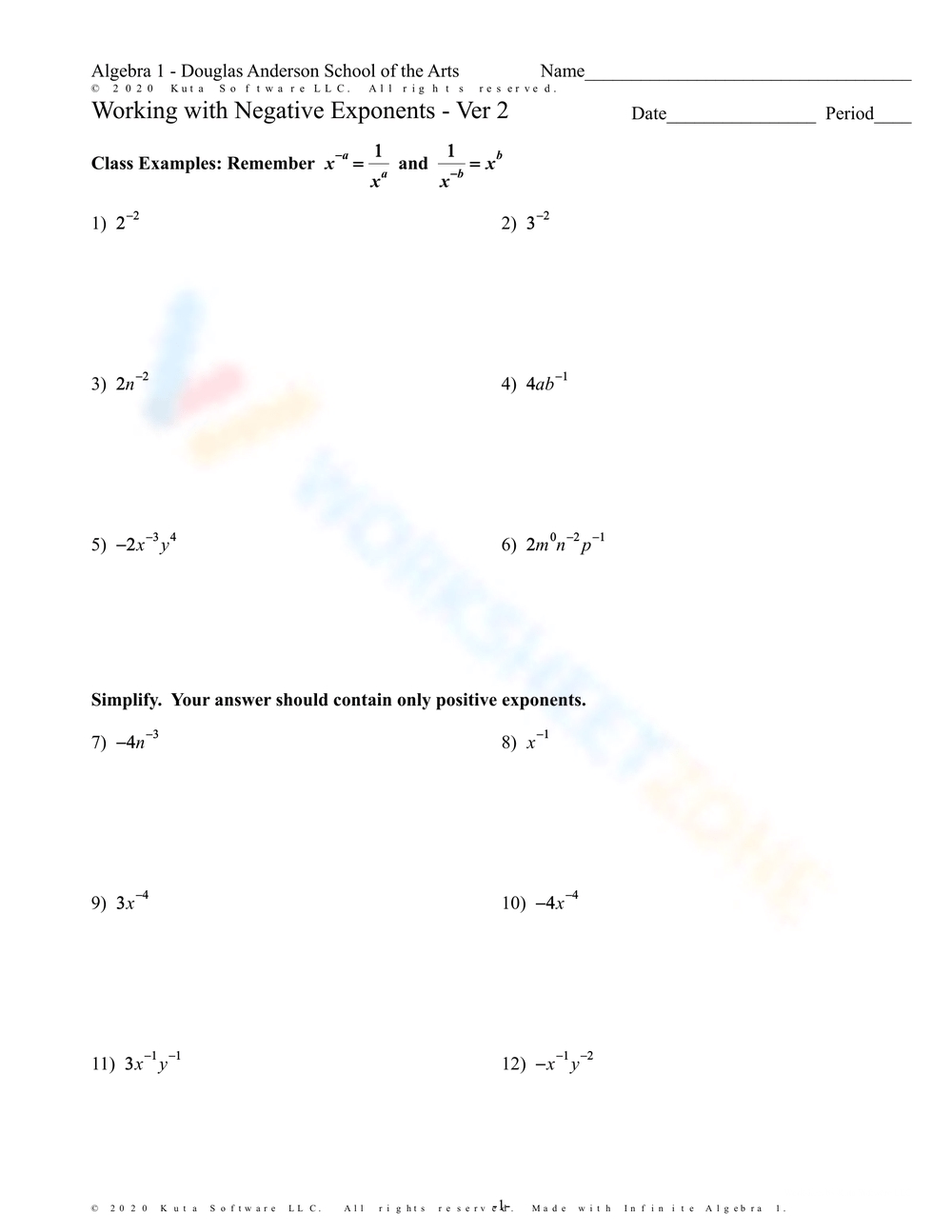
| Expression | Simplified Form |
|---|---|
| 2^(-1) | |
| 3^(-2) | |
| 4^(-3) | |
| 2^(-4) × 2^(-5) | |
| 3^(-2) ÷ 3^(-4) |
Answers:
| Expression | Simplified Form |
|---|---|
| 2^(-1) | 1⁄2 |
| 3^(-2) | 1⁄9 |
| 4^(-3) | 1⁄64 |
| 2^(-4) × 2^(-5) | 1⁄2^9 |
| 3^(-2) ÷ 3^(-4) | 3^2 |
Conclusion
Mastering negative exponents takes time and practice, but with the right mindset and resources, you can become a pro in no time. Remember to apply the negative exponent rule, simplify resulting fractions, and avoid common mistakes. Practice with the worksheet provided and soon you’ll be working with negative exponents like a breeze.
What is the purpose of negative exponents?
+Negative exponents are used to represent very small or very large numbers in a more manageable way.
How do I simplify negative exponents?
+To simplify negative exponents, apply the negative exponent rule (a^(-n) = 1/a^n), and then simplify the resulting fraction.
What are some common mistakes to avoid when working with negative exponents?
+Common mistakes to avoid include forgetting to apply the negative exponent rule, not simplifying resulting fractions, and confusing negative exponents with positive exponents.