Angles Worksheet: Identify and Name Angles with Ease

Understanding Angles and Their Types

Angles are a fundamental concept in geometry, and understanding them is crucial for various mathematical and real-world applications. In this article, we will delve into the world of angles, exploring their types, properties, and how to identify and name them with ease.

What is an Angle?

An angle is formed when two rays or lines intersect at a common point, called the vertex. The angle is measured in degrees, with 360 degrees making a full circle. Angles can be classified into different types based on their measure and orientation.

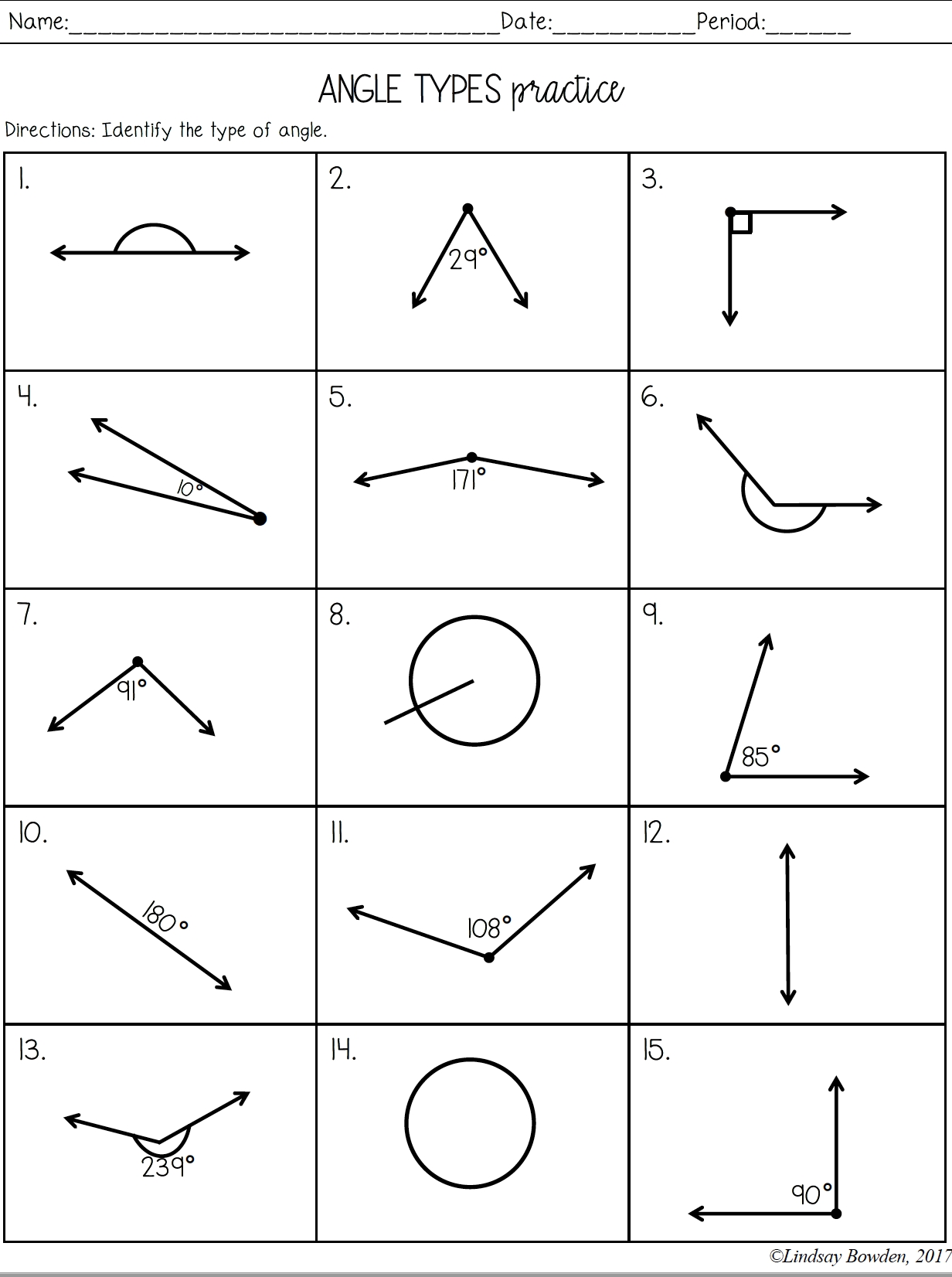
Types of Angles

There are several types of angles, including:

• Acute Angle: An angle whose measure is less than 90 degrees.
• Right Angle: An angle whose measure is exactly 90 degrees.
• Obtuse Angle: An angle whose measure is greater than 90 degrees but less than 180 degrees.
• Straight Angle: An angle whose measure is exactly 180 degrees.
• Reflex Angle: An angle whose measure is greater than 180 degrees but less than 360 degrees.

Naming Angles

Angles can be named using different methods, including:

• Vertex Method: Naming an angle by its vertex, using a single letter or symbol.
• Ray Method: Naming an angle by its two rays, using three letters or symbols.
• Measure Method: Naming an angle by its measure, using degrees.

Identifying Angles

To identify an angle, you need to determine its measure and type. Here are some steps to follow:

1. Determine the Vertex: Identify the common point where the two rays or lines intersect.
2. Determine the Rays: Identify the two rays or lines that form the angle.
3. Measure the Angle: Use a protractor or angle measurer to determine the angle’s measure.
4. Classify the Angle: Based on the measure, classify the angle as acute, right, obtuse, straight, or reflex.

📝 Note: When measuring angles, make sure to use the correct unit of measurement, which is degrees (°).

Angle Relationships

Angles can be related to each other in different ways, including:

• Adjacent Angles: Angles that share a common vertex and one common ray.
• Complementary Angles: Angles whose measures add up to 90 degrees.
• Supplementary Angles: Angles whose measures add up to 180 degrees.

Angle Properties

Angles have several properties that are important to understand, including:

• Angle Addition: The sum of the measures of two or more angles.
• Angle Subtraction: The difference between the measures of two or more angles.
• Angle Equality: Two or more angles with the same measure.

Real-World Applications of Angles

Angles have numerous real-world applications, including:

• Architecture: Angles are used in building design, construction, and layout.
• Engineering: Angles are used in mechanical engineering, civil engineering, and aerospace engineering.
• Navigation: Angles are used in navigation, including piloting and sailing.

Conclusion

Angles are a fundamental concept in geometry, and understanding them is crucial for various mathematical and real-world applications. By identifying and naming angles with ease, you can improve your problem-solving skills and apply angle concepts to real-world situations.