5 Ways to Multiply Mixed Fractions Easily
Multiplying Mixed Fractions with Ease
Multiplying mixed fractions can be a daunting task, especially for students who are new to fractions. However, with the right techniques and strategies, it can be made much easier. In this article, we will explore five ways to multiply mixed fractions easily.
Understanding Mixed Fractions
Before we dive into the multiplication of mixed fractions, let’s first understand what mixed fractions are. A mixed fraction is a combination of a whole number and a fraction. For example, 2 1⁄3 is a mixed fraction, where 2 is the whole number and 1⁄3 is the fraction.
Method 1: Converting to Improper Fractions
One way to multiply mixed fractions is to convert them to improper fractions first. An improper fraction is a fraction where the numerator is greater than the denominator. To convert a mixed fraction to an improper fraction, we multiply the denominator by the whole number and add the numerator.
📝 Note: Make sure to multiply the denominator by the whole number, not the numerator.
For example, let’s convert 2 1⁄3 to an improper fraction:
2 × 3 = 6 6 + 1 = 7
So, 2 1⁄3 is equal to 7⁄3.
Method 2: Using the Standard Multiplication Method
Another way to multiply mixed fractions is to use the standard multiplication method. This involves multiplying the numerators and denominators separately and then simplifying the result.
For example, let’s multiply 2 1⁄3 and 3 1⁄2:
Numerators: 1 × 1 = 1 Denominators: 3 × 2 = 6 Whole numbers: 2 × 3 = 6
So, the result is 6 1⁄6.
Method 3: Using the Box Method
The box method is a visual way to multiply mixed fractions. This involves drawing a box with the numerators and denominators in each corner and then multiplying the numbers in each corner.
For example, let’s multiply 2 1⁄3 and 3 1⁄2 using the box method:
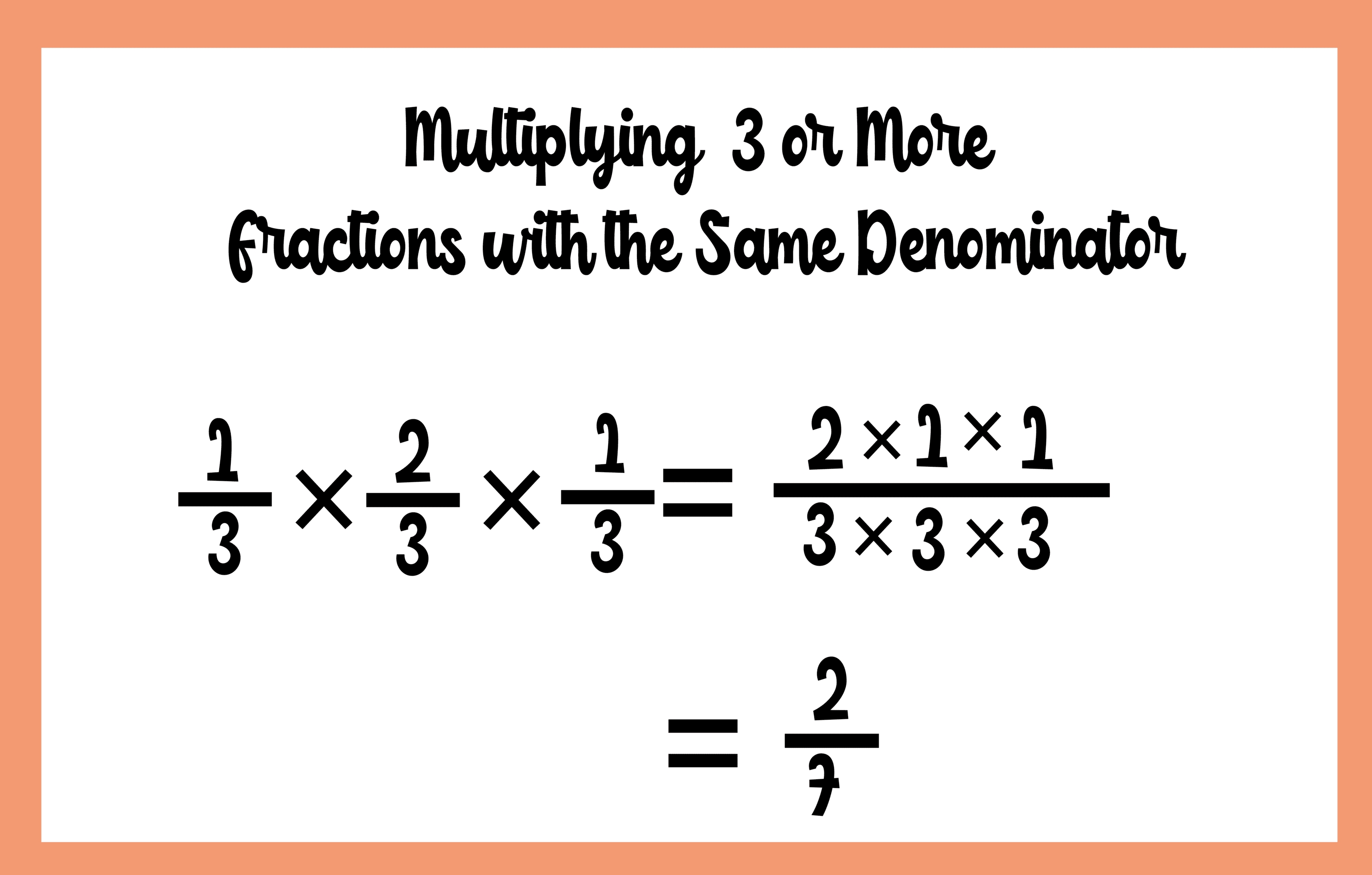
| 2 | 1 |
| 3 | 1 |
Numerators: 1 × 1 = 1 Denominators: 3 × 2 = 6 Whole numbers: 2 × 3 = 6
So, the result is 6 1⁄6.
Method 4: Using the Lattice Method
The lattice method is another visual way to multiply mixed fractions. This involves drawing a lattice with the numerators and denominators in each corner and then multiplying the numbers in each corner.
For example, let’s multiply 2 1⁄3 and 3 1⁄2 using the lattice method:
| 2 | 1 |
| 3 | 1 |
Numerators: 1 × 1 = 1 Denominators: 3 × 2 = 6 Whole numbers: 2 × 3 = 6
So, the result is 6 1⁄6.
Method 5: Using Online Calculators
Finally, we can use online calculators to multiply mixed fractions. This is a quick and easy way to get the result, especially for complex fractions.
For example, let’s multiply 2 1⁄3 and 3 1⁄2 using an online calculator:
So, the result is 6 1⁄6.
In conclusion, multiplying mixed fractions can be made easy with the right techniques and strategies. By converting to improper fractions, using the standard multiplication method, the box method, the lattice method, or online calculators, we can get the result quickly and accurately.
What is a mixed fraction?
+A mixed fraction is a combination of a whole number and a fraction.
How do I convert a mixed fraction to an improper fraction?
+To convert a mixed fraction to an improper fraction, multiply the denominator by the whole number and add the numerator.
What is the box method for multiplying mixed fractions?
+The box method is a visual way to multiply mixed fractions by drawing a box with the numerators and denominators in each corner and then multiplying the numbers in each corner.