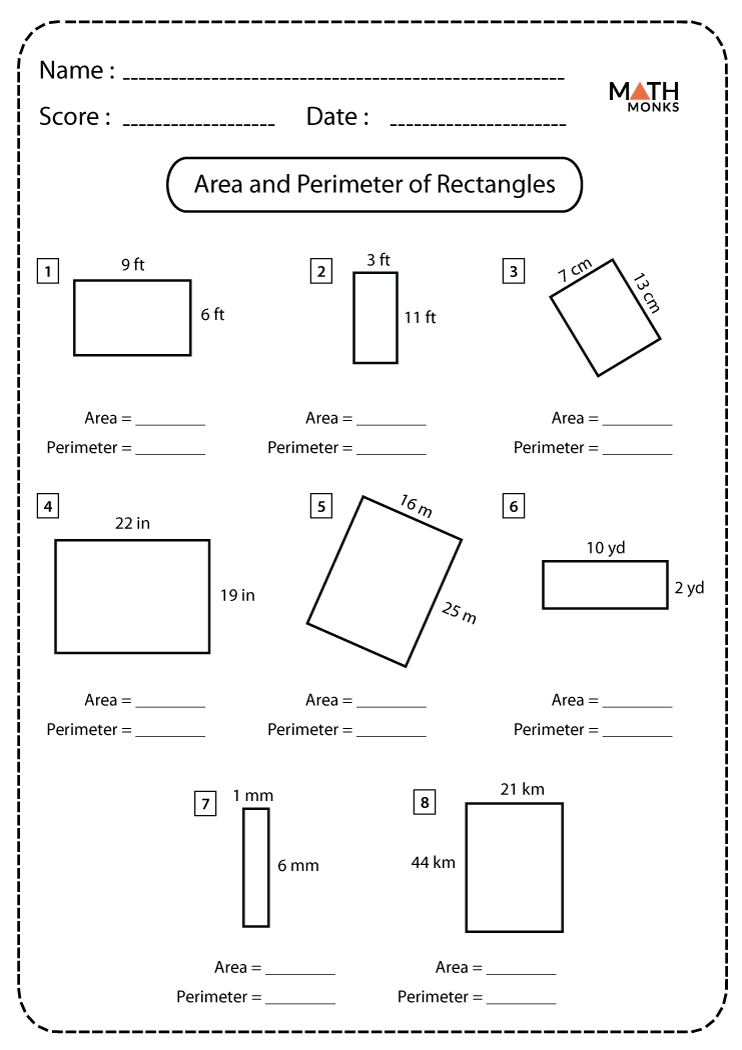
5 Ways to Master Fraction Multiplication

Unlocking the Secrets of Fraction Multiplication
Fraction multiplication is a fundamental concept in mathematics that can be intimidating for many students. However, with the right approach and practice, mastering fraction multiplication can become a breeze. In this article, we will explore five effective ways to master fraction multiplication, making it easier for you to tackle even the most complex math problems.
Understanding the Basics of Fraction Multiplication
Before diving into the advanced techniques, it’s essential to understand the basics of fraction multiplication. To multiply two fractions, you need to multiply the numerators (the numbers on top) and multiply the denominators (the numbers on the bottom). The resulting fraction is the product of the numerators divided by the product of the denominators.
Example:
Multiply 1⁄2 and 3⁄4
- Multiply the numerators: 1 × 3 = 3
- Multiply the denominators: 2 × 4 = 8
- The resulting fraction is: 3⁄8
Way 1: Using Real-World Examples
One of the most effective ways to master fraction multiplication is to use real-world examples. This approach helps students understand the practical application of fraction multiplication, making it more engaging and memorable.
Example:
Tom has 1⁄2 of a pizza, and he wants to share it with his friend, Alex, who has 3⁄4 of a pizza. How much pizza do they have in total?
- Multiply the fractions: 1⁄2 × 3⁄4 = 3⁄8
- The total amount of pizza they have is 3⁄8.
Way 2: Visualizing with Diagrams
Visualizing fraction multiplication with diagrams can help students understand the concept better. By using diagrams, students can see how the fractions are being multiplied, making it easier to comprehend.
Example:
Multiply 2⁄3 and 3⁄5 using a diagram.
| 1⁄3 | 1⁄3 | 1⁄3 | |
|---|---|---|---|
| 1⁄5 | 1⁄15 | 1⁄15 | 1⁄15 |
| 1⁄5 | 1⁄15 | 1⁄15 | 1⁄15 |
| 1⁄5 | 1⁄15 | 1⁄15 | 1⁄15 |
- The resulting fraction is: 6⁄15 = 2⁄5
Way 3: Breaking Down Complex Fractions
Breaking down complex fractions into simpler ones can make fraction multiplication more manageable. By simplifying the fractions, students can reduce the complexity of the problem, making it easier to solve.
Example:
Multiply 3⁄4 and 2⁄3.
- Break down the fractions: 3⁄4 = 1⁄4 + 1⁄4 + 1⁄4 and 2⁄3 = 1⁄3 + 1⁄3
- Multiply the simplified fractions: (1⁄4 + 1⁄4 + 1⁄4) × (1⁄3 + 1⁄3) = 1⁄12 + 1⁄12 + 1⁄12 + 1⁄12 + 1⁄12 + 1⁄12
- The resulting fraction is: 6⁄12 = 1⁄2
Way 4: Using the Area Model
The area model is a powerful tool for visualizing fraction multiplication. By using the area model, students can see how the fractions are being multiplied, making it easier to understand the concept.
Example:
Multiply 2⁄3 and 3⁄4 using the area model.
Rectangle with a width of 2⁄3 and a length of 3⁄4
- The area of the rectangle is: 2⁄3 × 3⁄4 = 6⁄12 = 1⁄2
Way 5: Practicing with Different Types of Fractions
Practicing with different types of fractions, such as mixed numbers and improper fractions, can help students become more confident in their ability to multiply fractions.
Example:
Multiply 2 1⁄3 and 3 3⁄4.
- Convert the mixed numbers to improper fractions: 2 1⁄3 = 7⁄3 and 3 3⁄4 = 15⁄4
- Multiply the improper fractions: 7⁄3 × 15⁄4 = 105⁄12
- The resulting fraction is: 105⁄12 = 8 9⁄12
📝 Note: When multiplying fractions, it's essential to simplify the resulting fraction to its simplest form.
In conclusion, mastering fraction multiplication requires practice, patience, and persistence. By using real-world examples, visualizing with diagrams, breaking down complex fractions, using the area model, and practicing with different types of fractions, you can become more confident in your ability to multiply fractions.
What is the best way to multiply fractions?
+The best way to multiply fractions is to multiply the numerators and multiply the denominators, then simplify the resulting fraction to its simplest form.
How do I visualize fraction multiplication?
+You can visualize fraction multiplication using diagrams, such as the area model, to help you understand how the fractions are being multiplied.
What is the difference between multiplying fractions and multiplying whole numbers?
+When multiplying fractions, you need to multiply the numerators and multiply the denominators, whereas when multiplying whole numbers, you simply multiply the numbers together.