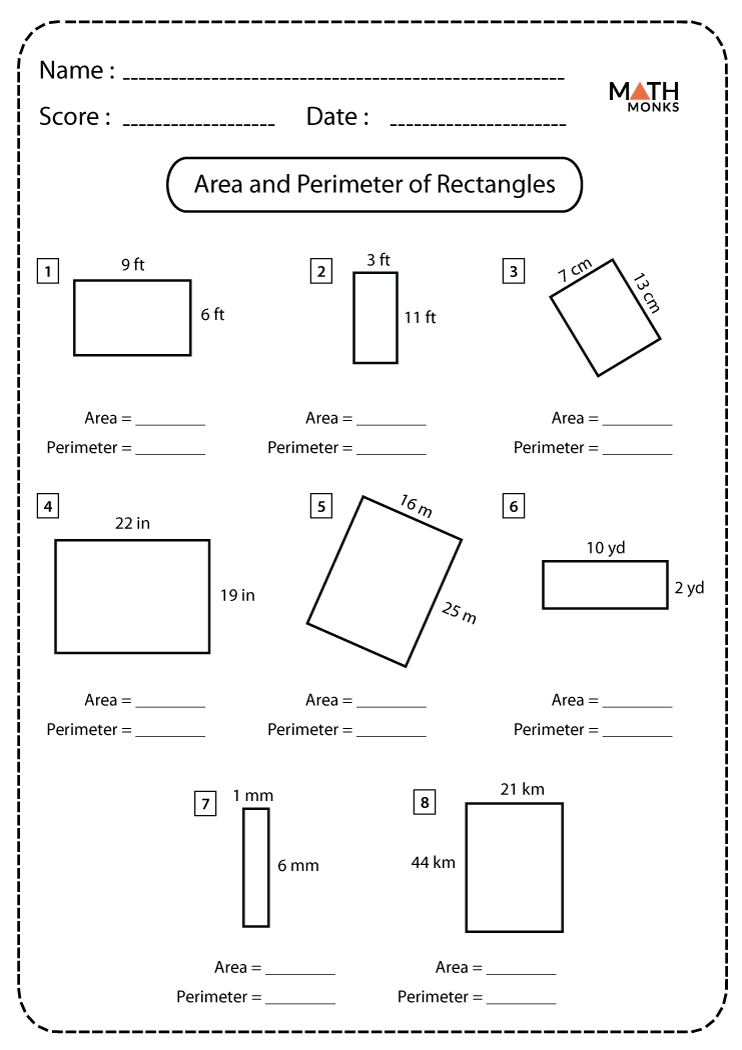
Understanding Area and Perimeter
Mathematics is a fundamental subject that requires a strong foundation in various concepts, including area and perimeter. These two terms are often used in geometry and are essential in calculating the size and shape of different objects. In this article, we will delve into the world of area and perimeter, exploring their definitions, formulas, and practical applications.
What is Area?
The area of a shape is the amount of space inside the shape. It is typically measured in square units, such as square meters, square feet, or square inches. The area of a shape can be calculated using various formulas, depending on the type of shape. For example, the area of a rectangle is calculated by multiplying its length and width.
Formulas for Calculating Area
Here are some common formulas for calculating the area of different shapes:
- Rectangle: Area = Length Γ Width
- Triangle: Area = (Base Γ Height) / 2
- Circle: Area = Ο Γ Radius^2
- Square: Area = Side^2
π Note: Ο (pi) is a mathematical constant approximately equal to 3.14.
What is Perimeter?
The perimeter of a shape is the distance around the shape. It is typically measured in linear units, such as meters, feet, or inches. The perimeter of a shape can be calculated by adding up the lengths of all its sides.
Formulas for Calculating Perimeter
Here are some common formulas for calculating the perimeter of different shapes:
- Rectangle: Perimeter = 2 Γ (Length + Width)
- Triangle: Perimeter = Side1 + Side2 + Side3
- Circle: Perimeter = 2 Γ Ο Γ Radius
- Square: Perimeter = 4 Γ Side
π Note: The perimeter of a circle is also known as its circumference.
Practical Applications of Area and Perimeter
Understanding area and perimeter is crucial in various real-life scenarios, such as:
- Building design and construction
- Interior decorating and furniture arrangement
- Landscaping and gardening
- Engineering and architecture
For example, when designing a room, it is essential to calculate the area of the floor to determine the amount of flooring material needed. Similarly, when building a fence, it is necessary to calculate the perimeter of the yard to determine the amount of fencing material required.
Area and Perimeter Worksheet
Now that we have explored the concepts of area and perimeter, letβs practice with some exercises. Here is a worksheet with various problems to help you reinforce your understanding:
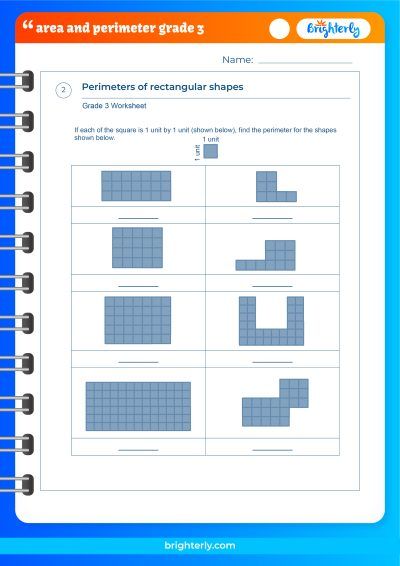
| Problem | Shape | Dimensions | Area/Perimeter |
|---|---|---|---|
| 1 | Rectangle | Length = 5m, Width = 3m | Area =? |
| 2 | Triangle | Base = 4m, Height = 6m | Area =? |
| 3 | Circle | Radius = 2m | Area =? |
| 4 | Square | Side = 4m | Perimeter =? |
| 5 | Rectangle | Length = 6m, Width = 2m | Perimeter =? |
Answers:
- 1. Area = 15m^2
- 2. Area = 12m^2
- 3. Area = 12.56m^2 (approximately)
- 4. Perimeter = 16m
- 5. Perimeter = 16m
In conclusion, understanding area and perimeter is essential in mathematics and has various practical applications. By practicing with worksheets and exercises, you can reinforce your understanding of these concepts and become more confident in your math skills.
What is the difference between area and perimeter?
+The area of a shape is the amount of space inside the shape, while the perimeter is the distance around the shape.
How do I calculate the area of a rectangle?
+The area of a rectangle is calculated by multiplying its length and width.
What is the formula for calculating the perimeter of a circle?
+The perimeter of a circle is calculated using the formula: Perimeter = 2 Γ Ο Γ Radius.
