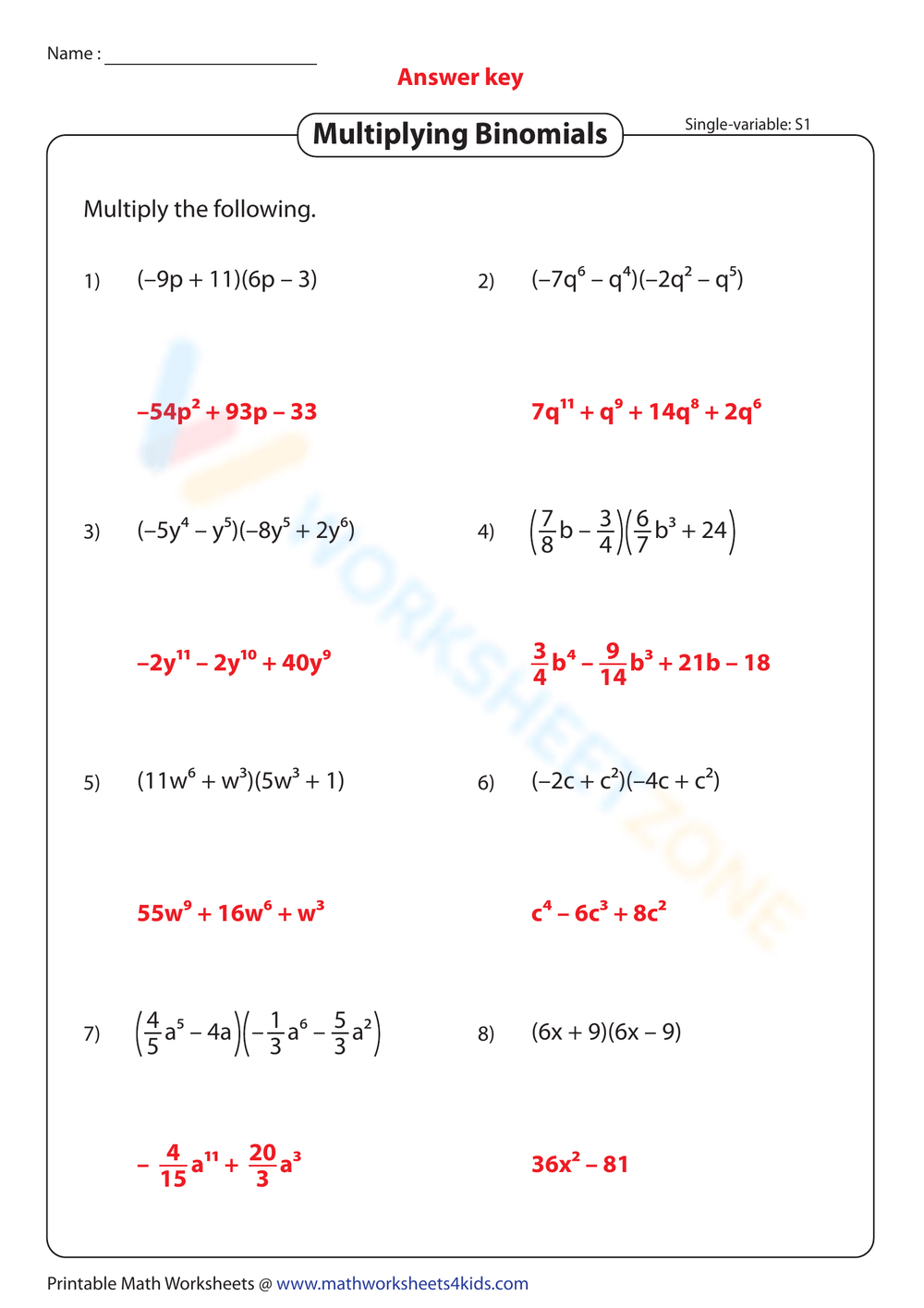
5 Easy Ways to Multiply Binomials
Mastering the Art of Multiplying Binomials: A Step-by-Step Guide
Multiplying binomials is a fundamental concept in algebra that can seem daunting at first, but with practice and the right techniques, it can become second nature. In this article, we will explore five easy ways to multiply binomials, making it easier for you to tackle even the most complex algebraic expressions.
What are Binomials?
Before we dive into the techniques, let’s define what binomials are. A binomial is an algebraic expression that consists of two terms, usually separated by a plus or minus sign. For example:
2x + 3
x^2 - 4
Binomials can be simple, as in the examples above, or more complex, involving multiple variables and coefficients.
Method 1: The FOIL Method
One of the most popular methods for multiplying binomials is the FOIL method. FOIL stands for First, Outer, Inner, Last, which refers to the order in which you multiply the terms.
Step-by-Step Instructions:
- Multiply the first terms of each binomial (First).
- Multiply the outer terms of each binomial (Outer).
- Multiply the inner terms of each binomial (Inner).
- Multiply the last terms of each binomial (Last).
- Combine like terms.
Example:
(2x + 3) × (x + 4)
- Multiply the first terms: 2x × x = 2x^2
- Multiply the outer terms: 2x × 4 = 8x
- Multiply the inner terms: 3 × x = 3x
- Multiply the last terms: 3 × 4 = 12
- Combine like terms: 2x^2 + 8x + 3x + 12 = 2x^2 + 11x + 12
Method 2: The Grid Method
The grid method is a visual approach to multiplying binomials. It involves creating a grid with the terms of each binomial and multiplying them accordingly.
Step-by-Step Instructions:
- Create a grid with the terms of each binomial.
- Multiply the terms in each cell of the grid.
- Combine like terms.
Example:
(2x + 3) × (x + 4)
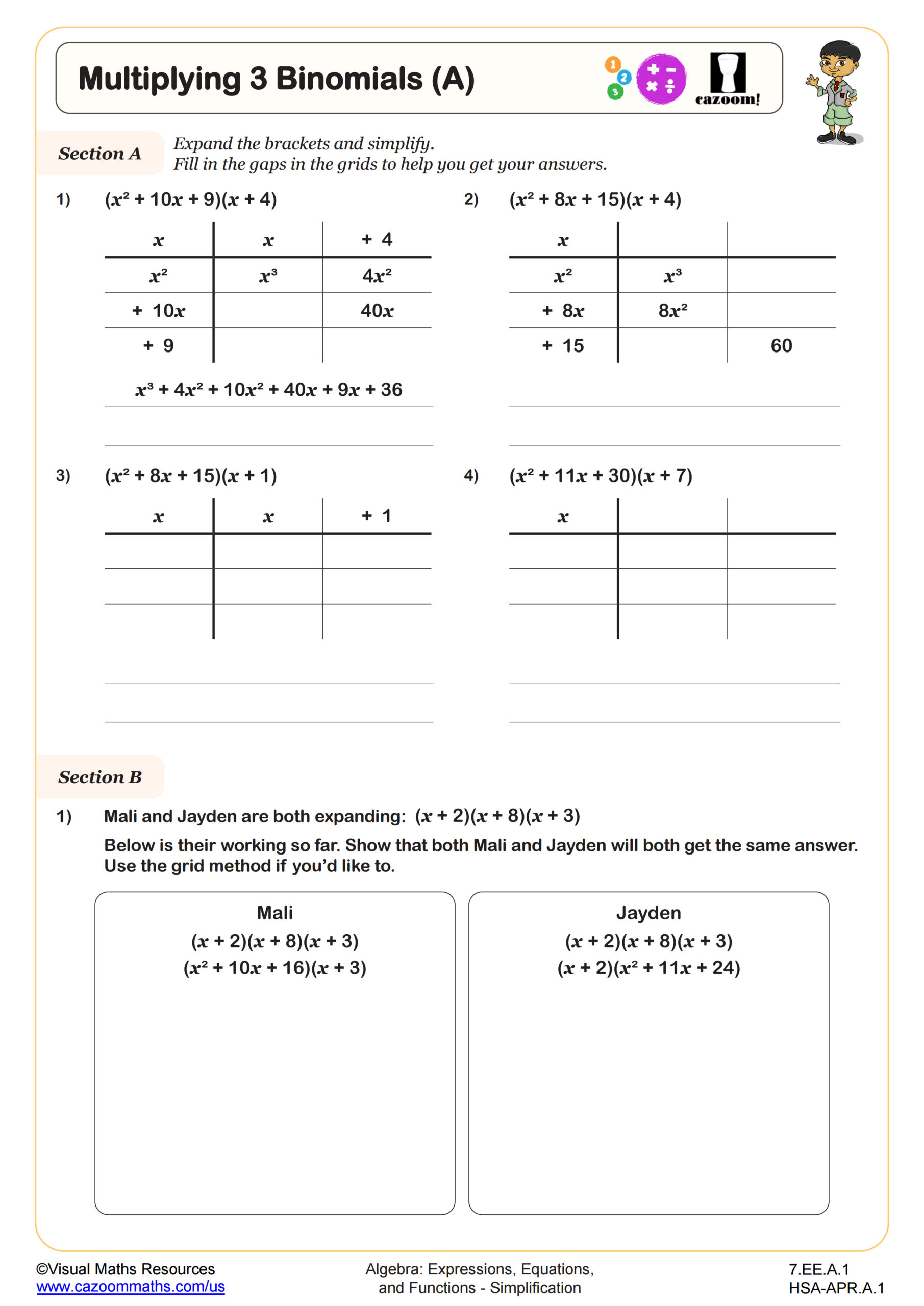
| x | 4 | |
|---|---|---|
| 2x | 2x^2 | 8x |
| 3 | 3x | 12 |
- Multiply the terms in each cell: 2x^2, 8x, 3x, 12
- Combine like terms: 2x^2 + 8x + 3x + 12 = 2x^2 + 11x + 12
Method 3: The Box Method
The box method is similar to the grid method, but it involves drawing a box around the terms of each binomial.
Step-by-Step Instructions:
- Draw a box around the terms of each binomial.
- Multiply the terms in each corner of the box.
- Combine like terms.
Example:
(2x + 3) × (x + 4)
[2x] [3] [—–] [x] [4]
- Multiply the terms in each corner: 2x^2, 8x, 3x, 12
- Combine like terms: 2x^2 + 8x + 3x + 12 = 2x^2 + 11x + 12
Method 4: The Column Method
The column method involves multiplying each term of one binomial by each term of the other binomial.
Step-by-Step Instructions:
- Multiply each term of one binomial by each term of the other binomial.
- Combine like terms.
Example:
(2x + 3) × (x + 4)
2x × x = 2x^2 2x × 4 = 8x 3 × x = 3x 3 × 4 = 12
- Combine like terms: 2x^2 + 8x + 3x + 12 = 2x^2 + 11x + 12
Method 5: The Calculator Method
If you have a calculator, you can use it to multiply binomials quickly and easily.
Step-by-Step Instructions:
- Enter the first binomial into your calculator.
- Enter the second binomial into your calculator.
- Multiply the two binomials using the multiplication function.
Example:
(2x + 3) × (x + 4)
Enter: (2x + 3) × (x + 4) Calculate: 2x^2 + 11x + 12
While the calculator method may seem like the easiest way to multiply binomials, it’s essential to understand the underlying math concepts to ensure accuracy and build a strong foundation in algebra.
[🤔] Note: It's essential to practice each method to become proficient in multiplying binomials.
In conclusion, multiplying binomials can be a straightforward process if you use the right techniques. Whether you prefer the FOIL method, grid method, box method, column method, or calculator method, with practice and patience, you’ll become a pro at multiplying binomials in no time.
What is the FOIL method?
+
The FOIL method is a technique for multiplying binomials that involves multiplying the First, Outer, Inner, and Last terms of each binomial.
How do I use the grid method to multiply binomials?
+
To use the grid method, create a grid with the terms of each binomial and multiply the terms in each cell. Then, combine like terms.
What is the box method?
+
The box method is a technique for multiplying binomials that involves drawing a box around the terms of each binomial and multiplying the terms in each corner.
Related Terms:
- Multiply Binomials Worksheet pdf
- Multiplying binomials and trinomials worksheet
- Multiplying Binomials Worksheet answer key