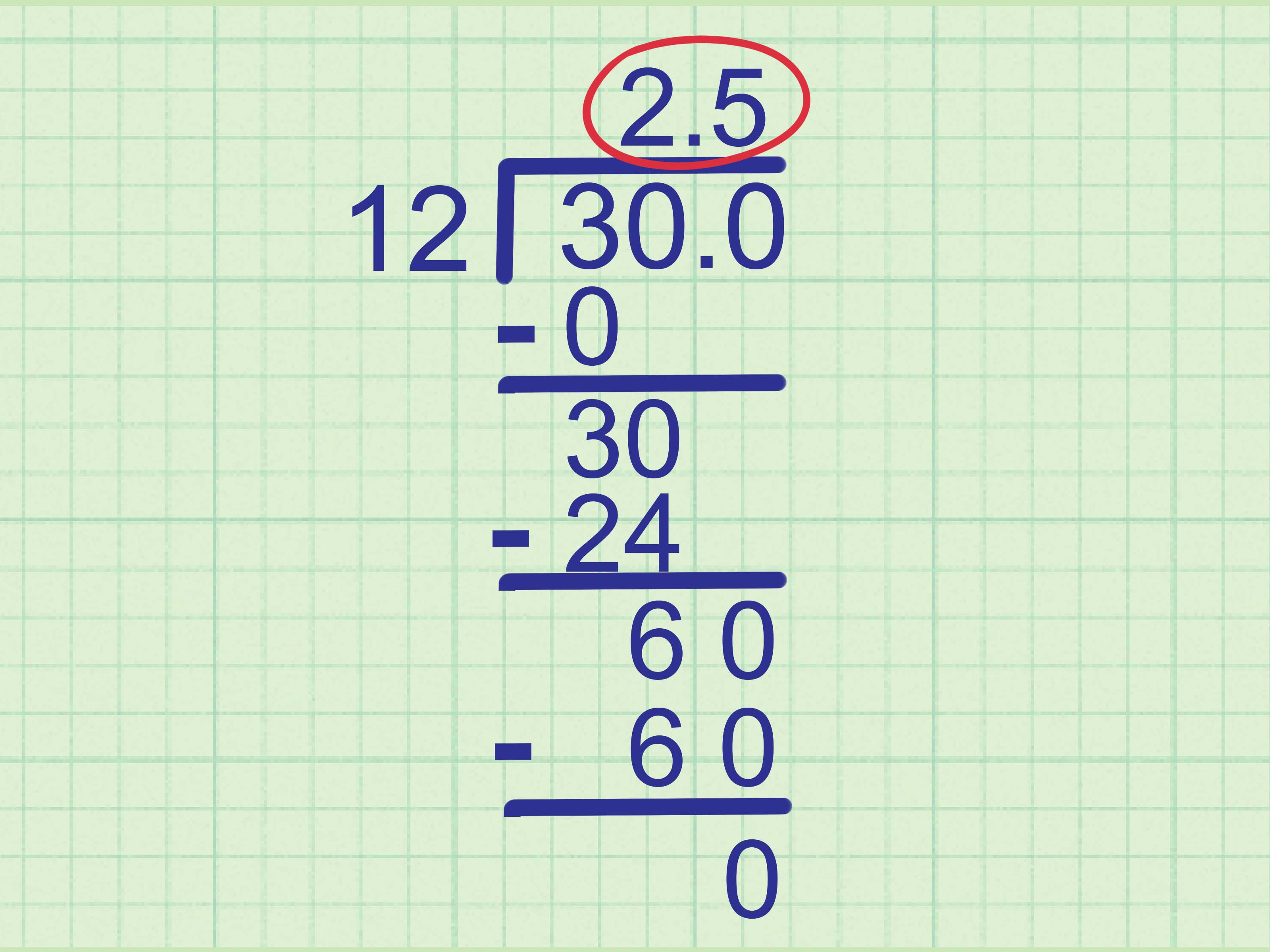Rational or Irrational Numbers Worksheet for Math Success
Rational and Irrational Numbers: Understanding the Basics
In mathematics, numbers are broadly classified into two categories: rational and irrational numbers. Understanding the difference between these two types of numbers is crucial for success in math. In this article, we will delve into the world of rational and irrational numbers, explore their definitions, and provide a comprehensive worksheet to help you practice and reinforce your understanding.
What are Rational Numbers?
Rational numbers are numbers that can be expressed as the ratio of two integers, i.e., a fraction. They can be written in the form a/b, where a and b are integers and b is non-zero. Rational numbers can be further divided into two subcategories: integers and non-integers.
Examples of Rational Numbers:
- Integers: 5, -3, 0
- Non-integers: 3⁄4, 22⁄7, -5⁄2
What are Irrational Numbers?
Irrational numbers are numbers that cannot be expressed as the ratio of two integers. They are numbers that have an infinite number of digits after the decimal point, and these digits never repeat in a predictable pattern. Irrational numbers cannot be written in the form a/b, where a and b are integers and b is non-zero.
Examples of Irrational Numbers:
- π (pi): 3.14159… (infinite non-repeating digits)
- e (Euler’s number): 2.71828… (infinite non-repeating digits)
- √2 (square root of 2): 1.41421… (infinite non-repeating digits)
Key Differences between Rational and Irrational Numbers

| Rational Numbers | Irrational Numbers | |
|---|---|---|
| Definition | Can be expressed as a ratio of two integers | Cannot be expressed as a ratio of two integers |
| Form | a/b, where a and b are integers and b is non-zero | Infinite non-repeating digits after the decimal point |
| Examples | 3⁄4, 22⁄7, -5⁄2 | π, e, √2 |
📝 Note: All integers are rational numbers, but not all rational numbers are integers.
Worksheet: Rational and Irrational Numbers
Now that we have covered the basics of rational and irrational numbers, it’s time to put your knowledge into practice. Here’s a comprehensive worksheet to help you reinforce your understanding:
Section 1: Multiple Choice Questions
- Which of the following numbers is rational? a) 5 b) π c) √2 d) -3⁄4
Answer: a) 5
- Which of the following numbers is irrational? a) 3⁄4 b) 22⁄7 c) π d) -5⁄2
Answer: c) π
Section 2: Short Answer Questions
- Write the decimal expansion of the rational number 3⁄4.
Answer: 0.75
- Identify whether the number 2.71828… is rational or irrational.
Answer: Irrational
Section 3: Fill in the Blanks
- The number _______________________ is an example of a rational number.
Answer: 3⁄4
- The number _______________________ is an example of an irrational number.
Answer: π
Section 4: True or False
All integers are irrational numbers. (False)
The number 22⁄7 is a rational number. (True)
Conclusion
Understanding the difference between rational and irrational numbers is essential for success in math. By practicing with the worksheet provided, you can reinforce your knowledge and develop a deeper understanding of these two types of numbers. Remember, rational numbers can be expressed as a ratio of two integers, while irrational numbers have an infinite number of digits after the decimal point that never repeat in a predictable pattern.
What is the difference between a rational and an irrational number?
+
Rational numbers can be expressed as the ratio of two integers, while irrational numbers have an infinite number of digits after the decimal point that never repeat in a predictable pattern.
Can all integers be expressed as rational numbers?
+
Yes, all integers can be expressed as rational numbers. For example, the integer 5 can be written as 5⁄1.
Is the number π a rational or irrational number?
+
The number π is an irrational number. It has an infinite number of digits after the decimal point that never repeat in a predictable pattern.
Related Terms:
- Rational or irrational Worksheet PDF
- Rational and irrational numbers practice