5 Ways to Master Multiplication with Distributive Property
Multiplication can be a challenging concept for many students to grasp, but one powerful tool to help master it is the distributive property. The distributive property is a fundamental concept in mathematics that can simplify multiplication problems and make them more manageable. In this post, we will explore five ways to apply the distributive property to master multiplication.
Understanding the Distributive Property
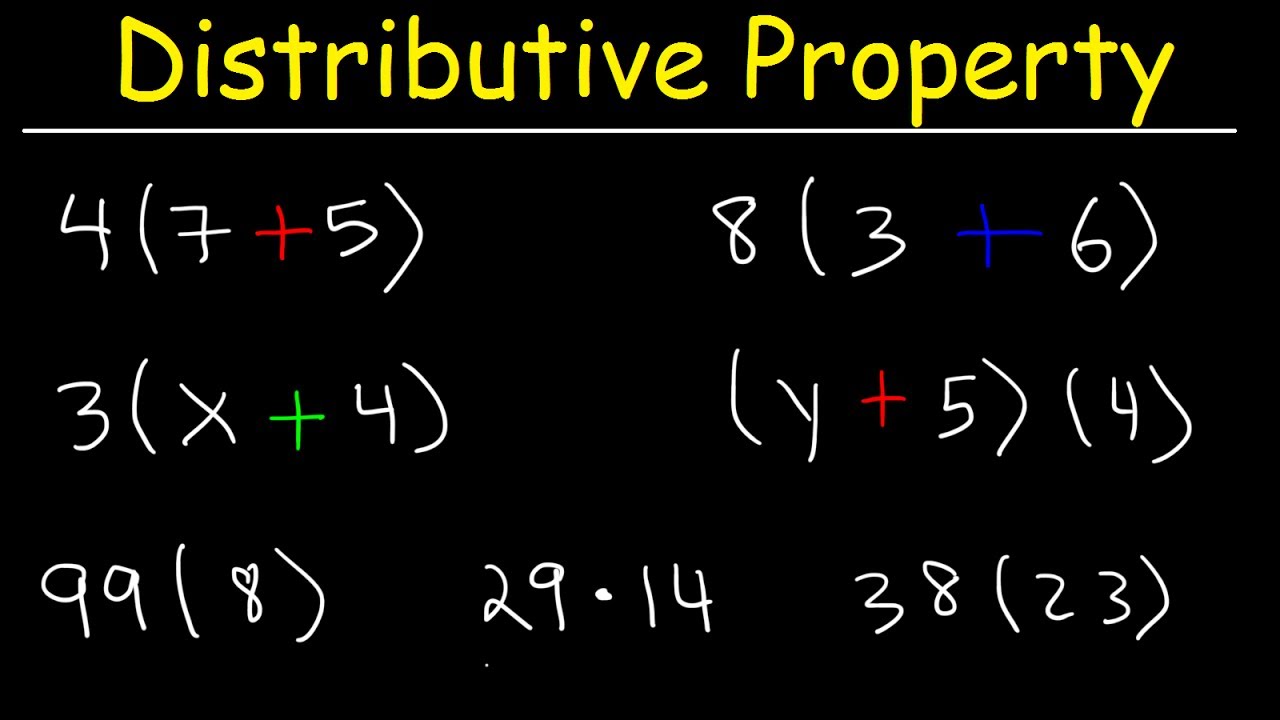
The distributive property states that a single term can be distributed across multiple terms inside parentheses. In mathematical terms, it can be expressed as:
a(b + c) = a*b + a*c
This property allows us to break down complex multiplication problems into simpler ones, making it easier to calculate the product.
1. Breaking Down Multiplication Problems
One of the most common ways to apply the distributive property is to break down multiplication problems into smaller parts. For example, consider the problem:
4 × (6 + 3)
Using the distributive property, we can break this problem down into two smaller multiplication problems:
4 × 6 = 24 4 × 3 = 12
Adding the results together, we get:
24 + 12 = 36
Therefore, 4 × (6 + 3) = 36.
2. Simplifying Multiplication with Variables
The distributive property can also be used to simplify multiplication problems involving variables. For example, consider the problem:
2x × (3x + 4)
Using the distributive property, we can distribute the 2x across the terms inside the parentheses:
2x × 3x = 6x^2 2x × 4 = 8x
Combining the results, we get:
6x^2 + 8x
Therefore, 2x × (3x + 4) = 6x^2 + 8x.
3. Solving Multi-Digit Multiplication Problems
The distributive property can also be used to solve multi-digit multiplication problems. For example, consider the problem:
43 × 27
Using the distributive property, we can break this problem down into smaller parts:
40 × 27 = 1080 3 × 27 = 81
Adding the results together, we get:
1080 + 81 = 1161
Therefore, 43 × 27 = 1161.
4. Applying the Distributive Property to Word Problems
The distributive property can also be applied to word problems involving multiplication. For example, consider the problem:
Tom has 3 groups of 4 boxes, and each box contains 6 pencils. How many pencils does Tom have in total?
Using the distributive property, we can break this problem down into smaller parts:
3 × 4 = 12 (groups of boxes) 12 × 6 = 72 (pencils)
Therefore, Tom has 72 pencils in total.
5. Using the Distributive Property to Check Answers
Finally, the distributive property can be used to check answers to multiplication problems. For example, consider the problem:
6 × 9 =?
Using the distributive property, we can break this problem down into smaller parts:
6 × (5 + 4) = 6 × 5 + 6 × 4 = 30 + 24 = 54
Therefore, 6 × 9 = 54.
In conclusion, mastering the distributive property is a powerful way to simplify multiplication problems and make them more manageable. By breaking down complex problems into smaller parts, simplifying multiplication with variables, solving multi-digit multiplication problems, applying the distributive property to word problems, and using it to check answers, students can become more confident and proficient in their multiplication skills.
What is the distributive property?
+
The distributive property is a mathematical concept that states that a single term can be distributed across multiple terms inside parentheses. It can be expressed as: a(b + c) = a*b + a*c.
How can the distributive property be used to simplify multiplication problems?
+
The distributive property can be used to simplify multiplication problems by breaking them down into smaller parts, making it easier to calculate the product.
Can the distributive property be applied to word problems?
+
Yes, the distributive property can be applied to word problems involving multiplication. It can help to break down complex problems into smaller parts, making it easier to solve.