5 Ways to Master Multiplication with Decimals
Mastering Multiplication with Decimals: A Comprehensive Guide
Multiplication with decimals can be a daunting task for many students, but with the right approach, it can be mastered with ease. In this article, we will explore five effective ways to master multiplication with decimals. Whether you’re a student struggling with decimal multiplication or a teacher looking for new ways to teach this concept, this guide is for you.
Understanding Decimal Multiplication
Before we dive into the methods, it’s essential to understand the basics of decimal multiplication. When multiplying decimals, we need to multiply the numbers as if they were whole numbers, and then place the decimal point in the correct position.
The Rule:
- Multiply the numbers as if they were whole numbers.
- Count the total number of decimal places in both numbers.
- Place the decimal point in the product so that it has the same number of decimal places as the sum of the decimal places in both numbers.
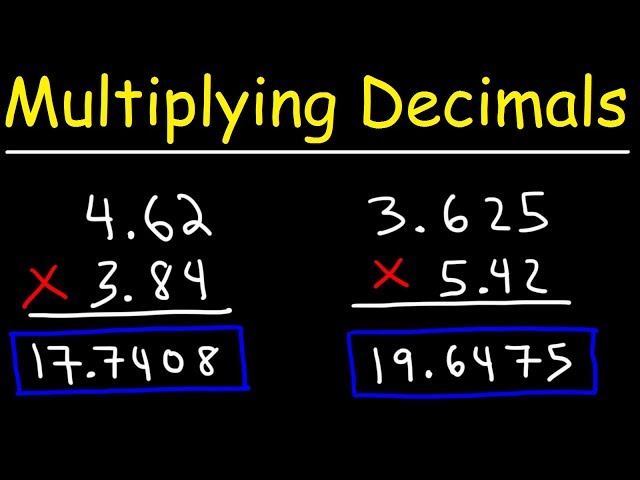
Method 1: Using the Standard Algorithm
The standard algorithm for multiplying decimals is the most straightforward method. It involves multiplying the numbers as if they were whole numbers and then placing the decimal point in the correct position.
Example:
Multiply 2.5 and 3.8
- Multiply 25 and 38 (treating them as whole numbers) = 950
- Count the total number of decimal places in both numbers: 2.5 has 1 decimal place, and 3.8 has 1 decimal place, so the product should have 2 decimal places.
- Place the decimal point in the product: 9.50
📝 Note: When using the standard algorithm, make sure to line up the decimal points correctly to avoid errors.
Method 2: Using the Grid Method
The grid method is a visual approach to multiplying decimals. It involves creating a grid with the numbers and then multiplying them in parts.
Example:
Multiply 2.5 and 3.8
- Create a grid with 2.5 and 3.8
- Multiply 2 and 3 = 6
- Multiply 2 and 8 = 16
- Multiply 5 and 3 = 15
- Multiply 5 and 8 = 40
- Add up the partial products: 6 + 16 + 15 + 40 = 77
- Place the decimal point in the product: 9.50
📝 Note: The grid method can be helpful for visual learners, but it can be time-consuming for larger numbers.
Method 3: Using the Lattice Method
The lattice method is another visual approach to multiplying decimals. It involves creating a lattice with the numbers and then multiplying them in parts.
Example:
Multiply 2.5 and 3.8
- Create a lattice with 2.5 and 3.8
- Multiply 2 and 3 = 6
- Multiply 2 and 8 = 16
- Multiply 5 and 3 = 15
- Multiply 5 and 8 = 40
- Add up the partial products: 6 + 16 + 15 + 40 = 77
- Place the decimal point in the product: 9.50
📝 Note: The lattice method is similar to the grid method, but it can be more efficient for larger numbers.
Method 4: Using Mental Math
Mental math is a quick and efficient way to multiply decimals. It involves using multiplication facts and estimation to calculate the product.
Example:
Multiply 2.5 and 3.8
- Use the multiplication fact: 2 x 3 = 6
- Estimate the product: 6 x 3.8 ≈ 22.8
- Adjust the estimate: 2.5 x 3.8 ≈ 9.50
📝 Note: Mental math requires practice and fluency with multiplication facts, but it can be a powerful tool for multiplying decimals.
Method 5: Using Technology
Technology can be a useful tool for multiplying decimals. Calculators and online tools can quickly and accurately calculate the product.
Example:
Multiply 2.5 and 3.8 using a calculator
- Enter the numbers into the calculator: 2.5 x 3.8
- Press the equal sign: 9.50
📝 Note: Technology can be a helpful tool, but it's essential to understand the underlying math concepts to use it effectively.
In conclusion, mastering multiplication with decimals requires practice, patience, and persistence. By using the standard algorithm, grid method, lattice method, mental math, and technology, you can become proficient in multiplying decimals. Remember to practice regularly and apply these methods to different types of problems to reinforce your understanding.
What is the rule for multiplying decimals?
+
Multiply the numbers as if they were whole numbers, and then place the decimal point in the correct position by counting the total number of decimal places in both numbers.
What is the difference between the grid method and the lattice method?
+
The grid method and the lattice method are both visual approaches to multiplying decimals, but the lattice method can be more efficient for larger numbers.
Can I use technology to multiply decimals?
+
Yes, technology such as calculators and online tools can be used to quickly and accurately multiply decimals.
Related Terms:
- Adding decimals worksheet
- Decimal worksheet
- Decimals Worksheet grade 5 pdf
- Fraction to decimal Worksheet
- Fraction Worksheet Grade 5