Solving Multi Step Equations Made Easy for Students

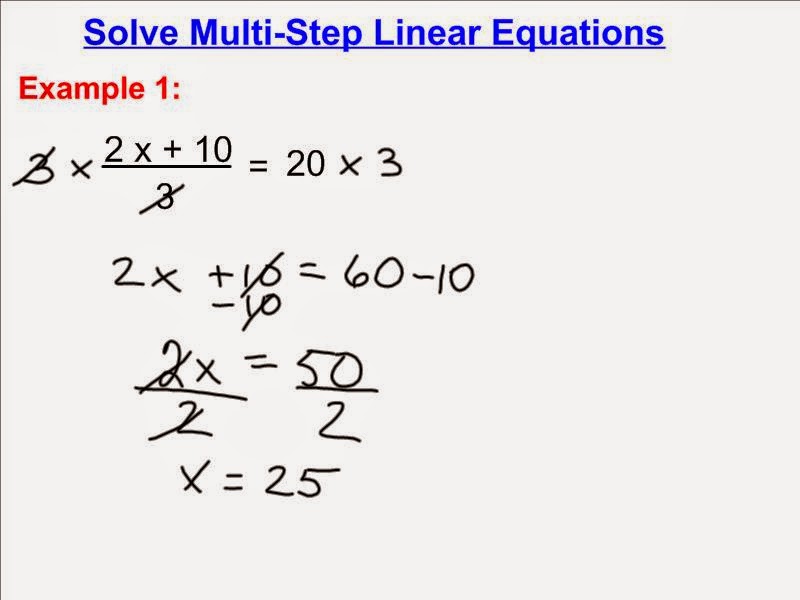
Understanding the Basics of Multi-Step Equations

Multi-step equations are a fundamental concept in algebra, and they can be intimidating for many students. However, with a clear understanding of the basics and a step-by-step approach, solving these equations can become a breeze. In this article, we will break down the process of solving multi-step equations, providing you with the tools and confidence you need to tackle even the most complex problems.

What are Multi-Step Equations?

Multi-step equations are algebraic equations that require more than one step to solve. They involve a combination of addition, subtraction, multiplication, and division operations, and often require the use of inverse operations to isolate the variable. These equations can be linear or quadratic, and may involve fractions, decimals, or integers.

Example of a Multi-Step Equation:

2x + 5 = 11

This equation requires multiple steps to solve, as we need to isolate the variable x. We will use a combination of subtraction and division operations to solve for x.

Step-by-Step Guide to Solving Multi-Step Equations

Solving multi-step equations involves a systematic approach. Here are the steps to follow:

1. Read and Understand the Equation: Read the equation carefully, identifying the variable and the constants. Understand what operations are being performed and what the equation is asking you to solve for.
2. Simplify the Equation: Simplify the equation by combining like terms and performing any necessary operations. This will help you to focus on the variable and the constants.
3. Use Inverse Operations: Use inverse operations to isolate the variable. Inverse operations are operations that “undo” each other, such as addition and subtraction, or multiplication and division.
4. Check Your Solution: Once you have solved for the variable, check your solution by plugging it back into the original equation.

Example Solution:

2x + 5 = 11

Step 1: Read and Understand the Equation

We are solving for the variable x. The equation involves addition and multiplication operations.

Step 2: Simplify the Equation

Subtract 5 from both sides of the equation:

2x = 11 - 5 2x = 6

Step 3: Use Inverse Operations

Divide both sides of the equation by 2:

x = 6 ÷ 2 x = 3

Step 4: Check Your Solution

Plug x = 3 back into the original equation:

2(3) + 5 = 11 6 + 5 = 11 11 = 11

Our solution is correct!

Common Challenges and Tips

• Distributive Property: When dealing with equations involving the distributive property, make sure to distribute the operation to all terms inside the parentheses.
• Order of Operations: Always follow the order of operations (PEMDAS) when simplifying and solving equations.
• Inverse Operations: Use inverse operations to isolate the variable. Remember that addition and subtraction are inverse operations, as are multiplication and division.
• Check Your Work: Always check your solution by plugging it back into the original equation.

💡 Note: When solving multi-step equations, it's essential to be patient and take your time. Break down the equation into smaller, manageable steps, and use inverse operations to isolate the variable.

Real-World Applications of Multi-Step Equations

Multi-step equations have numerous real-world applications in fields such as science, engineering, economics, and finance. They are used to model complex systems, make predictions, and optimize processes.

Example Application:

A company is producing a new product, and they need to determine the cost of production. The equation they use is:

2x + 500 = 2000

Where x is the number of units produced. By solving this equation, the company can determine the cost of production and make informed decisions about pricing and production levels.

Conclusion

Solving multi-step equations requires a step-by-step approach, using inverse operations to isolate the variable. By understanding the basics and following these steps, you can become proficient in solving even the most complex multi-step equations. Remember to be patient, take your time, and always check your solution.