Mixed Numbers and Improper Fractions Made Easy
Mixed Numbers and Improper Fractions Made Easy
Working with fractions can be a challenging task for many students, especially when it comes to mixed numbers and improper fractions. However, with the right approach and techniques, understanding and working with these concepts can become much easier. In this article, we will explore the world of mixed numbers and improper fractions, and provide you with a comprehensive guide on how to work with them.
What are Mixed Numbers?
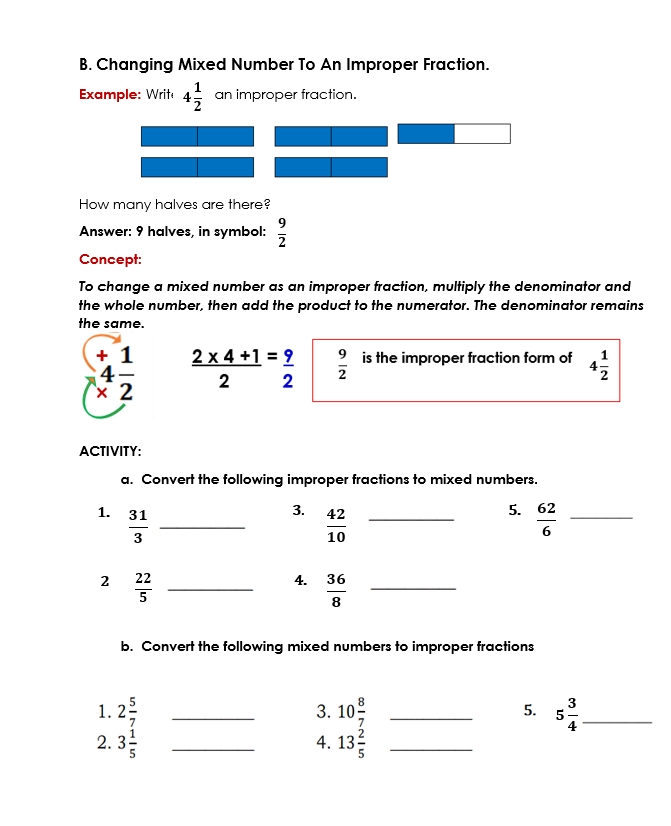
A mixed number is a combination of a whole number and a fraction. It is used to represent a value that is greater than a whole number but less than the next whole number. For example, 2 1⁄2 is a mixed number, where 2 is the whole number and 1⁄2 is the fraction.
What are Improper Fractions?
An improper fraction is a fraction where the numerator is greater than the denominator. In other words, it is a fraction that represents a value that is greater than a whole number. For example, 3⁄2 is an improper fraction, where 3 is the numerator and 2 is the denominator.
Converting Mixed Numbers to Improper Fractions
Converting mixed numbers to improper fractions is a simple process. Here are the steps:
- Multiply the whole number by the denominator
- Add the numerator to the result
- Write the result as an improper fraction
For example, let’s convert the mixed number 2 1⁄2 to an improper fraction:
- Multiply the whole number (2) by the denominator (2): 2 x 2 = 4
- Add the numerator (1) to the result: 4 + 1 = 5
- Write the result as an improper fraction: 5⁄2
📝 Note: When converting mixed numbers to improper fractions, make sure to multiply the whole number by the denominator, not the numerator.
Converting Improper Fractions to Mixed Numbers
Converting improper fractions to mixed numbers is also a simple process. Here are the steps:
- Divide the numerator by the denominator
- Write the result as a whole number and a remainder
- Write the remainder as a fraction
For example, let’s convert the improper fraction 5⁄2 to a mixed number:
- Divide the numerator (5) by the denominator (2): 5 ÷ 2 = 2 with a remainder of 1
- Write the result as a whole number and a remainder: 2 1⁄2
📝 Note: When converting improper fractions to mixed numbers, make sure to divide the numerator by the denominator, not the other way around.
Adding and Subtracting Mixed Numbers and Improper Fractions
Adding and subtracting mixed numbers and improper fractions can be a bit tricky, but with the right approach, it can be made easy. Here are the steps:
- Convert both numbers to improper fractions
- Add or subtract the numerators
- Keep the denominator the same
- Simplify the result, if possible
For example, let’s add the mixed numbers 2 1⁄2 and 1 3⁄4:
- Convert both numbers to improper fractions: 2 1⁄2 = 5⁄2 and 1 3⁄4 = 7⁄4
- Add the numerators: 5 + 7 = 12
- Keep the denominator the same: 12⁄4
- Simplify the result: 12⁄4 = 3
Multiplying and Dividing Mixed Numbers and Improper Fractions
Multiplying and dividing mixed numbers and improper fractions can be a bit more complex, but with the right approach, it can be made easy. Here are the steps:
- Convert both numbers to improper fractions
- Multiply or divide the numerators and denominators
- Simplify the result, if possible
For example, let’s multiply the mixed numbers 2 1⁄2 and 1 3⁄4:
- Convert both numbers to improper fractions: 2 1⁄2 = 5⁄2 and 1 3⁄4 = 7⁄4
- Multiply the numerators and denominators: (5 x 7) / (2 x 4) = 35⁄8
- Simplify the result: 35⁄8 = 4 3⁄8
📝 Note: When multiplying and dividing mixed numbers and improper fractions, make sure to follow the order of operations (PEMDAS).
Conclusion
Working with mixed numbers and improper fractions can be challenging, but with the right approach and techniques, it can be made easy. By following the steps outlined in this article, you can convert mixed numbers to improper fractions, convert improper fractions to mixed numbers, add, subtract, multiply, and divide mixed numbers and improper fractions. Remember to always follow the order of operations and simplify your results, if possible.
What is the difference between a mixed number and an improper fraction?
+A mixed number is a combination of a whole number and a fraction, while an improper fraction is a fraction where the numerator is greater than the denominator.
How do I convert a mixed number to an improper fraction?
+To convert a mixed number to an improper fraction, multiply the whole number by the denominator, add the numerator to the result, and write the result as an improper fraction.
How do I add and subtract mixed numbers and improper fractions?
+To add and subtract mixed numbers and improper fractions, convert both numbers to improper fractions, add or subtract the numerators, keep the denominator the same, and simplify the result, if possible.
Related Terms:
- Subtracting fractions Worksheet