Convert Mixed Numbers to Improper Fractions in 5 Steps
Converting Mixed Numbers to Improper Fractions: A Step-by-Step Guide
In mathematics, fractions are used to represent a part of a whole. There are different types of fractions, including proper fractions, improper fractions, and mixed numbers. In this article, we will focus on converting mixed numbers to improper fractions. We will provide a step-by-step guide on how to do this conversion.
What are Mixed Numbers and Improper Fractions?
Before we dive into the conversion process, let’s first understand what mixed numbers and improper fractions are.
- Mixed Numbers: A mixed number is a combination of a whole number and a proper fraction. For example, 2 1⁄3 is a mixed number.
- Improper Fractions: An improper fraction is a fraction where the numerator is greater than or equal to the denominator. For example, 3⁄2 is an improper fraction.
Converting Mixed Numbers to Improper Fractions: A 5-Step Process
Now that we understand what mixed numbers and improper fractions are, let’s move on to the conversion process. Here are the steps to convert a mixed number to an improper fraction:
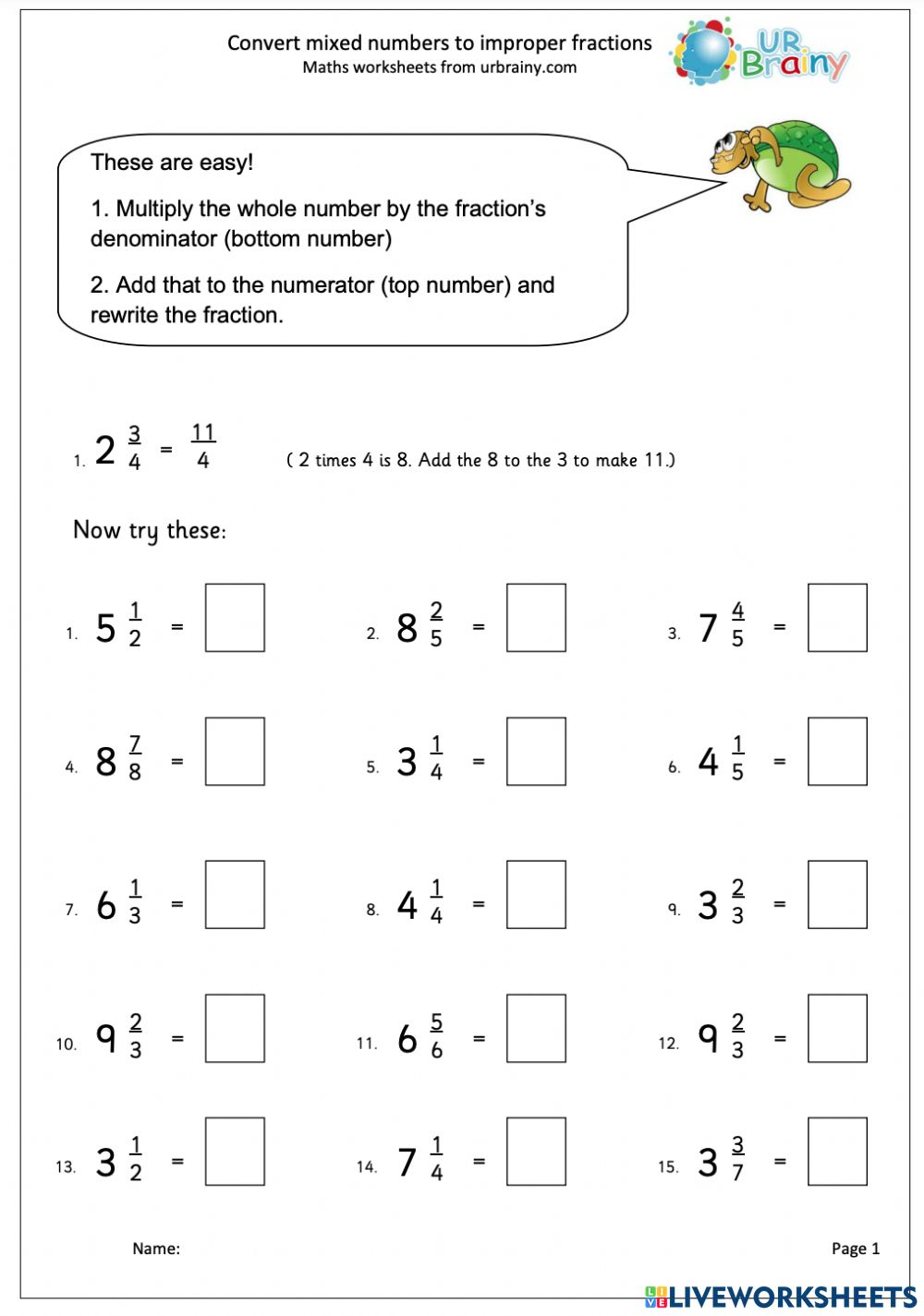
Step 1: Multiply the Whole Number by the Denominator
Take the whole number part of the mixed number and multiply it by the denominator. For example, if we have the mixed number 2 1⁄3, we multiply 2 by 3, which gives us 6.
Step 2: Add the Numerator to the Product
Take the product from step 1 and add the numerator to it. Using the same example, we add 1 to 6, which gives us 7.
Step 3: Write the Result as an Improper Fraction
Take the result from step 2 and write it as an improper fraction by placing it over the original denominator. Using the same example, we write 7⁄3.
Step 4: Simplify the Improper Fraction (Optional)
If the improper fraction can be simplified, simplify it by dividing both the numerator and denominator by their greatest common divisor (GCD). Using the same example, 7⁄3 cannot be simplified further.
Step 5: Check Your Answer
Finally, check your answer by converting the improper fraction back to a mixed number to ensure that it is correct.
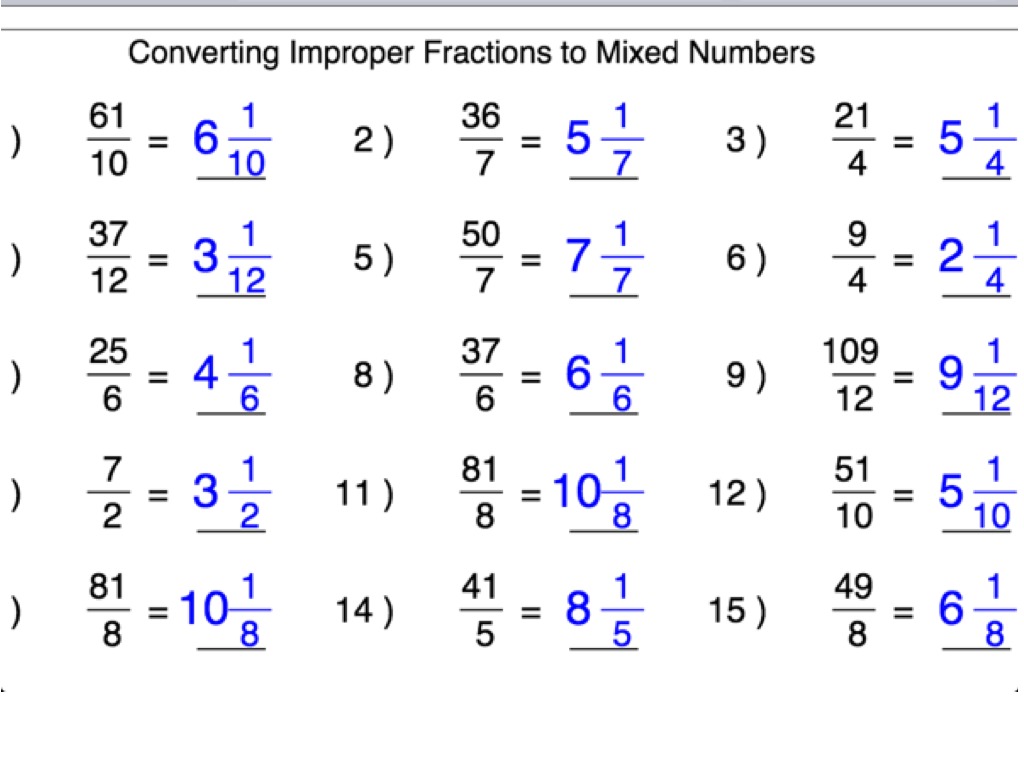
| Mixed Number | Improper Fraction |
|---|---|
| 2 1/3 | 7/3 |
| 3 2/5 | 17/5 |
| 1 3/4 | 7/4 |
📝 Note: It's essential to check your answer to ensure that the conversion is correct.
The ability to convert mixed numbers to improper fractions is a fundamental math skill. By following these steps, you can easily convert mixed numbers to improper fractions.
What is the difference between a mixed number and an improper fraction?
+A mixed number is a combination of a whole number and a proper fraction, while an improper fraction is a fraction where the numerator is greater than or equal to the denominator.
Why is it essential to check your answer when converting mixed numbers to improper fractions?
+It's crucial to check your answer to ensure that the conversion is correct. This helps to avoid errors and ensures that the improper fraction accurately represents the original mixed number.
Can all mixed numbers be converted to improper fractions?
+Yes, all mixed numbers can be converted to improper fractions using the steps outlined in this article.
To summarize, converting mixed numbers to improper fractions is a straightforward process that involves multiplying the whole number by the denominator, adding the numerator to the product, and writing the result as an improper fraction. By following these steps and checking your answer, you can accurately convert mixed numbers to improper fractions.