Mean Median Mode And Range Worksheets

Understanding the Basics of Statistics: Mean, Median, Mode, and Range
Statistics is a branch of mathematics that deals with the collection, analysis, interpretation, presentation, and organization of data. In this article, we will focus on four fundamental concepts in statistics: mean, median, mode, and range. These concepts are essential in understanding and working with data, and are commonly used in various fields such as business, economics, social sciences, and more.
What is Mean?
The mean, also known as the average, is a measure of the central tendency of a set of numbers. It is calculated by adding up all the numbers in the dataset and dividing by the total number of values. The mean is sensitive to extreme values, also known as outliers, which can affect the accuracy of the mean.
Formula for Mean:
mean = (sum of all values) / (total number of values)
Example:
Find the mean of the following set of numbers: 2, 4, 6, 8, 10
mean = (2 + 4 + 6 + 8 + 10) / 5 mean = 30 / 5 mean = 6
What is Median?
The median is another measure of central tendency, which is the middle value of a dataset when it is arranged in order. If the dataset has an even number of values, the median is the average of the two middle values.
Example:
Find the median of the following set of numbers: 1, 3, 5, 7, 9
Median = 5 (since it is the middle value)
What is Mode?
The mode is the value that appears most frequently in a dataset. A dataset can have multiple modes if there are multiple values that appear with the same frequency.
Example:
Find the mode of the following set of numbers: 2, 4, 4, 6, 4, 8
Mode = 4 (since it appears most frequently)
What is Range?
The range is the difference between the largest and smallest values in a dataset. It is a measure of dispersion, which indicates how spread out the data is.
Formula for Range:
range = (largest value) - (smallest value)
Example:
Find the range of the following set of numbers: 2, 4, 6, 8, 10
range = 10 - 2 range = 8
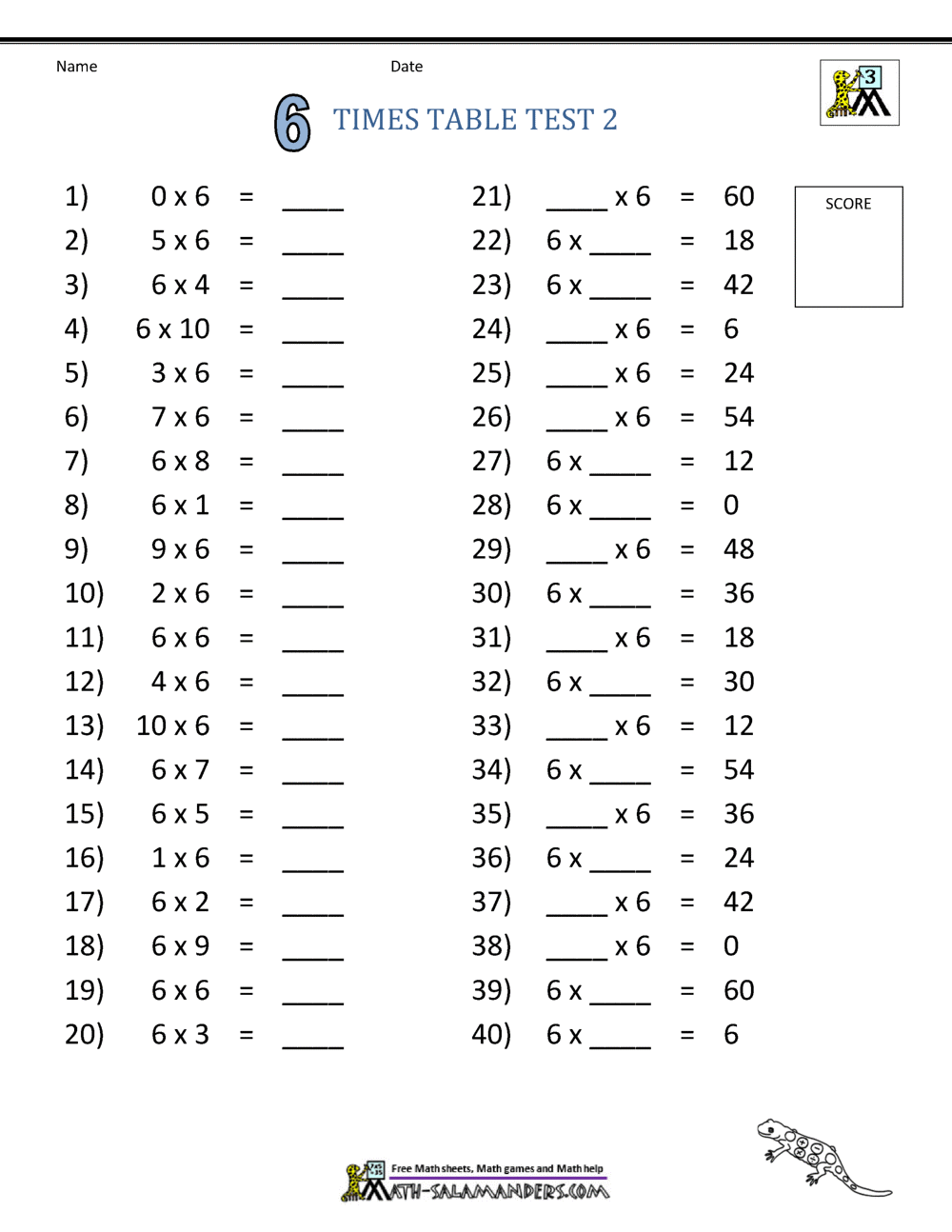
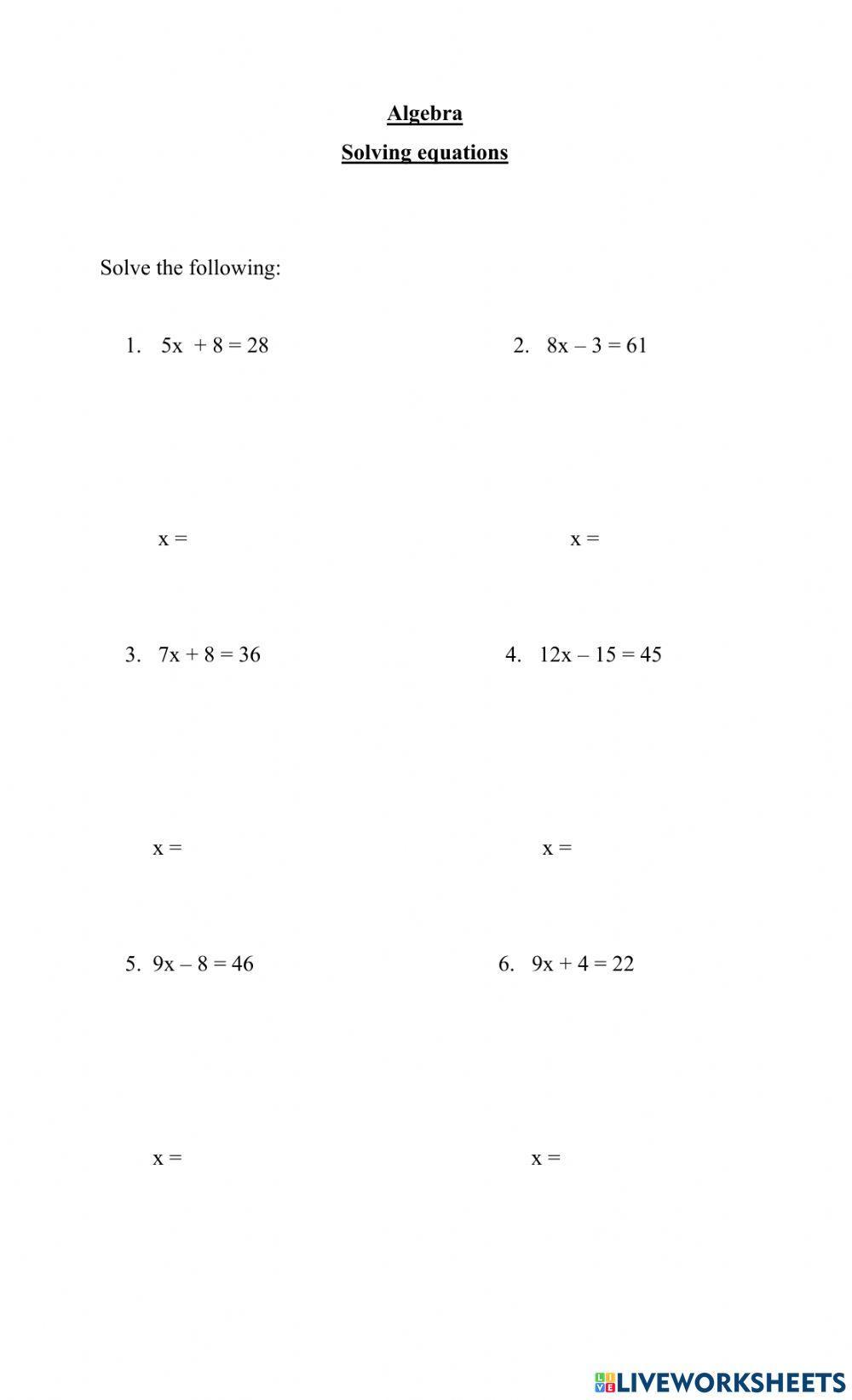
Worksheets and Exercises
To help you practice and understand these concepts better, here are some worksheets and exercises:
Worksheet 1:
Find the mean, median, mode, and range of the following datasets:

| Dataset | Mean | Median | Mode | Range |
|---|---|---|---|---|
| 1, 2, 3, 4, 5 | ||||
| 2, 4, 6, 8, 10 | ||||
| 1, 3, 5, 7, 9 |
Worksheet 2:
A basketball team scored the following points in five games: 80, 70, 90, 85, 75. Find the mean, median, mode, and range of the scores.
Worksheet 3:
A student scored the following grades in five subjects: 80, 70, 90, 85, 75. Find the mean, median, mode, and range of the grades.
Tips and Notes
- Always arrange the data in order before finding the median.
- The mode can be affected by outliers, so it’s essential to check for outliers before finding the mode.
- The range is sensitive to extreme values, so it’s essential to check for outliers before finding the range.
Conclusion
In conclusion, understanding the mean, median, mode, and range is essential in statistics and data analysis. These concepts help us to summarize and describe datasets, and are used in various fields such as business, economics, social sciences, and more. By practicing with worksheets and exercises, you can improve your understanding of these concepts and become proficient in working with data.
FAQs
What is the difference between mean and median?
+The mean is the average of all values, while the median is the middle value of a dataset when it is arranged in order.
Can a dataset have multiple modes?
+Yes, a dataset can have multiple modes if there are multiple values that appear with the same frequency.
What is the range of a dataset?
+The range is the difference between the largest and smallest values in a dataset.