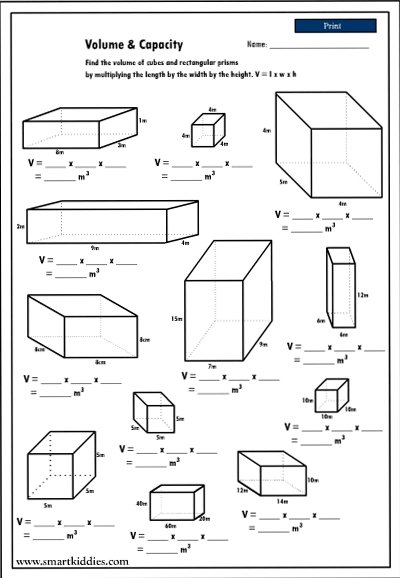
5 Ways to Master Long Division with No Remainders
Understanding the Basics of Long Division
Long division is a fundamental math operation that can be daunting for many students. However, with the right approach, it can be mastered with ease. In this article, we will focus on long division with no remainders, which means that the divisor will divide the dividend exactly without leaving any remainder.
Method 1: Using the Standard Long Division Algorithm
The standard long division algorithm is the most common method used to perform long division. Here’s a step-by-step guide to using this method:
- Write the dividend (the number being divided) on top of a line, and the divisor (the number by which we are dividing) below it.
- Divide the first digit of the dividend by the divisor, and write the result below the line.
- Multiply the result by the divisor, and subtract the product from the dividend.
- Bring down the next digit of the dividend, and repeat the process until the dividend is reduced to zero.
Example: 48 ÷ 6 =?

| 6 | 4 8 | |
|---|---|---|
| × | 6 0 | |
| - | 4 8 | |
| 8 0 | ||
| × | 6 0 | |
| - | 8 0 | |
| 0 0 |
Result: 8
📝 Note: Make sure to line up the numbers correctly and perform the multiplication and subtraction steps accurately.
Method 2: Using the Partial Quotients Method
The partial quotients method is another way to perform long division. This method involves breaking down the dividend into smaller parts and finding the partial quotients.
- Write the dividend and divisor as before.
- Divide the first digit of the dividend by the divisor, and write the result below the line.
- Multiply the result by the divisor, and subtract the product from the dividend.
- Bring down the next digit of the dividend, and repeat the process until the dividend is reduced to zero.
Example: 48 ÷ 6 =?
| 6 | 4 8 | |
|---|---|---|
| × | 4 0 | |
| - | 4 8 | |
| 4 8 | ||
| × | 4 0 | |
| - | 4 8 | |
| 0 0 |
Result: 8
Method 3: Using the Repeated Subtraction Method
The repeated subtraction method is a simple way to perform long division. This method involves repeatedly subtracting the divisor from the dividend until the dividend is reduced to zero.
- Write the dividend and divisor as before.
- Subtract the divisor from the dividend repeatedly, keeping track of the number of subtractions.
- The number of subtractions is the quotient.
Example: 48 ÷ 6 =?
48 - 6 = 42 42 - 6 = 36 36 - 6 = 30 30 - 6 = 24 24 - 6 = 18 18 - 6 = 12 12 - 6 = 6 6 - 6 = 0
Result: 8
Method 4: Using the Mental Math Method
The mental math method is a quick way to perform long division. This method involves estimating the quotient and then adjusting as necessary.
- Estimate the quotient by dividing the first digit of the dividend by the divisor.
- Adjust the estimate by multiplying the divisor by the estimate and subtracting the product from the dividend.
- Repeat the process until the dividend is reduced to zero.
Example: 48 ÷ 6 =?
Estimated quotient: 8 8 × 6 = 48 48 - 48 = 0
Result: 8
Method 5: Using Technology
Technology can be a great tool for performing long division. There are many online calculators and math software programs that can perform long division quickly and accurately.
- Enter the dividend and divisor into the calculator or software program.
- Perform the long division operation.
- Check the result to ensure it is accurate.
Example: 48 ÷ 6 =?
Using a calculator, we get:
Result: 8
What is the best method for performing long division?
+The best method for performing long division is the one that works best for you. Some people prefer the standard long division algorithm, while others prefer the partial quotients method or the repeated subtraction method.
How can I check my result to ensure it is accurate?
+To check your result, multiply the quotient by the divisor and add the remainder (if any). This should equal the dividend.
Can I use technology to perform long division?
+Yes, you can use technology to perform long division. There are many online calculators and math software programs that can perform long division quickly and accurately.
In conclusion, mastering long division with no remainders requires practice and patience. By using one or more of the methods outlined above, you can become proficient in performing long division and solve problems with ease.